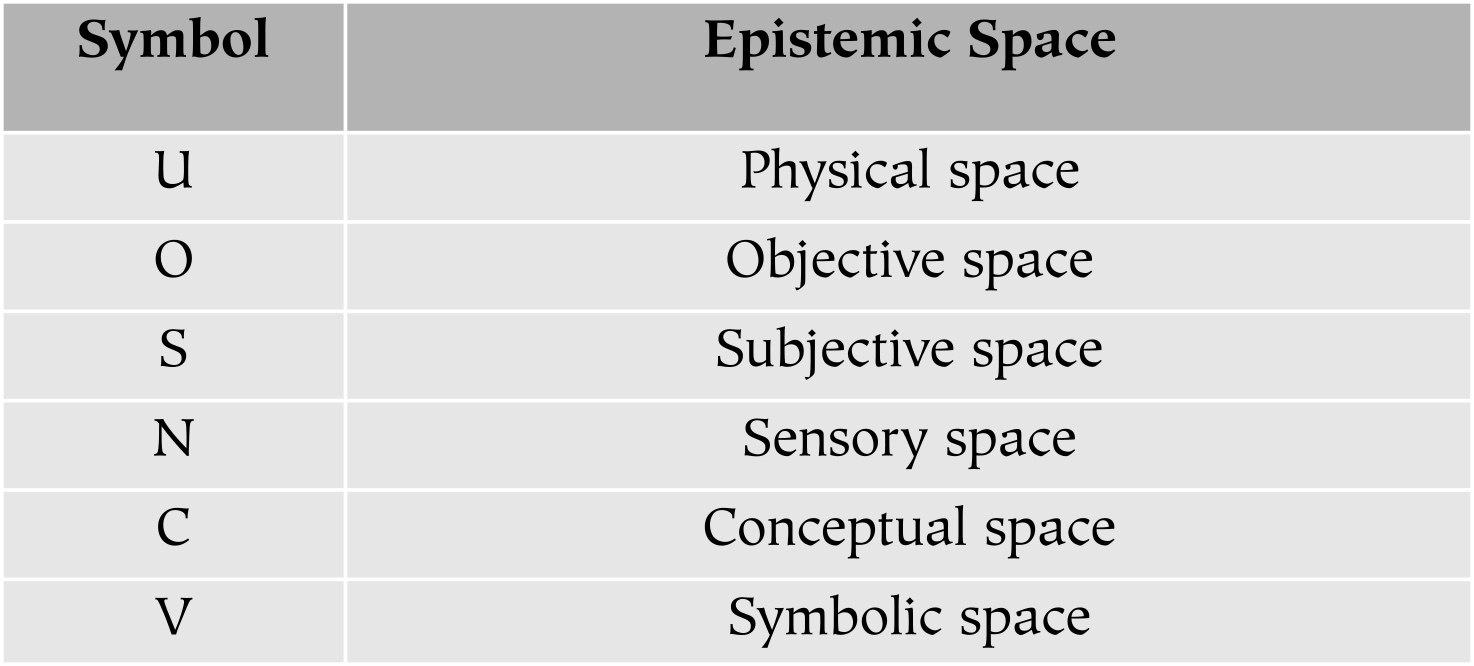
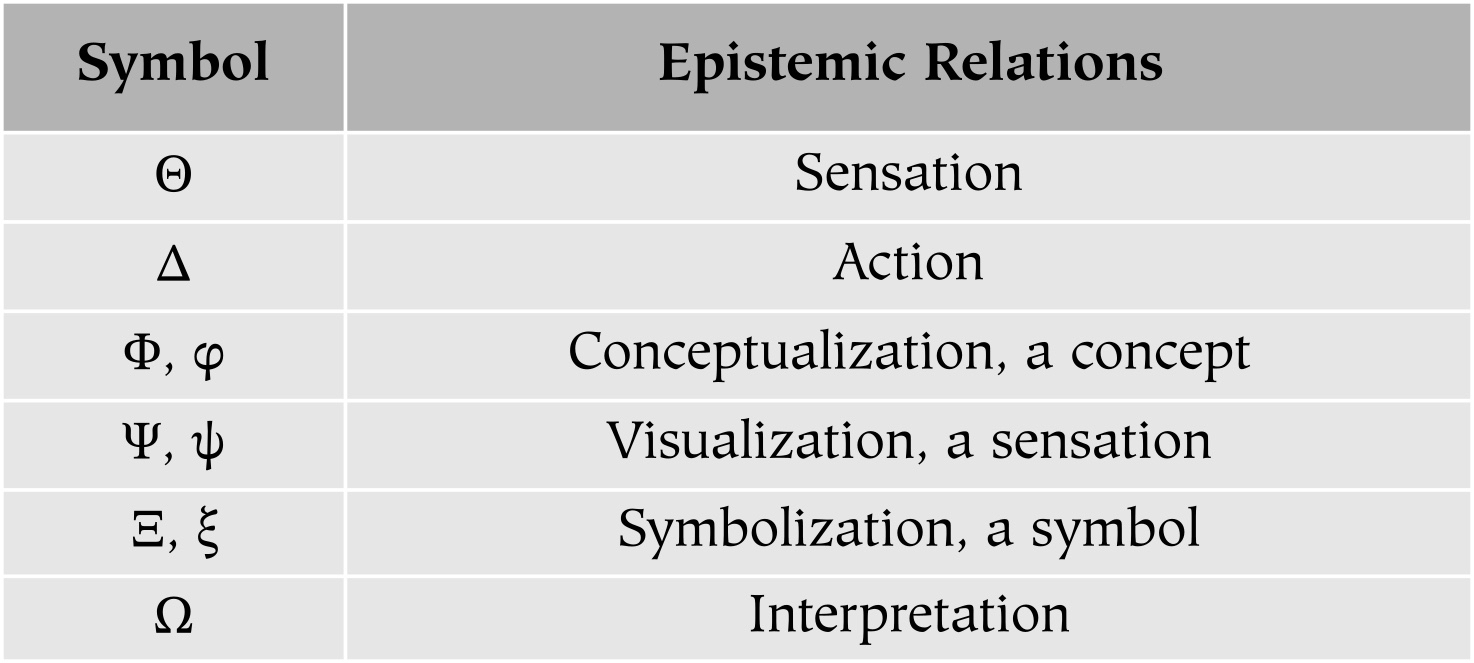
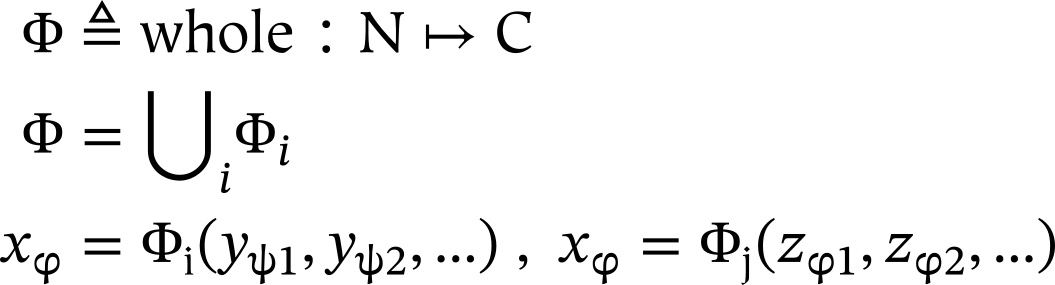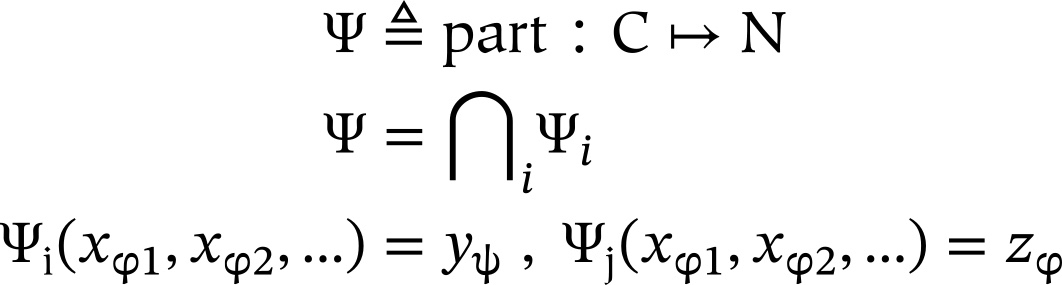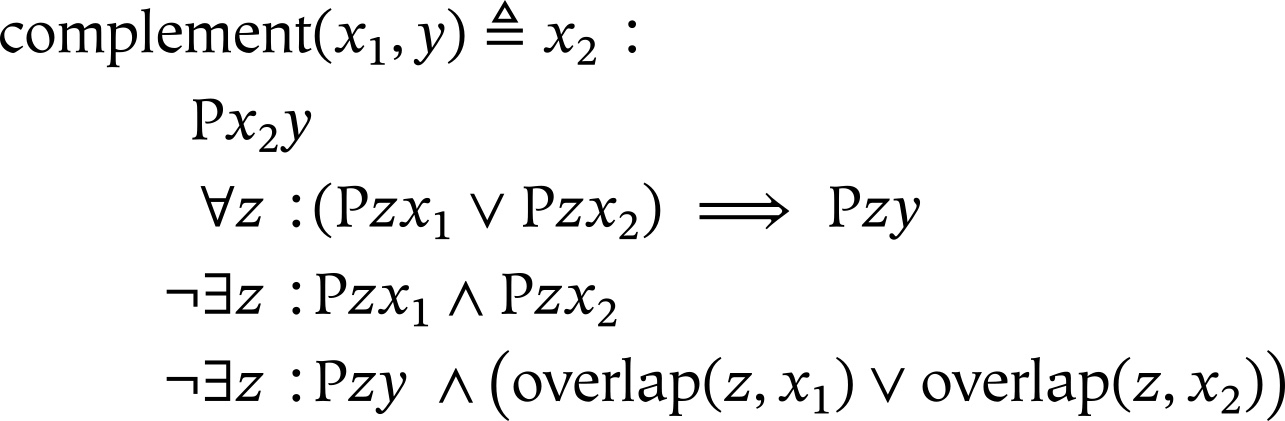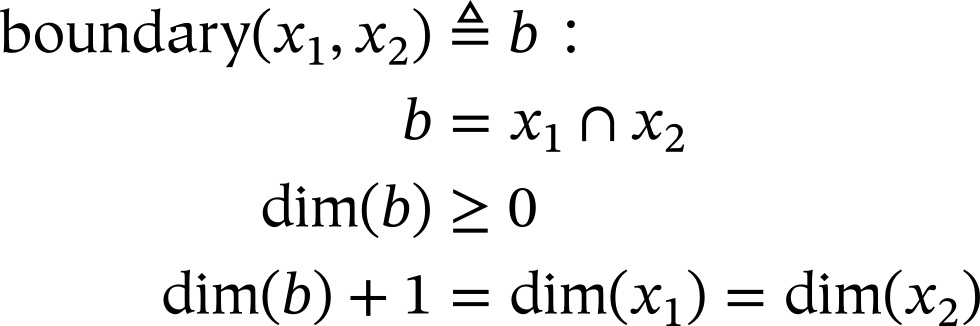Part 2
THEORY OF MEREOLOGY AND REFERENCE

What is it […] that divides the atmosphere from the water? It is necessary that there should be a common boundary which is neither air nor water but is without substance, because a body interposed between two bodies prevents their contact, and this does not happen in water with air. […] Therefore a surface is the common boundary of two bodies which are not continuous, and does not form part of either one or the other, for if the surface formed part of it, it would have divisible bulk, whereas, however, it is not divisible and nothingness divides these bodies the one from the other.
Leonardo da Vinci [Varzi, 2015].
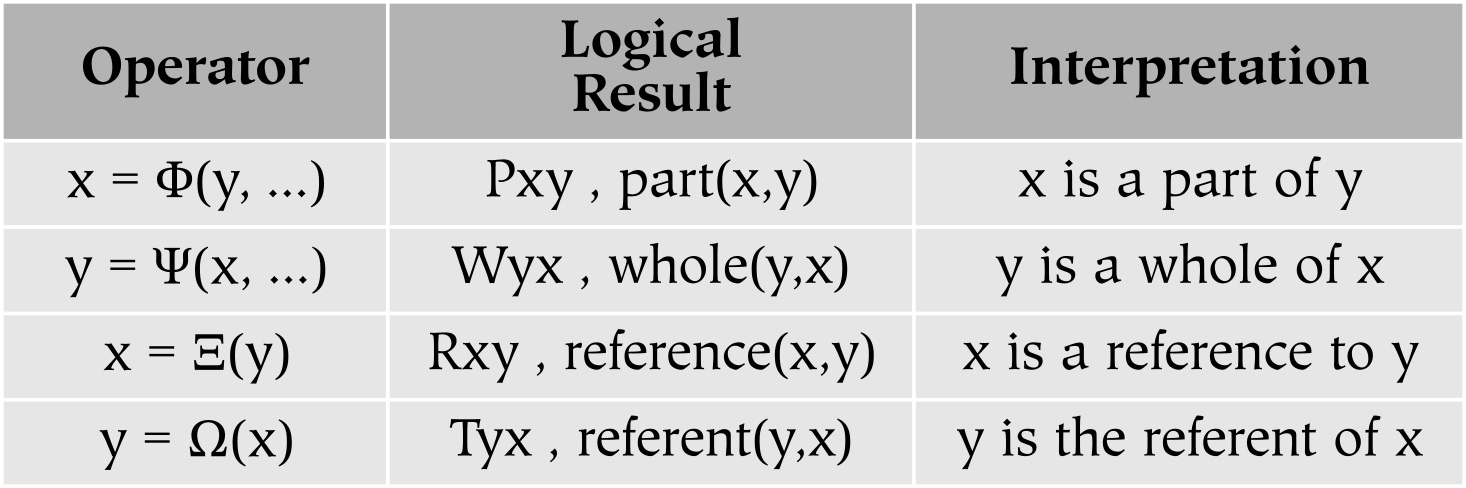
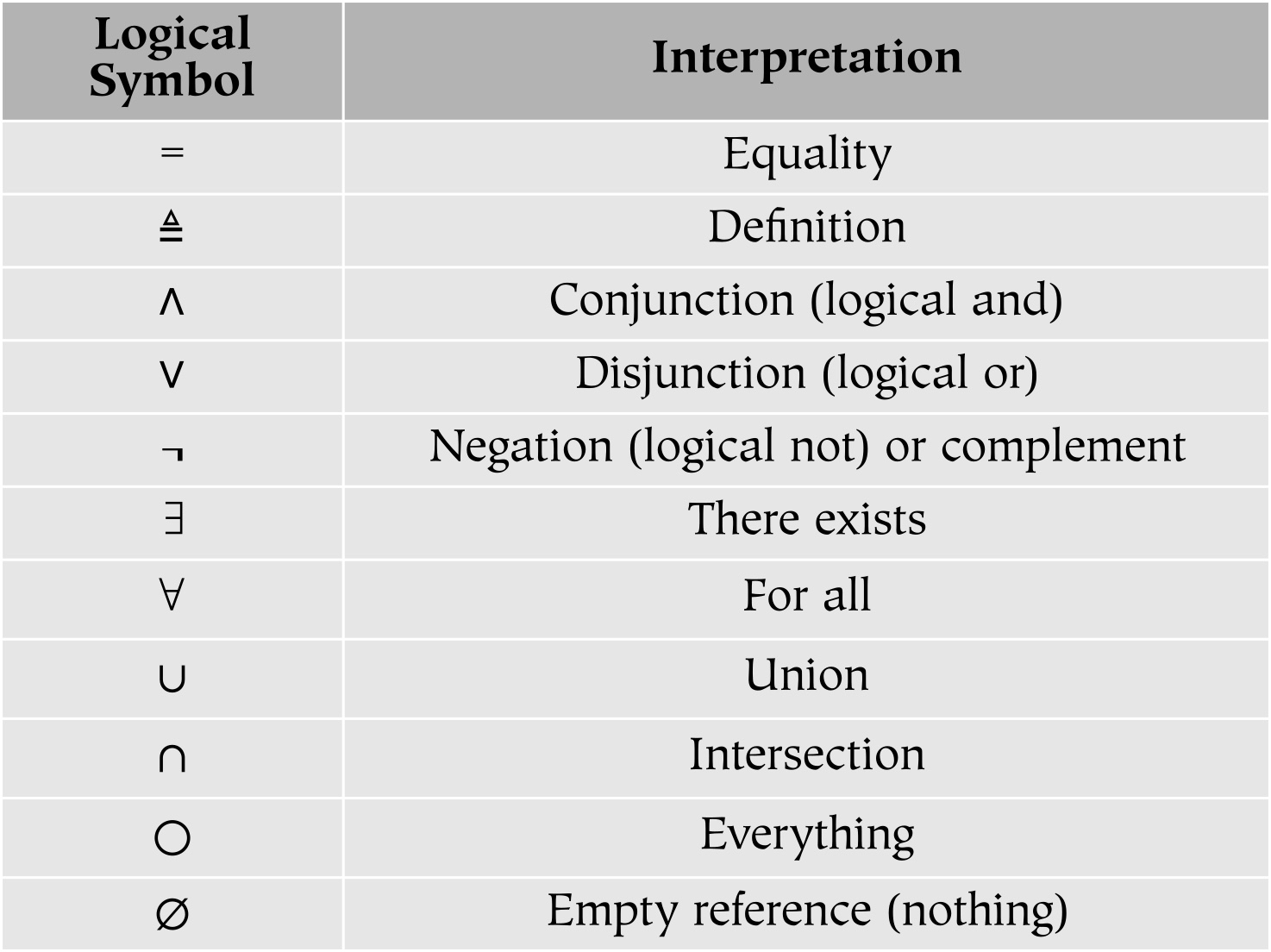
This book uses two types of analyses: mereological and referential. Mereological spaces are structured by the whole/part relationship, and referential spaces are structured by the reference/referent relationship. Mereological and referential analyses of a given space are nominalistic analyses that create individual things within that space. They are nominal in the sense that the structure of space is determined by the operation that is used to name or categorize it. As a result of these analyses, things can be divided into four general types: wholes, parts, references, and referents. However, most things may be any or all of these four types of things, depending on one’s point of view. For example, a word written on a piece of paper is a whole of many cellulose fibers, a part of a book, a reference to a concept, and the referent of this sentence.
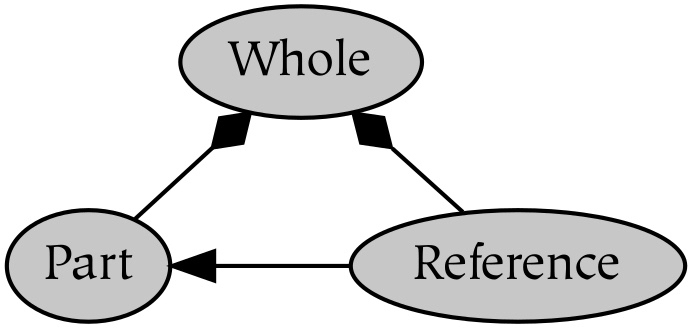
[Figure 2: A whole, a part, and a part that is a reference. ]
As an example, Figure 2 shows the way that references play a role in both mereological and referential spaces at the same time. To make this example more concrete, suppose that the diagram describes a room, a vase, and a piece of paper that has the word “vase” written on it (objects that are a whole, a part, and a reference, respectively). Both the vase and the paper are parts of the room, at least temporarily. The word on the paper (“vase”) is a reference to the vase object. Therefore, the word on the paper is both a thing in-and-of-itself and a reference to something else, as indicated in the diagram by the two attached arrows. In other words, it has a dual role: it has a mereological role (in relation to the paper, the ink, etc.) and a referential role (in relation to the vase object). Even the vase itself has a dual role, since it is both a part and a referent.
The multiple roles of the objects in this example create two different kinds of space: referential space (which is structured by references) and physical or mereological space (which is structured by wholes and parts). As the former space requires subjective interpretation, it may also be characterized as mental space. These differing types of spaces illustrate that the concept of space is used very generally in the context of this book as a metaphor to describe both physical and mental reality. Space should also be understood generally in that it is high-dimensional or open-dimensional. Understanding space in this way is particularly important because a central thesis of this work is that the dimensionality of space is shared with all things within that space. As a consequence, if the physical universe is four-dimensional, then our world contains four-dimensional objects as parts, and cannot contain three-dimensional objects except as abstractions.
As far as possible, space is left unanalyzed. Although analysis of physical space may determine things to be one, many, wholes, parts, references, or referents, space itself does not have a predetermined structure or fixed number of dimensions. In other words, physical space and the material within it are not inherently many things or one thing, so applying these mutually exclusive concepts to physical space would limit comprehension. This stance avoids conjectures about reality that are impossible to determine, and leaves open the possibility that space is inexpressible as it is experienced.
Chapter 3
Mereological Space

A mereological space is structured with wholes and parts. Mereological spaces are structured by the whole/part relationship. Both physical spaces and sensory spaces can be structured mereologically; for example, a tree is a part of a forest and the sensation of a tree is a part of the sensation of a forest. However, the relation between a referent and its reference is not mereological. In other words, symbols are not necessarily either parts or wholes of what they symbolize.
All parts of a mereological whole share the dimensionality of that whole. For example, there are no 2‑D surfaces in a 3‑D world, and there are no 3‑D objects in a 4‑D world; therefore, it is not correct to conceive of a 2‑D line existing in a 3‑D space from a mereological point of view (although it may be a useful approximation or an interesting epistemological limit). This means that abstract elements such as points, lines, and planes are incapable of constituting physical space. In other words, parts have a spatial extent along every dimension of the space that contains them, just as a piece of paper has more than two dimensions.
Further, for any two entities A and B in a mereological space, one of the following relations holds:
Neither is a part of the other (i.e., the entities are disjoint).
A is a part of B (i.e., B is a whole of A).
B is a part of A (i.e., A is a whole of B).
Both are parts of each other (i.e., the things are identical).
There is no definite parthood relationship between the entities (i.e., A is neither a part of B, nor is A not a part of B).
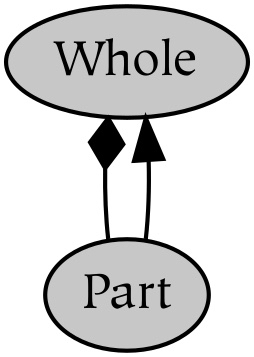
These relations are illustrated graphically in Figure 2.3a. The fifth case is somewhat problematic, because there is no simple answer to the question “Is A a part of B?”. To state that either “A is a part of B” or “A is not a part of B” would be incorrect: one part of A is a part of B and another part of A is not a part of B. It is tempting to say that “A is a part of B” AND “A is not a part of B”, but expressing that is beyond the capacity of ordinary (bivalent) logic.

[Figure 2.3a: The five possible mereological relations.]
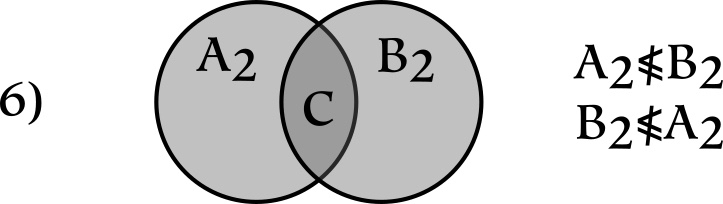
[Figure 2.3b: Recategorizing overlap
as three non-overlapping parts.]
In order to ensure that the part operation imposes at least a partial order on a given space, overlap must be prevented by categorizing any overlapping space as a new entity, as in Figure 2.3b. Epistemologically, however, it may make more sense to extend logic so that it accurately describes uncertainty, rather than removing all overlap (which may be impossible in some cases). To illustrate this, a continuous version of logic is introduced in appendix Formal Summary.
2.3.1 Wholes
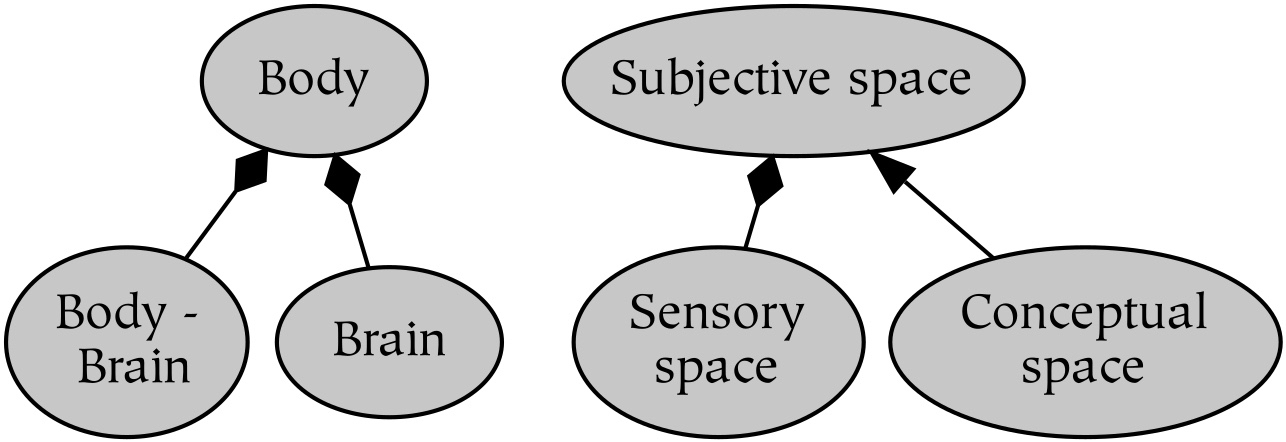
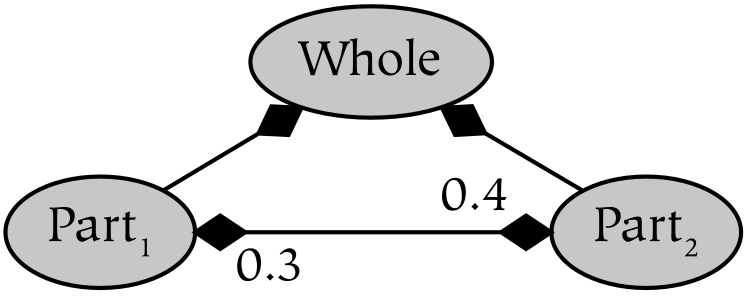
Wholes are composed of their parts. Figure 2.3.1 shows a whole that is composed of two parts. Although reading this figure from the top down indicates a whole being made of two parts, the orientation of the figure makes no functional difference since all structural relationships are indicated by the directionality of the arrows. Therefore, from a procedural point of view, a branch like the one depicted can be understood as either a division or a collection. As a division, it creates parts out of a larger whole, and as a collection, it creates a whole out of smaller parts.
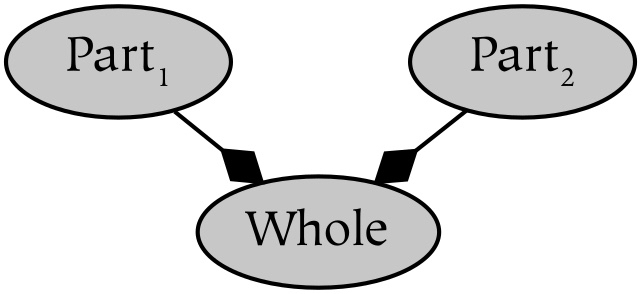
[Figure 2.3.1: A whole in relation to two parts.]
Because there is no mereological relation depicted between Part1 and Part2, one might assume that they do not overlap. In symbolic logic, however, this may only indicate that overlap cannot be expressed between discrete entities using the part/whole relation. Unfortunately, this inability to easily express overlap is problematic given the abundance of overlap in the world. For example, arbitrary parts of a tree such as the bark are partially coextensive with the trunk, so neither is entirely a part of the other.
As a result, any overlap which is not analytically removed is most often represented in separate hierarchies (i.e., mereological space within a single hierarchy most often has non-overlapping parts). Further, it is common for the parts within a single hierarchy to form a partition of that whole. Parts form a partition of their respective whole if the parts do not overlap and the space of that whole is completely covered by those parts. For example, apples and oranges do not form a partition of fruits, because there are some fruits that are neither apples nor oranges.
2.3.2 Parts
Parts compose their wholes. Figure 2.3.2 depicts exactly the same information as the figure in the previous section: a whole that is composed of two parts. In both cases, the figure illustrates that the same object can be nominally constituted by either one whole or two parts. Similarly, because the boundary is nominal, the presence or absence of a boundary does not materially affect the composition of the whole. In other words, although the universe may be mentally divided into things, the dividing lines do not have any concrete existence. This finding is non-trivial, since it implies that the boundary that does the dividing does not occupy the same space as the things it divides. For example, when points divide lines, 0‑D things partition a 1‑D space. The same principle applies when lines divide planes; in both cases, the extent of the partitioning element is zero along the dimension of the space that it divides.
Although this may not sound significant, it is an important departure from point-set topology, which is the field of mathematics that is typically used to describe space. Point-set topology assumes the existence of partless parts (or points) that constitute spaces of higher dimensionality. For example, a three-dimensional space is made up of an uncountable infinity of zero-dimensional parts. While this analysis works mathematically, it is problematic from a psychological point of view (for further details, see appendix Formal Summary).
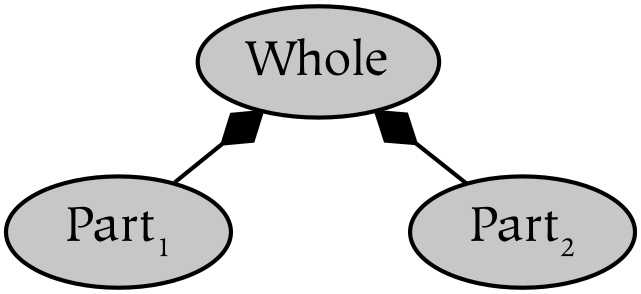
[Figure 2.3.2: Two parts in relation to a whole.]
These several principles of point-free-topology may be informally summarized as follows:
The dimensionality of a part is always equal to the dimensionality of its whole.
Boundaries such as dividing lines are nominal (i.e., they are not parts of the things that they divide).
Space is not a whole of partless particles and is not a part of a whole-less whole (i.e., it is not bounded below or above).
2.3.3 Mereological Identity
Mereological identity entails both
internal identity and external identity. Two things are mereologically identical if they have both the same wholes and parts.
If two objects have the same parts, they are internally identical. For example, the internal or material identity of a car may entail that it has four wheels, two axles, a body, a steering wheel, and an engine as parts. Internal identity is closely associated with essentialism, since the essence of a thing is generally considered to be internal to that thing.
If two objects have the same wholes, they are externally identical. External identity is often stated in terms of functional properties. For example:
A thing is a chair if you can sit on it.
However, all definitions using properties can also be stated using the language of wholes and parts. For example, the previous sentence can be restated as:
A thing is a chair if it is a part of the class of all things on which you can sit.
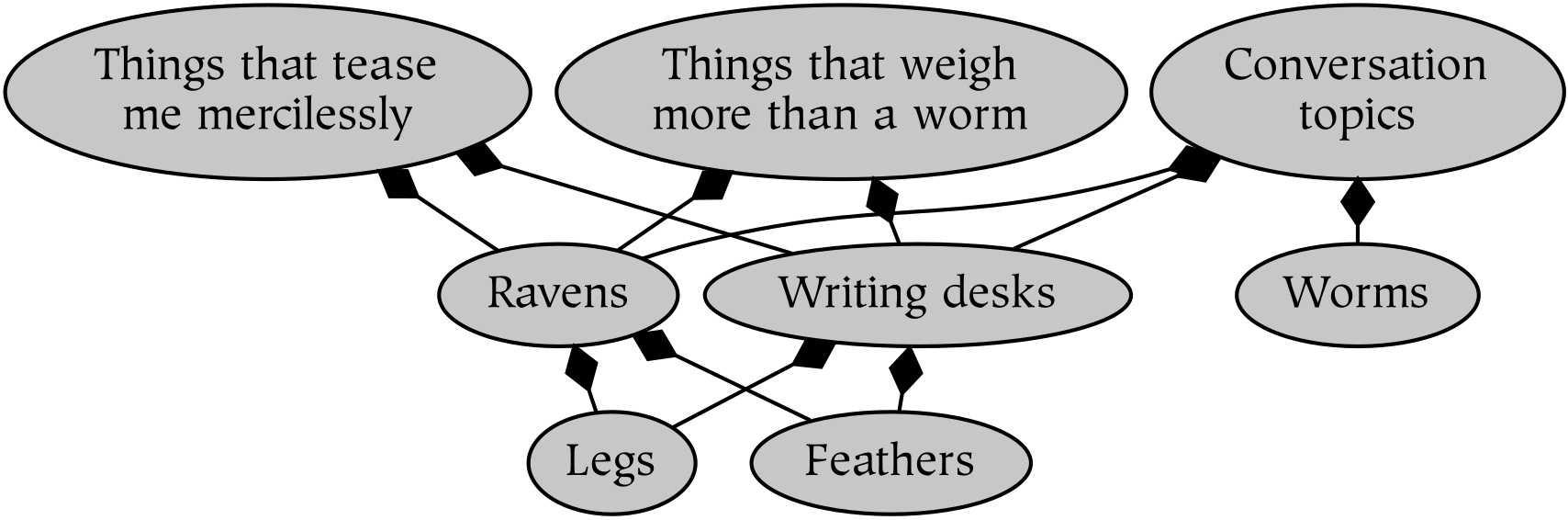
[Figure 2.3.3: The mereological identity
of ravens and writing desks.]
In order to illustrate the role of mereological context, Figure 2.3.3 depicts several of the wholes and parts of ravens, writing desks, and worms. In that mereological context, ravens, writing desks, and worms are parts of larger wholes, while ravens and writing desks are also wholes of smaller parts (worms are atoms because they have no parts). Because ravens and writing desks have the same wholes and parts in this limited context, they are both externally and internally identical, and therefore mereologically identical. In mathematical terminology, there is an isomorphism between ravens and writing desks.
2.3.4 Mereological Edge Cases
There are two mereological edge cases:
wholeless wholes and partless parts. The edge cases of the whole/part continuum are whole-less wholes and part-less parts, or ultimate wholes and ultimate parts. Ultimate objects are particularly interesting because things with no wholes lack external properties, and things with no parts lack internal properties. This makes them paradoxical to talk about or even think about, although they figure into our mental lives frequently.
In mathematical mereology, a space with upper or lower limits on the operation of parthood is called bounded above or bounded below, respectively. If there are no such bounds, that space is called open (i.e., open above or open below).
2.3.4.1 Ultimate Wholes
A mereological thing that has no wholes is
an ultimate whole, or a universe. The ultimate whole is the biggest thing. Only one ultimate whole can exist within a single mereological space, since if two ultimate wholes exist in the same space, then there would be a larger (conceptual) whole that consists of both. In an epistemological context, the ultimate whole is similar to the mathematical notion of the set of all sets. In an ontological context, the ultimate whole is known as the universe.
The term “universe” derives from the Latin universum, meaning “[every object] combined into one”. Unfortunately, this term may also designate an object that is not the largest thing (i.e., if one believes in things that extend beyond the universe, such as multiverses). In this work, the word “universe” is used as originally intended; the universe is that thing which is a whole of everything else. As with space, the universe is open-dimensional. Therefore, although the universe is typically described with spatial (i.e., atemporal) metaphors, it is better understood as a long-lasting event because it has both spatial and temporal extents.

[Figure 2.3.4a: Universes are wholeless wholes.]
Because there is nothing to which the universe can be compared other than itself or parts of itself, it is difficult to define. One could call it large in relation to its parts, but that would entail that all things are large things. As the universe cannot have external properties, it cannot be big or small, heavy or light. In other words, since definitions are always given in terms of other things (i.e., they are relative), it is not possible to define “the one without a second”. For example, to call the universe a unity implies that there is some plurality with which it can be contrasted, which is not the case. However, while it may be mistaken to make a relative statement about the universe, it may help to counter prior misconceptions. For example, the assertion that the universe is one entity might be correct in so far as it counters the assertion that the universe is a multiplicity of independent things.
Conceptually, the universe is a space without limits; it is not finite or closed, which is required by wholes that have no further wholes. Closed space is paradoxical, since one could imagine going to the end of the universe and then moving a bit beyond that location. If that is possible, then the universe is not closed. If that is not possible, it suggests that the obstruction is a thing that lies beyond the boundary, which also entails that the world is not closed. In other words, if boundaries exist only as the division between parts, then wholes which are not parts in any larger whole do not have boundaries. On that account, universes cannot have a boundary because there is nothing for them to be divided from, and therefore they are unbounded by definition.
2.3.4.2 Ultimate Parts
A mereological thing that has no parts
is an ultimate part, or an atom. An ultimate part is the smallest thing, and is called an atom. The term “atom” derives from the Greek atomos, which means uncuttable or indivisible. For space to be atomic, or to have parts which are atoms, means that the process of creating parts cannot occur indefinitely. In other words, they are things that cannot be subdivided.

[Figure 2.3.4b: Atoms are partless parts.]
Atoms have no parts in virtue of which they can be distinguished from one another. Therefore, all atoms are internally identical, and can only be distinguished from one another in virtue of their larger wholes. As a result, the atom represents the limit of reductionism as an explanatory strategy; it is not possible for reductionism to explain what an atom is in terms of its parts, because it has no parts.
Although both atoms and points are partless and therefore indivisible, atoms are not equivalent to points in the mathematical sense. Atoms have a spatial extent, whereas points have no extent; therefore, atoms exist in discrete space, while points exist in continuous space. More information about the difference between points and atoms is presented in appendix Formal Summary.
Chapter 4
Referential Space

A referential space is structured with
references and referents. Referential spaces are structured by the reference/referent relationship. The things that are named are called referents, and the things that name them are called references. References are directional, in that references point to their referents.
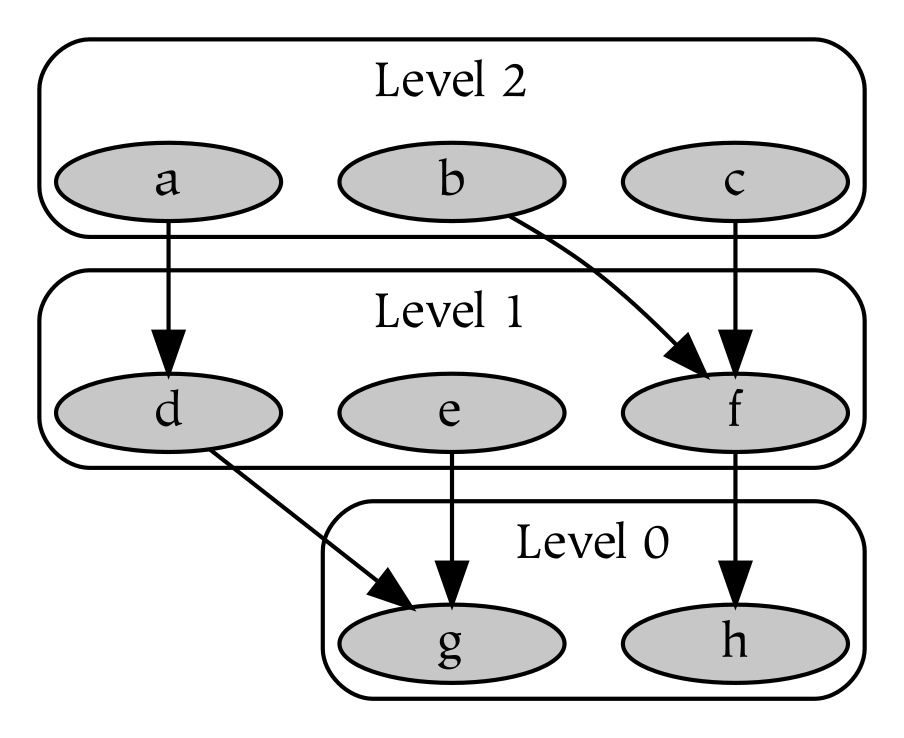
[Figure 2.4: References stratify things
according to their referential level.]
References are not mereological because the relationship between a reference and its referent has nothing to do with whether they are parts of one another, although references and the referents on which they depend often form parts of a mereological space. References are discrete, although the referential relation may in general be continuous (in which case it is called reflection).
Referential space itself is typically divided into subspaces of referenced objects and referencing objects. Because references can in turn be referents, a stratification of referential level is established. As Figure 2.4 illustrates, references form referential chains (depicted vertically) that may pass through multiple referential levels (depicted horizontally), mapping onto referents in lower levels. Things on the bottom level of the diagram have a referential level of zero and are not references to anything else (they are “things in themselves”). The referential level of each successive level is one more than the level to which it refers. Therefore, referential level indicates the distance of a reference from ground, or its ultimate object of reference.
2.4.1 References
References refer to referents. The word reference derives from the Latin referent, which means “bringing back”. References are things that are capable of referencing or denoting a referent, so the existence of a reference implies that its referent can be found in the world. There may be multiple references for a single referent, but a reference can only refer to a single referent, a relation depicted in Figure 2.4.1.
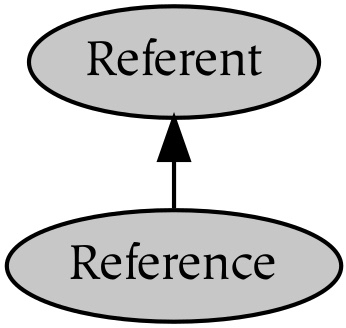
[Figure 2.4.1: A reference in relation to its referent.]
References are both things themselves as well as things that refer to other things. They do not need to resemble their referents in any way, a principle known in linguistics as the arbitrariness of signs. Therefore, the significance of references as references does not derive from what they are, but from what they represent or signify. They play a crucial role in cognitive psychology, where the referenced objects of the external world become referential parts of the space of subjective awareness.
2.4.2 Referents
Referents are denoted by references.
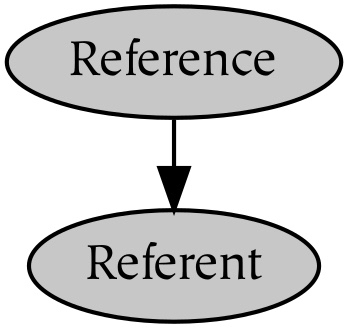
[Figure 2.4.2: A referent in relation to its reference.]
Referents are the things which references point to, reference, denote, or name. Referents may be denoted by multiple references, and they may in turn be references to other referents, but they do not have a mereological structure. Thus, although referents may have dimensionality in virtue of being parts of mereological spaces, their only structure within referential space is derived from their referential relationships. In other words, references and referents are atoms within discrete referential spaces, and consequently, there is no such thing as a half of a reference.
2.4.3 Referential Identity
Referential identity entails that two references
refer to the same referent. The notion of identity can be applied to references in at least two ways. Referential identity means that two things are identical if they have the same referent. For example, the references “the first man to walk on the moon” and “Neil Armstrong” have the same referent, so these two statements are referentially identical. References also establish an identity between a reference and its referent.
Since references can represent something unlike themselves, the validity of the reference/referent mapping is established via isomorphism. For example, isomorphism between a reference and its referent, such as the concept riverφ and a physical river, entails that the concept relates to other concepts just as the river relates to other physical objects. This relational basis for identity allows a reference to be independent of its referent; for example, the concept of the river may be contextually similar to the physical river without needing to have identical physical characteristics, such as being physically wet.
2.4.4 Referential Edge Cases
There are several referential edge cases: ultimate referents, ultimate references, self‑referential references,
empty references, and full references. There are two primary edge cases in referential spaces: ultimate references and ultimate referents. Ultimate references cannot be referenced, and ultimate referents are non-referential. Unlike parts, which require a relation between exactly two objects, references have two additional edge cases that involve only one object: references that refer to themselves (or self-references) and references that refer to nothing (or empty references). This section also introduces full references, which are the complement of empty references.
2.4.4.1 Ultimate Referents
An ultimate referent does not reference any other referent.

[Figure 2.4.4a: An ultimate referent is not a reference
to any other referent.]
In some philosophical theories such as Kant’s, ultimate referents are things-in-themselves (dinge an sich) that form a referential ground of being; without them, references become vacuous. In other words, ultimate referents are particularly significant because they create a grounding that gives all other references meaning. If this grounding is not present, then all references become meaningless. However, what are ultimate referents from one point of view may not be ultimate referents from another, so while it may be necessary to begin with a referential ground, that referential ground is not necessarily unique.
A profound example of references with no ultimate referents is a dictionary, since all of the words in a dictionary are defined by other words. Therefore, their definitions consist of references that are eventually circular. For example, if an alien who didn’t know any words tried to find the definition of a word in a dictionary, the definition of that word would necessarily contain other words that the alien does not know, which would entail looking up further words. This situation involves an infinite regress; if the alien does not know the meaning of any words to begin with, how is it possible to learn even one of them? The only way to break this endless cycle is to know the meaning (experientially) of one or more of the words, and in this process, those words become ultimate referents.
2.4.4.2 Ultimate References
Ultimate references cannot be referenced.

[Figure 2.4.4b: An ultimate reference is not a referent of any other reference.]
NB: It is not possible to talk about ultimate references, because to do so would be to refer to them.
2.4.4.3 Self‑Referential References
Self‑Referential references refer to themselves. Self-referential references seem possible, unlike the obviously impossible example of a (proper) part that contains its whole. However, self-reference is problematic and the basis for innumerable paradoxes.

[Figure 2.4.4c: A self-reference refers to itself.]
A common example of self-reference is the Liar’s Paradox, attributed to Parmenides (ca. 485 BCE):
I am lying.
Although this statement seems rather innocuous at first, its truth or falsity is difficult to assess. If it is assumed to be true, then it becomes false. At the point of being negated, it becomes true once again. Establishing the ultimate validity of this sentence is therefore impossible, as it involves an infinite regress.
Probably the most significant thing about Parmenides’ statement is that it is self-referential: it describes itself. Hence, a reasonable first step in the elimination of paradoxes eliminates self-reference. Unfortunately, the recognition of self-reference is confounded because reference does not have to be immediate; it can be a multi-step, circular phenomena, as illustrated by the two statements below:
Statement (2) is true.
Statement (1) is false.
The paradox in this case is more difficult to spot, but it is an example of self-reference when both statements are considered as a single whole that refers to itself. Specifically, the first statement is neither true nor false until it is evaluated. If one assumes that it is true, the truth of the second statement is asserted. The second statement asserts that the first statement is false, which contradicts the original assumption, and so on, such that the truth conditions never converge.
2.4.4.4 Empty References
Empty references refer to nothing.

[Figure 2.4.4d: An empty reference does not refer to anything.]
Although almost all referential relations involve a referent, there is one important exception: the empty reference, which is a reference whose referent does not exist or which refers to nothing. Since it has no referent, it is not entirely clear that it is a reference, although it is widely used in practice.
2.4.4.5 Full References
Full references refer to everything.
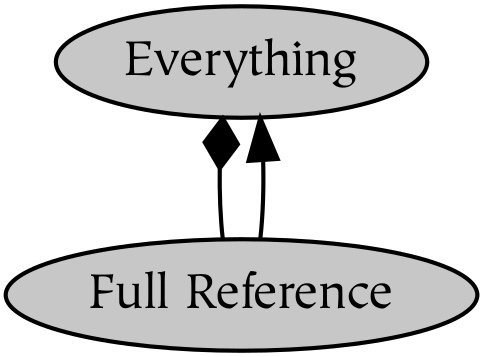
[Figure 2.4.4e: A full reference refers to everything.]
Another unusual type of reference that bears mention is the full reference, which refers to everything, or to all things at the referential level to which it refers. It is particularly significant because it forms a trivial isomorphism between two referential levels (i.e., because it refers to all referents at a given referential level, it does not require any discrimination to be learned between those referents).
The full reference is defined constructively, or in virtue of things that exist at a lower referential level. As an important side effect, a full reference never refers to itself. This definition avoids the paradoxes associated with self-reference by using a (constructivist) solution identical to that proposed for similar conundrums related to the set of all sets.
Chapter 5
Combining Spaces

The combination of mereological and referential spaces yields spaces unlike either one. Most of this book uses hierarchies to structure spaces because of their relative simplicity. Hierarchies are a structure whose nodes are described using the metaphor of a family tree: parents are depicted above children and siblings are depicted next to one another. Hierarchy can also be described using the tree metaphor, although the trees are typically depicted as branching downwards instead of upwards, such that parent and child nodes are called “root” and “branch” nodes.
Different types of hierarchies use different structuring elements as relations. This chapter examines the two different types of hierarchies that correspond most closely to mereological and referential spaces: meronomies and taxonomies.
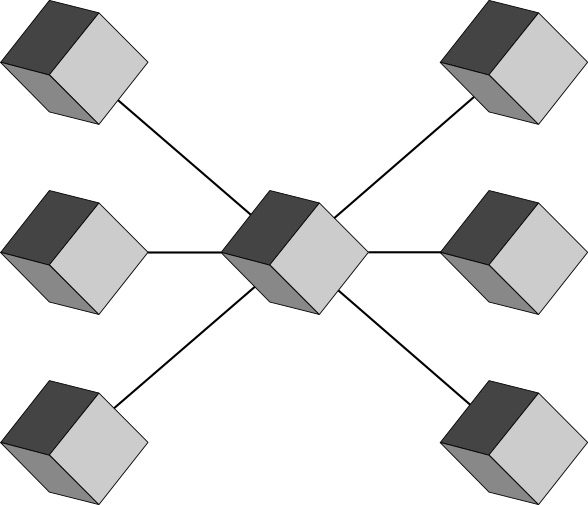
2.5.1 Meronomies
Meronomies are hierarchies that are structured
with the composition relation. Meronomies are mereological hierarchies in which the children are parts of the parent. Meronomies simultaneously represent divisions of a larger whole and collections of parts, so if the trunk is-a-part-of the tree then the tree is-a-whole-of the trunk, and vice-versa. Figure 2.5.1a depicts the meronomy of a tree, whose parts are roots, trunk, branches, and leaves. It depicts those parts using the composition relation, which is often called the has-a relation, as in “a tree has-a trunk”.
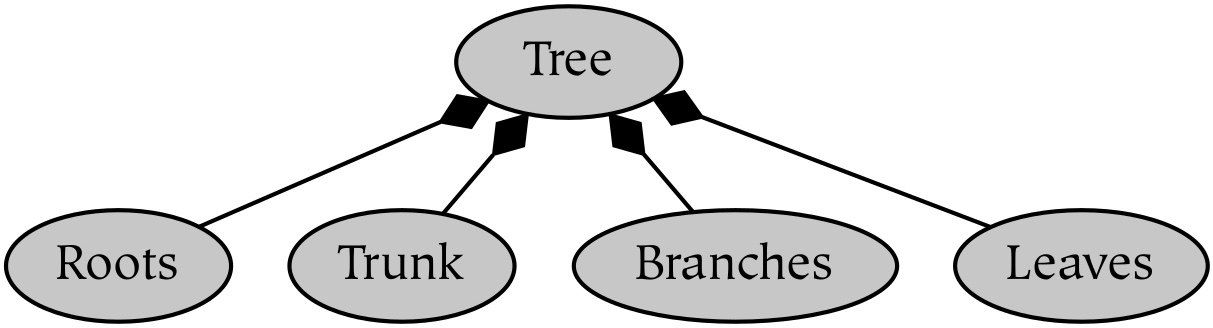
[Figure 2.5.1a: A meronomy is a hierarchy that uses
the composition relation.]
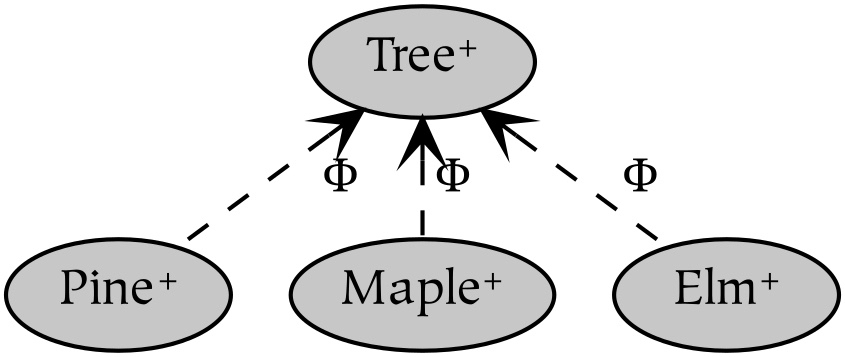
It is not possible to create meronomies with abstract child nodes; the rationale behind this is based on their cognitive structure, and is discussed in section Cognitive Taxonomies. Therefore, even though it is true that a pine is a tree, a maple is a tree, and an elm is a tree, meronomies like those shown in Figure 2.5.1b are invalid (where the superscript “+” denotes that the entity is abstract).
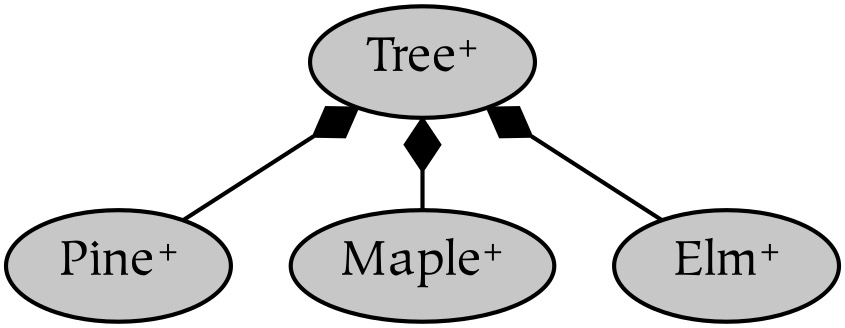
[Figure 2.5.1b: A meronomy is invalid
if its child nodes are abstract.]
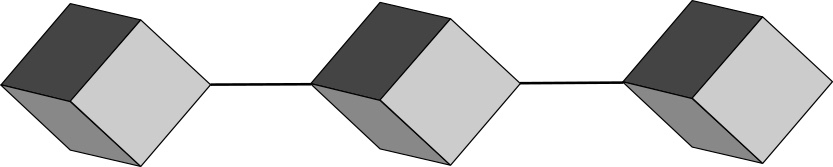
It is possible to create meronomies that have discontiguous concrete nodes, although they are somewhat uncommon (perhaps because it is easier to form concrete concepts of contiguous objects than of discontiguous objects). As an example, Figure 2.5.1c illustrates a whole (“All trees”) that is composed of parts that are concrete collections of different trees (“All pines”, “All maples”, and “All elms”).
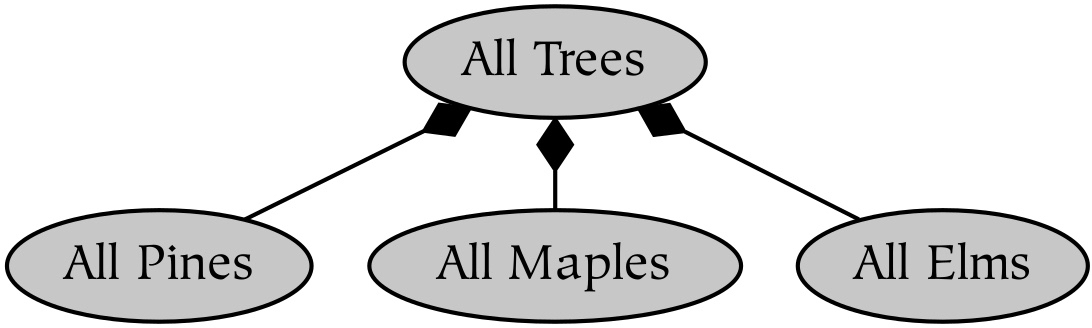
[Figure 2.5.1c: A discontiguous meronomy.]
2.5.3 Dimensions
Dimensions are axes along which
a thing can be differentiated. Dimensions are the measurable extents of things in space. The number of dimensions of an object are the number that are necessary to specify a unique location within that space. Although physical dimensions are continuous, dimensions may in general correspond to several types of scales: ordinal, nominal, and interval (ratio scales are omitted for brevity). These types of scales correspond to sorted, unsorted, and measurable dimensions, as illustrated in the following examples:
The order in which children arrive at the dinner table imposes an ordinal (sorted) dimension over those children.
The names of children create a nominal (unsorted) dimension over those children.
The ages of children impose an interval (measurable) dimension over those children.
2.5.3.1 Ordinal Dimensions
Ordinal dimensions are sorted by a structural element such as the composition relation. An ordinal dimension imposes a total or partial order on the elements that it structures. For example, finishing first, second, or third in a marathon are relative positions that constitute an ordinal dimension. Knowing the position does not tell you exactly what the finishing time was, but it does convey that one finishing time is greater or lesser than another.

[Figure 2.5.3a: Ordinal dimensions sort their elements.]
Two different ordinal relationships are shown in Figure 2.5.3a. Because parts must be smaller than wholes, an order is established between the nodes labeled “tree”, “branch”, and “leaf”. Ordinal relations are transitive: if a leaf is a part of a branch, and a branch is a part of a tree, then a leaf is a part of a tree. Similarly, if a Labrador is a dog, and a dog is an animal, then a Labrador is an animal. As the diagram illustrates, an ordinal dimension imposes an order which is not present among siblings (e.g., there is no order between different types of animals such as dogs and cats).
2.5.3.2 Nominal Dimensions
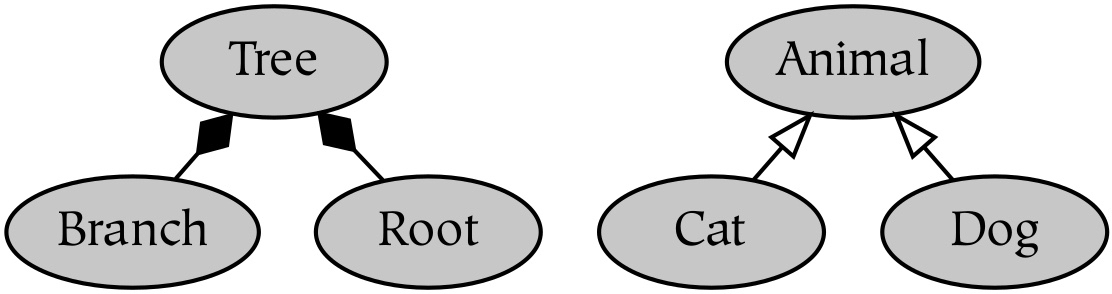
Nominal dimensions are constituted by unordered entities, which are distinguished only by name.
[Figure 2.5.3b: Nominal dimensions name their elements.]
A nominal dimension is unordered, since it has no basis to determine the relative positions of things. Figure 2.5.3b depicts two such dimensions, parts of trees and types of animals, both of which are nominal because the position of the child nodes is not significant (and therefore the children must be distinguished by name).
For taxonomies, nominal dimensions create nominal identity. Nominal identity works by creating a parent type that contains all of the children as subtypes or tokens, which become identical with respect to being tokens of that type (in this way, the children form an equivalence class). For example, cats and dogs are the same to the extent that they are both animals. Nominal identity is explored further in section Wholes of References.
2.5.3.3 Interval Dimensions
Interval dimensions are formed by combining
nominal and ordinal dimensions.

[Figure 2.5.3c: Interval dimensions combine ordinal and nominal dimensions.]
An interval dimension introduces an additional relation between its parts that results in a measurable distance (or metric) between those parts. A numerical example is shown in Figure 2.5.3c, where 1 is the same distance from 2 as 2 is from 3 and as 3 is from 4, precisely because the distance from one to the next is the same number (1). Since the same node cannot appear more than once in a meronomy, the meronomy on the left depicts the repeated unit element (1) as different things (1n). These different things form an equivalence class in the taxonomy on the right, however, which is what makes the intervals identical (i.e., all “1”).
2.5.4 Orthogonality
Two dimensions are orthogonal if a change in one dimension does not necessitate a change in the other. When working with multiple dimensions, it is useful to characterize them with respect to one another as being either dependent or independent. If dimensions are independent of one another, they are called orthogonal. For example:
The colors and sizes of an object form orthogonal dimensions, since you can change the color of an object without changing its size (and vice-versa).
The size and weight of a thing are not orthogonal, since the size of an object is correlated to its weight (assuming constant density).
Euclidean dimensions are orthogonal to each other, because you can change the position of an object on the x-axis without changing its position on the y-axis.
Diagrammatically, orthogonality determines how hierarchies can be combined with one another. For example, assume that one wishes to combine the two taxonomies shown in Figure 2.5.4a, which divide a common type (x) into subtypes a, b, c, and d. These hierarchies can be combined in two primary ways: either by grafting parent nodes together or by appending the branches of one hierarchy to each of the terminal nodes of the other. The criteria for choosing one method as opposed to the other is both to prevent overlap of the nodes and to ensure that the space is fully partitioned.
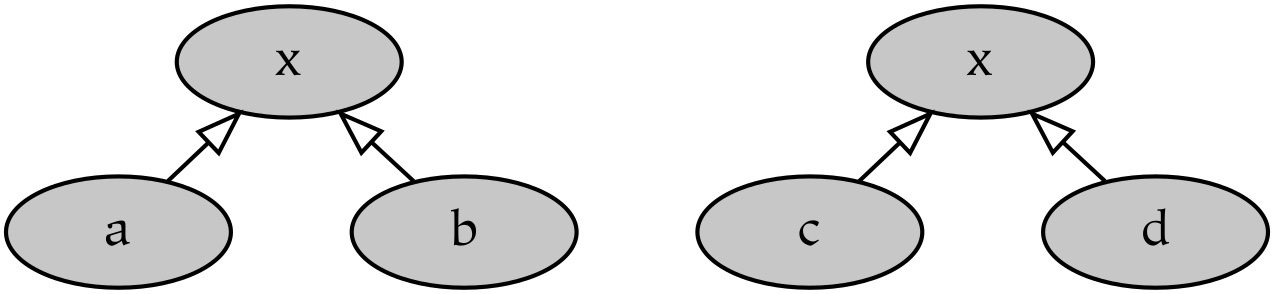
[Figure 2.5.4a: Two hierarchies representing different divisions of the same whole.]
The first possibility for combining these two taxonomies is to create a single tree with a parent (x) and four child nodes (a, b, c, and d). For example, if the a/b and c/d distinctions both correspond to color, and no object can have multiple colors, then the two dependent hierarchies are combined, as in Figure 2.5.4b. When the a/b and c/d dimensions are orthogonal, however, this type of combination is not appropriate. For example, if the a/b dimension represents “young animals”/“old animals”, and c/d represents “stupid animals”/“smart animals”, then combining these dimensions in a flat hierarchy would not create a structure capable of categorizing animals that are both smart and young.
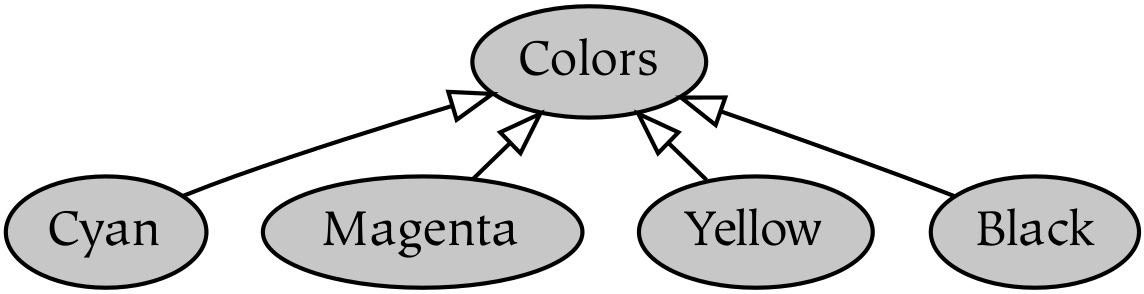
[Figure 2.5.4b: A combined (1‑D) hierarchy.]
Therefore, combining hierarchies that are orthogonal requires appending the branches of one tree to each of the terminal nodes of the other tree, as shown in Figure 2.5.4c. This kind of combination increases the depth of the hierarchy, and that additional depth allows additional information to be encoded. Each of the four different choices corresponds to a path from the root of the tree to a terminal node, or a selection from each of the two constituent categorizations, young/old and smart/stupid. As a result, the previous dilemma of how to categorize smart young animals is resolved (and corresponds to the rightmost path in the tree).

[Figure 2.5.4c: A combined (2‑D) hierarchy.]
2.5.5 Wholes of References
Wholes of references are wholes of parts that are also references in a referential space. In mereological space, things can be both wholes and parts. In referential space, things can be both references and referents. The combination of mereological and referential spaces results in further subtypes:
Wholes that are references
Wholes that are referents
Parts that are references
Parts that are referents
The arbitrary combination of wholes, parts, referents, and references in the context of cognition is not always useful, however, since many of these combinations do not exist. For example, it is not possible to form parts of references, so that combination is not explored further. It is possible, however, to form wholes-of-parts-that-are-references (or wholes of references), and that construct is widely used.
A whole of references exists within mereological space; however, each of the parts of that whole (i.e., the references) also exist within referential space. Wholes of references are unlike wholes of parts for the following reasons:
Wholes combine their parts in a single space.
The dimensionality of continuous parts is equivalent to the dimensionality of their wholes.
References are discrete (i.e., they are atoms).
Combining discrete entities into a single space creates a discrete space whose dimensionality is one higher than its constituent referential atoms (i.e., since combining discrete entities in a single space is not possible without doing so).
In a cognitive context, wholes of references are called symbols, which are explored further in section Symbolic Space.
2.5.6 Beyond Hierarchy
Hierarchies are a pragmatic but limited structure
for comprehending reality. Although hierarchies are useful and relatively easy to grasp conceptually, there are several problems associated with the reification of hierarchies:
Hierarchies have only one root, which reinforces the notion that all parts have a single whole.
Hierarchies do not have overlapping nodes; therefore, they implicitly reinforce the notion that there is a single correct way to partition reality.
Because a single hierarchy categorizes things in only one way, objects are often identified exclusively as parts, wholes, references, referents, or some combination thereof.
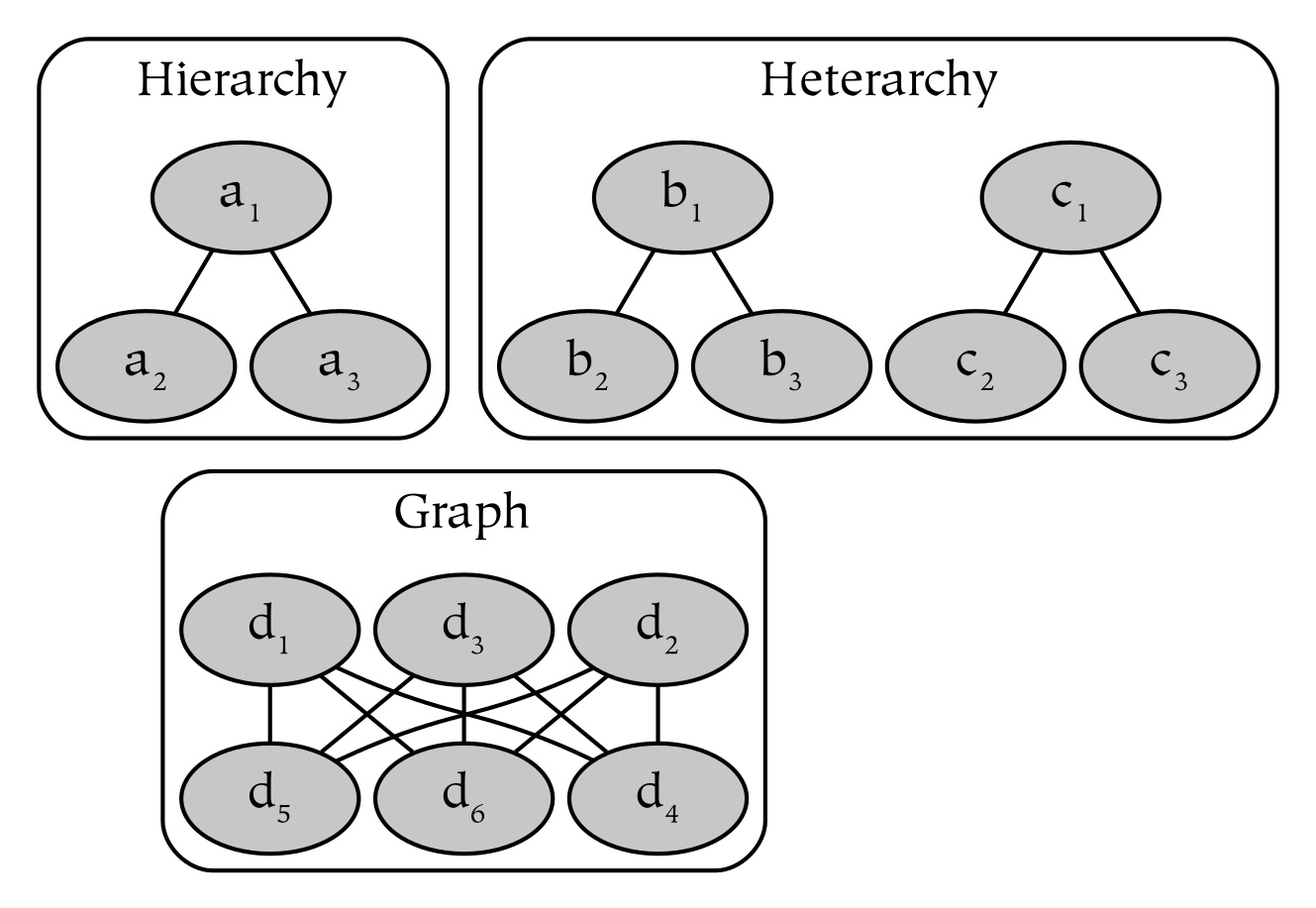
[Figure 2.5.6: Three different ways to organize nodes.]
Two alternatives to hierarchies are presented in Figure 2.5.6: heterarchies and graphs. Although these alternatives are comparatively less simplistic, neither of them addresses the complexity of multiple overlapping layers and nodes. To partially address this lack, bidirectional parthood (overlap) and bidirectional reference are introduced in appendix Ideographic Conventions.
Part 3
THE BASIC MODEL

Applying the theory of mereology and reference to human experience creates physical, subjective, and conceptual spaces, as well as several relations between those spaces.
The basic model of cognition begins with the universe, or physical space, and defines two further subspaces from the perspective of an individual. Everything within physical space that is experienced by that individual constitutes subjective space, and everything within subjective space that is conceptualized by that individual constitutes conceptual space. In summary:
1. Physical space (U) is the physical universe, or reality. Its parts are called events.
2. Subjective space (S) is everything in physical space that is experienced by a given individual. Its parts are called experiences.
3. Conceptual space (C) is everything in subjective space that is conceptualized by that individual. Its parts are called concepts.
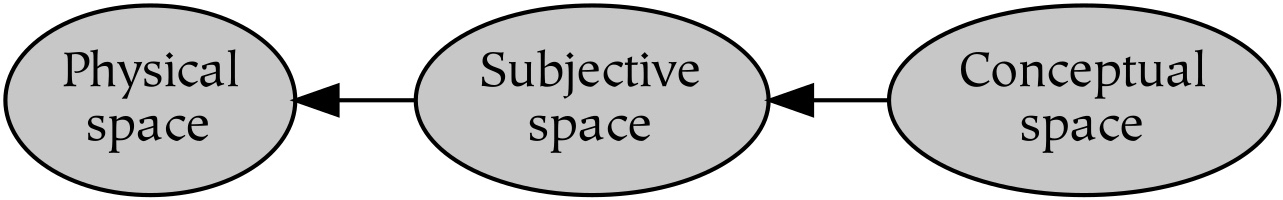
[Figure 3a: Three referential spaces.]
These spaces are distinguished in terms of reference: subjective space contains references to physical space, and conceptual space contains references to subjective space. Because the notion of reference in a psychological context corresponds to knowing, they are called epistemic spaces. For example, for a concept to reference an object is for it to know about (or represent) that object. Therefore, minds are collections of references, in addition to whatever else they may be.
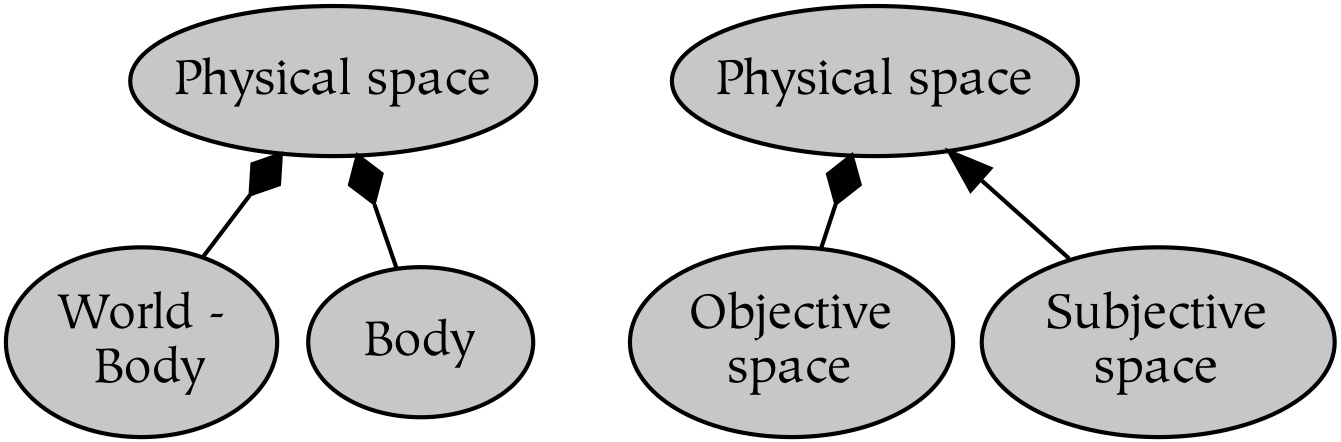
These epistemic spaces can be depicted in virtue of their referential or reflective relationship to one another, as in Figure 3a. These spaces are also parts of one another: subjective space is a part of physical space and conceptual space is a part of subjective space. This is graphically depicted in Figures 3b and 3c, both of which express the same thing.
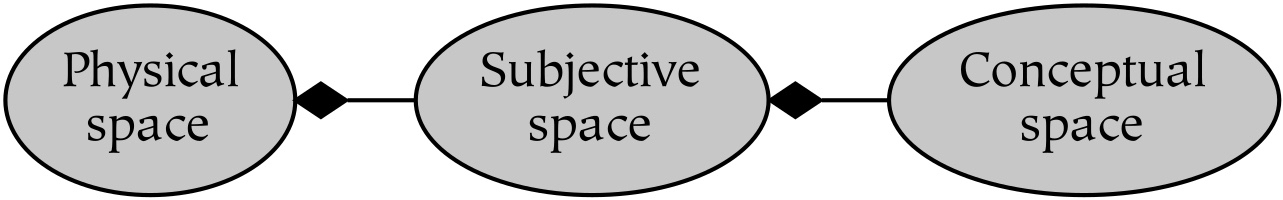
[Figure 3b: Depicting spaces as parts
of one another.]
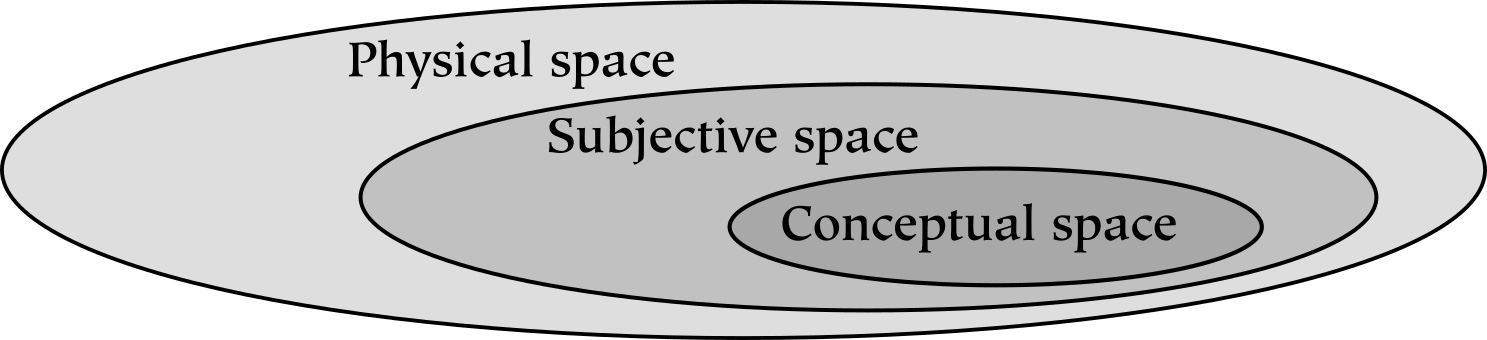
[Figure 3c: Depicting spaces contained
within one another.]
Using the second type of notation (UML), these three spaces can also be depicted as referential parts of each other, as in Figure 3d.
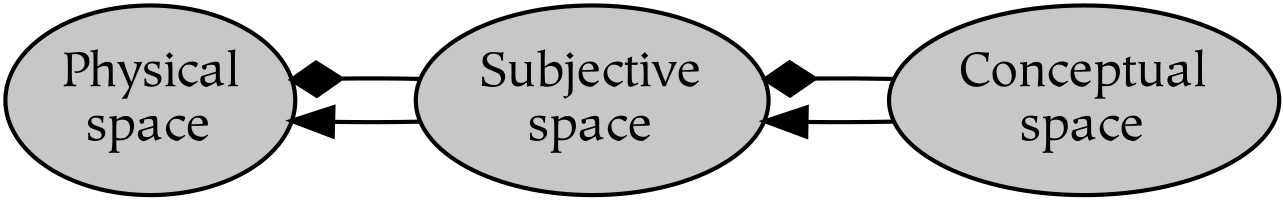
[Figure 3d: Three spaces as referential parts.]
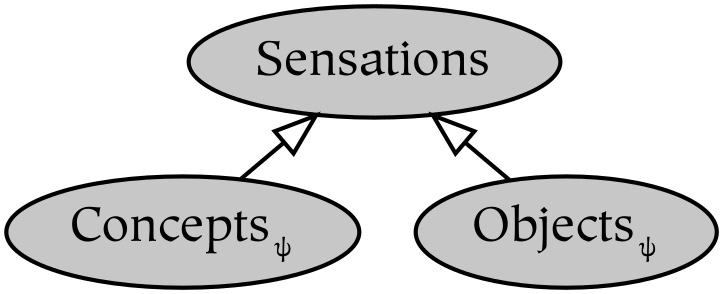
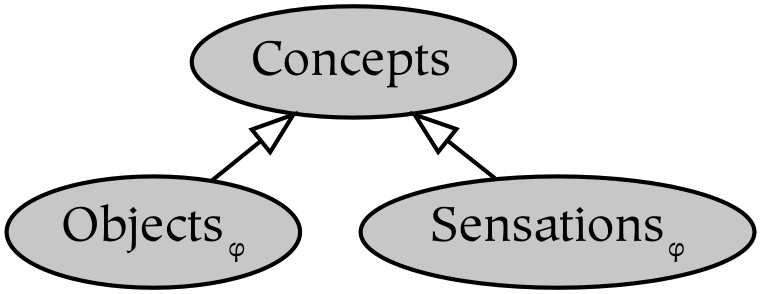
When these spaces are viewed as parts of one another, the complements of those parts with respect to their wholes creates two further spaces, objective space and sensory space. By definition, therefore, objective space is not sensed (O=U-S) and sensory space is not conceptualized (N=S-C). It is also useful to distinguish a symbolic space that is a specialized part of sensory space, the parts of which are symbols that reference concepts. These three further spaces may be summarized as follows:
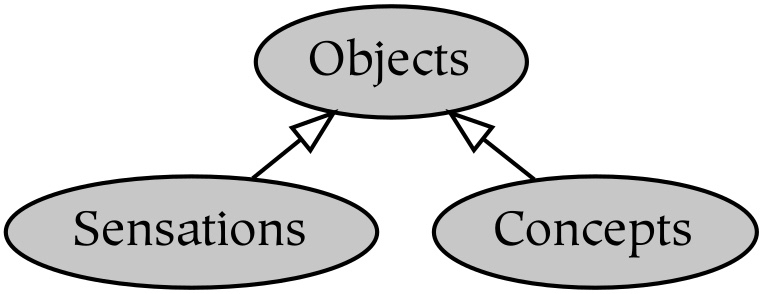
Objective space (O) is the space within physical space that is composed of all events that are not subjectively experienced (i.e., they are unobserved events in the physical universe, independent of the observer). Its parts are called objects.
Sensory space (N) is the space within a subjective space that is composed of all subjective experience that is not conceptual, such as feelings and emotions. It is composed of sensation.
Symbolic space (V) is the subspace of sensory space that consists of references to concepts. Its parts are called symbols.
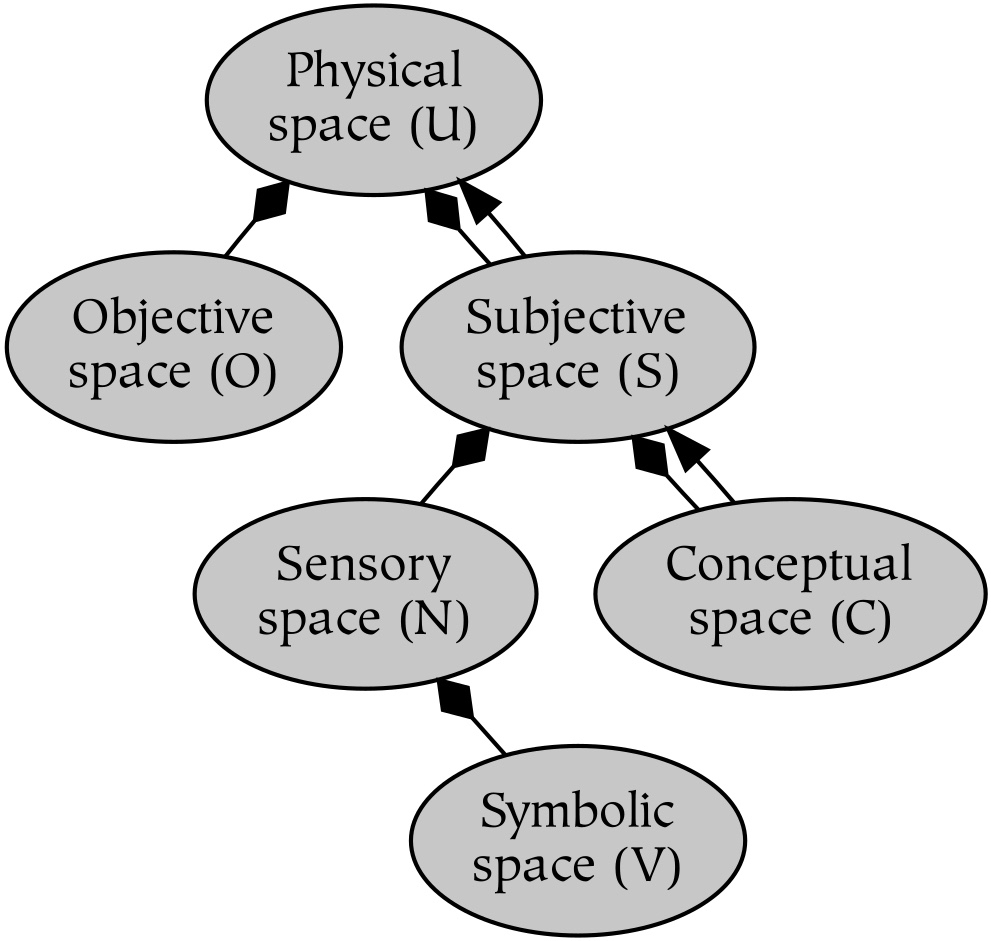
[Figure 3e: The six spaces (U, O, S, N, C, V),
shown as referential parts of one another. ]
Diagrammatically, all six spaces are depicted in Figure 3e. The objective (O), sensory (N), and conceptual (C) spaces, and the relations between them, form the basis for the basic model of cognition that is used throughout the book. They form a partition of physical space that is defined by two dichotomies: the subjective/objective dichotomy and the sensory/conceptual dichotomy.
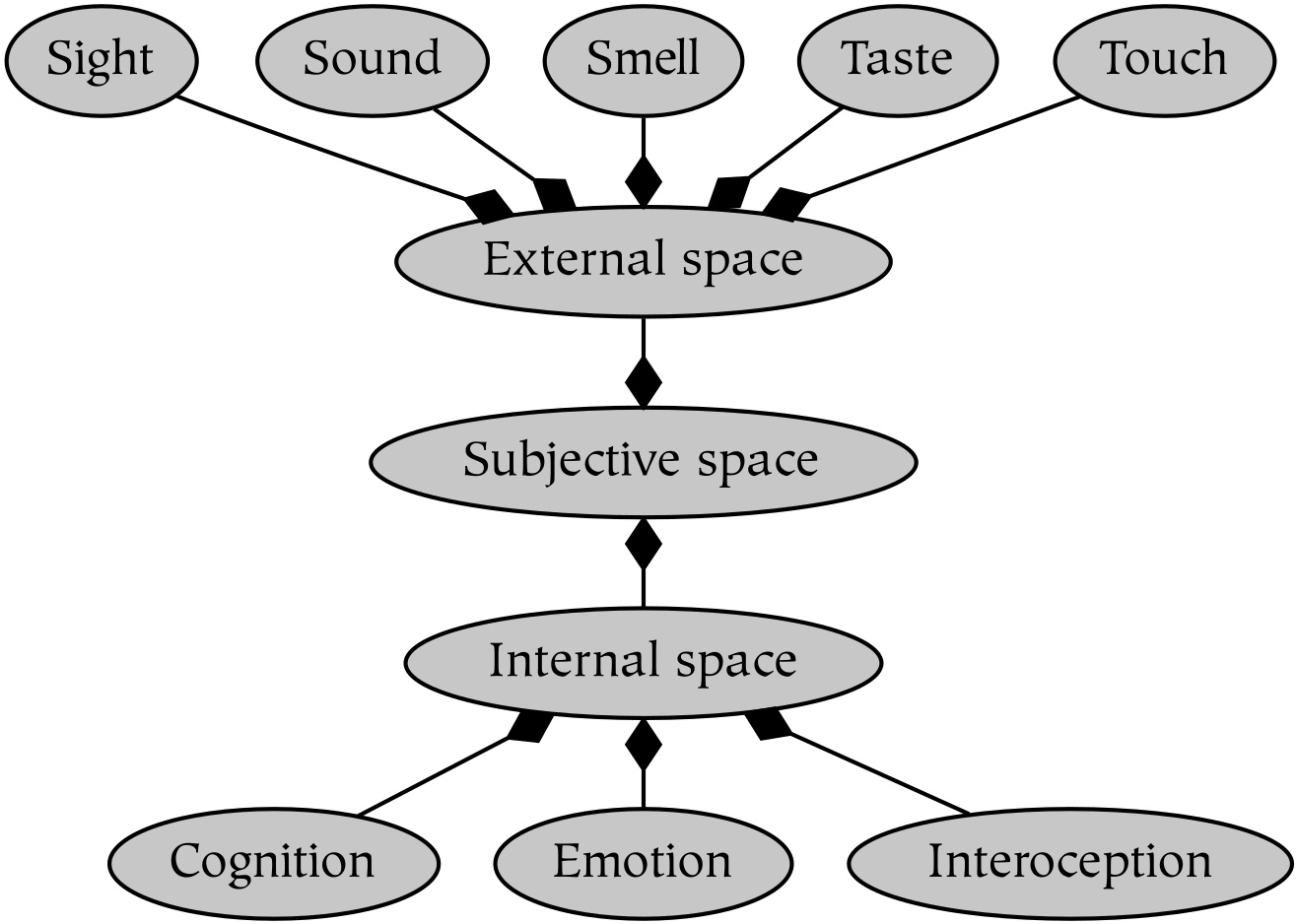
The subjective/objective dichotomy is the basis for two important relations, sensation and action:
These relations are depicted in the context of objective and subjective space in Figure 3f. As a model of cognition, this corresponds closely to behaviorism, although stimulus and response are replaced with the more humanistic terms sensation and action. Further, the model developed here presents subjective space as a mind with a subjective point of view, rather than as an object or animal.

[Figure 3f: The relations between
the subjective and objective spaces.]
The sensory/conceptual dichotomy divides subjective space into conceptual and nonconceptual subspaces, which is represented in Figure 3g. As mentioned, subjective space consists of references to physical space. Within subjective space, the relations of the references between the conceptual and sensory spaces represent the mereological and referential relations of objects in the world.
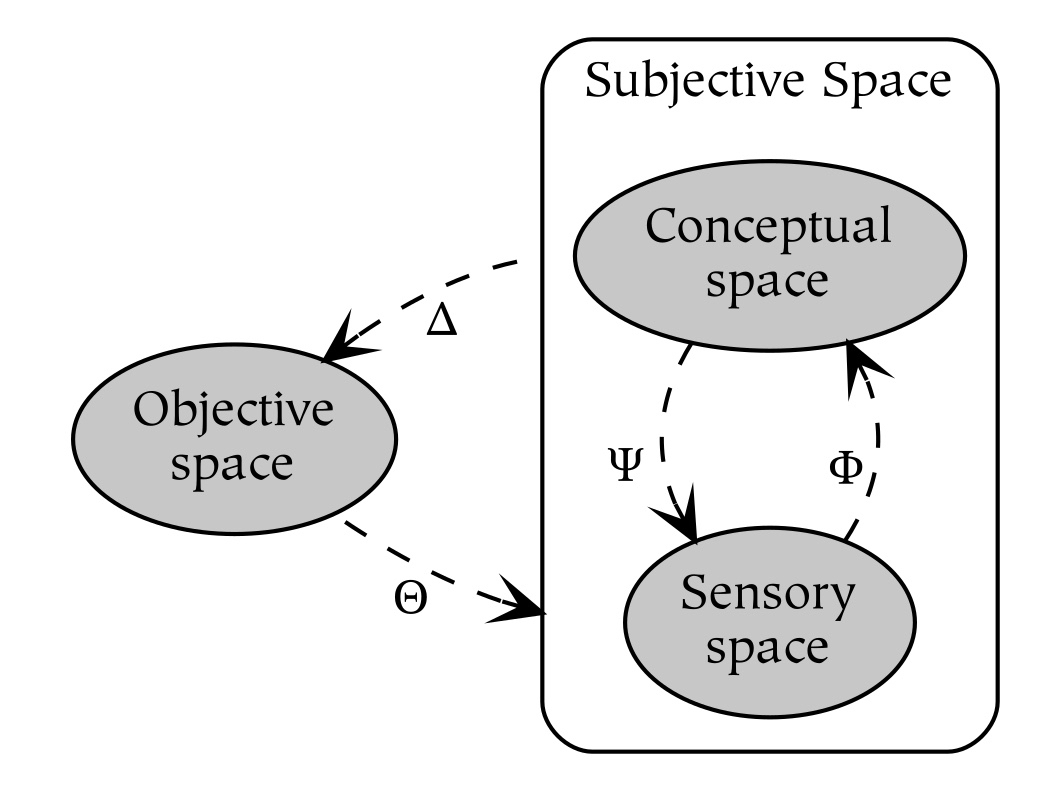
[Figure 3g: Dividing subjective space into
sensory and conceptual spaces.]
Sensory and conceptual spaces, although they are modeled as discrete entities, form a continuum whose content is sensation, and which is collected into increasingly large conceptual wholes as one moves from sensory space to conceptual space. Movement in the opposite direction is also possible, which entails creating increasingly sensory perceptual parts from conceptual wholes. These two operations are called conceptualization and visualization, respectively. Conceptualization and visualization correspond to the mereological operations of whole and part:
Conceptualization (Φ) is an act of whole-making, which creates concepts out of sensation.
Visualization (Ψ) is an act of part-making, which creates sensation out of concepts.
In addition to these mereological relations corresponding to whole and part, there are two referential relations which capture symbolic relations between conceptual and sensory space (or more specifically, between conceptual and symbolic space):
These two relations associate concepts and symbols, and thus map between conceptual space and symbolic space. Because symbols are modeled as sensations with symbolic meaning, the symbolic space that they form is a subspace of sensory space. Hence, symbols are significantly different from concepts; while concepts provide understanding in virtue of being wholes of sensory content, symbols are representations that correspond to words and enable language and thought (symbols are explored further in section Symbolic Space).
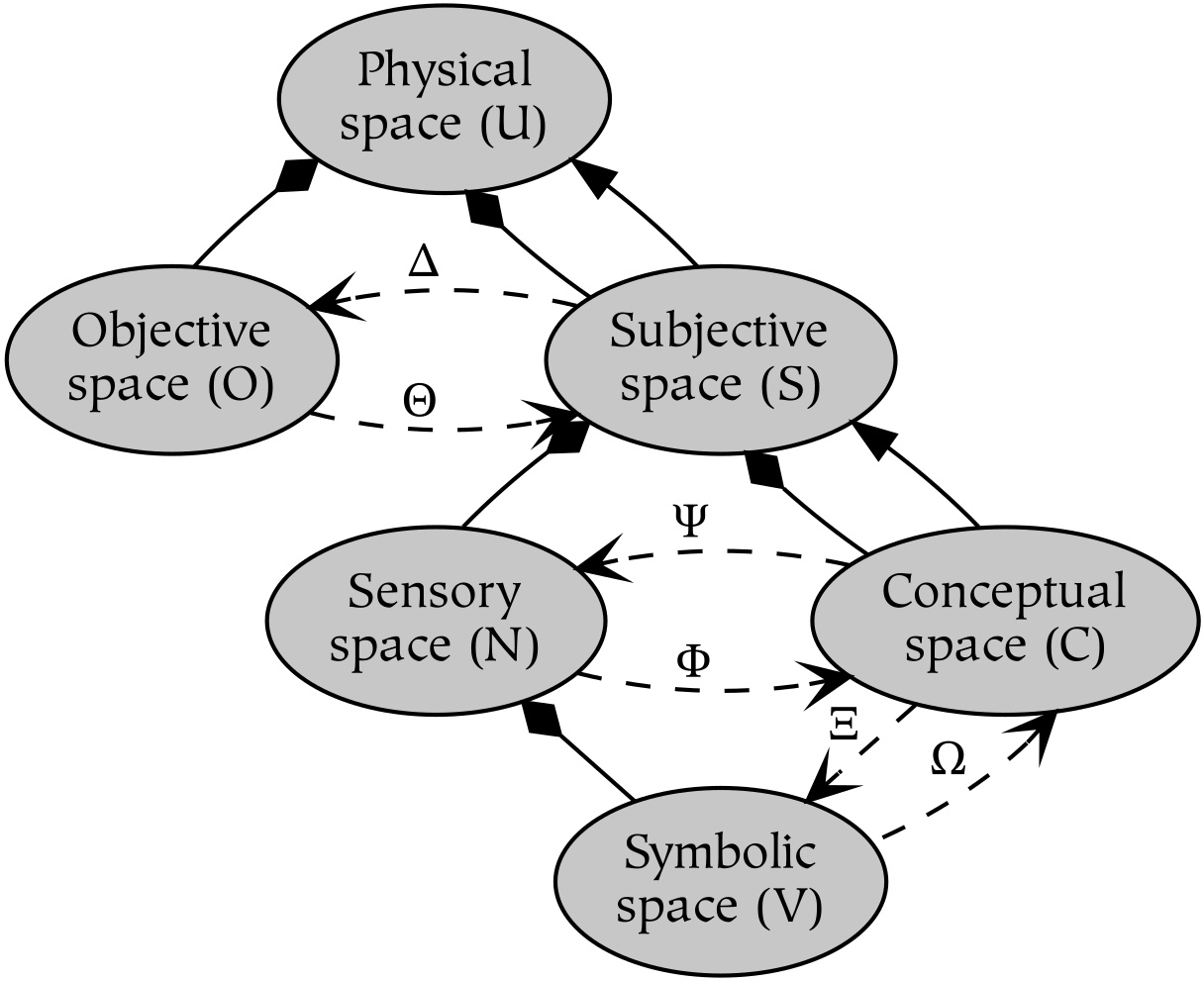
[Figure 3h: The basic model in full detail, with relations:
sensation (Θ), action (Δ),
conceptualization (Φ), visualization (Ψ),
interpretation (Ω), and symbolization (Ξ).]
Combining Figures 3e and 3g and adding the interpretation and symbolization relations yields the model shown in Figure 3h.
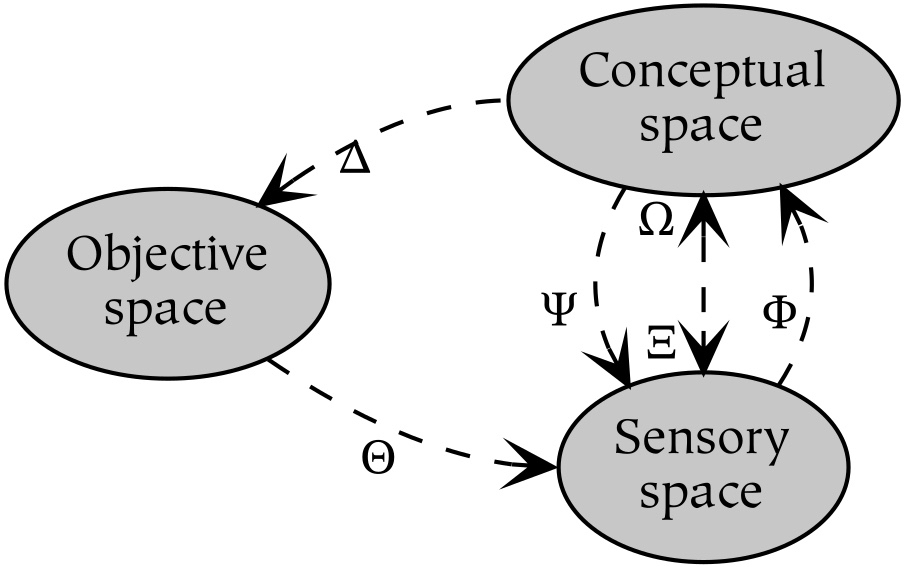
[Figure 3i: The basic model of cognition.]
In the interest of simplicity, however, this diagram is reduced to the basic model of cognition shown in Figure 3i. The three nodes represent mutually-exclusive spaces, and the edges (or dashed arrows) depict the flow of information between those nodes.
Chapter 6
Epistemic Universes

A presentation of three epistemic universes. The next three chapters examine the physical, subjective, and conceptual spaces consecutively. Since each space is boundless from its own point of view, they are also referred to as universes. For example, the subjective universe is a complete whole or totality in so far as all things can be experienced, and a given individual can never have experience outside of it. Similarly, conceptual space is also a universe in that all things can be conceptualized. That said, they are universes determined by the subjective perspective, so things that form a subjective universe from one point of view do not do so from another.
For clarity, it is important to explicitly specify the intended epistemic space of things that exist in multiple epistemic spaces. For example, the idea of an orange, the sensation of an orange, and the orange itself must be carefully distinguished from each other. Therefore, this book follows a convention for referring to the different types of entities as that is depicted in the taxonomy of Figure 3.6a, where the root node (a thing) is a generic type that can be used in any epistemological space, and its subtypes are scoped to each subsequent epistemic space (which mirrors the spaces as depicted in Figure 3e).
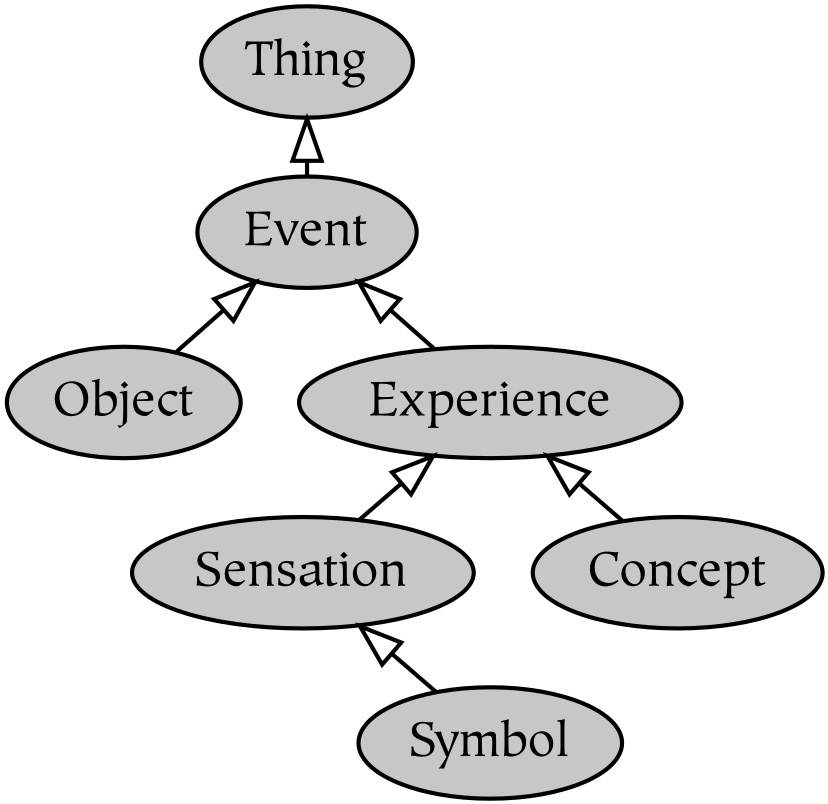
[Figure 3.6a: The types of things within each epistemic space.]
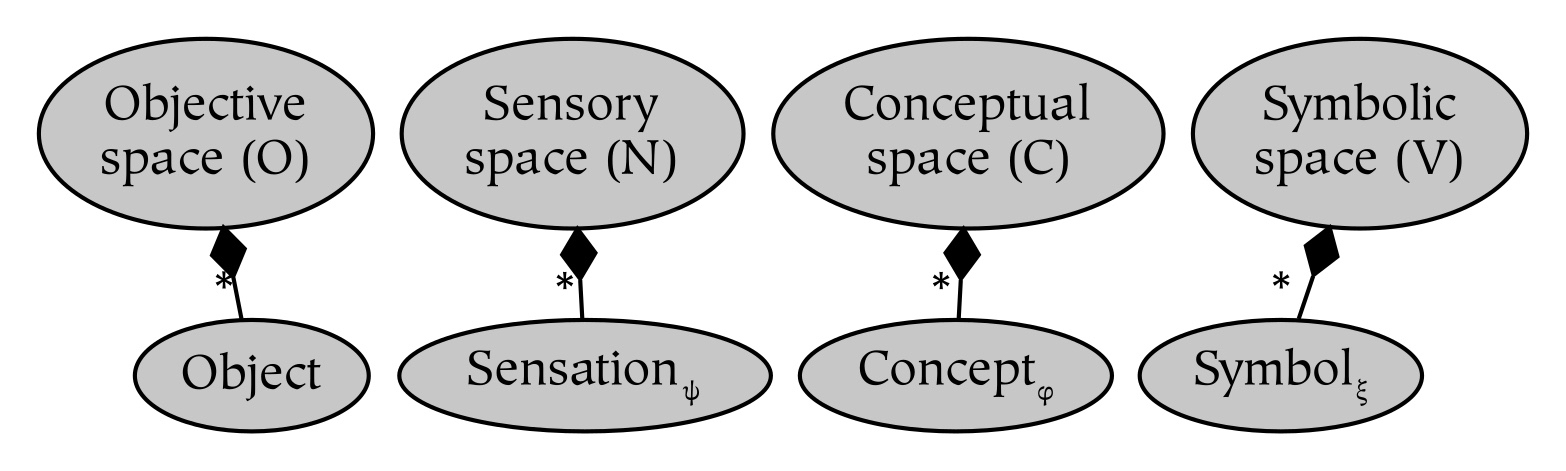
[Figure 3.6b: The parts of objective, sensory, conceptual, and symbolic spaces are respectively known as
objects, sensations, concepts, and symbols.]
Epistemic types corresponding to four of those spaces are illustrated in Figure 3.6b. The subscripts (or lack thereof) designate their associated entities as either objects, sensations, concepts, or symbols. For example, orangeφ refers to the concept of an orange (for more details, see appendix Typographical Conventions).
Chapter 7
Epistemic Relations

Six epistemic relations are defined between the objective, sensory, and conceptual spaces. This chapter looks at the six relations between the three epistemic universes of the basic model. These six relations may be summarized as follows with respect to the objective (O), sensory (N), and conceptual (C) spaces, and where symbolic space (V, a subspace of sensory space) is denoted explicitly:
Sensation (Θ): Sensation is a causal function. It changes N as a result of O.
Action (Δ): Action is a causal function. It changes O as a result of C.
Conceptualization (Φ): Conceptualization is the whole function. It creates increasingly conceptual wholes as one moves from N to C.
Visualization (Ψ): Visualization is the part function. It creates increasingly sensory parts as one moves from C to N.
Interpretation (Ω): Interpretation is the referent function. It activates referents in C in virtue of symbolic references in V.
Symbolization (Ξ): Symbolization is the reference function. It activates symbolic references in V in virtue of referents in C.
3.7.1 Sensation
Sensation affects sensory space as a result of
changes in objective space.
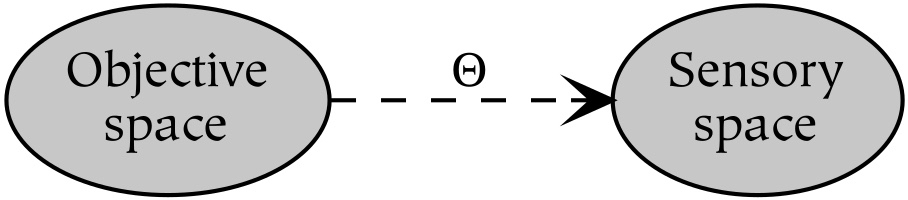
[Figure 3.7.1: Sensation (Θ) is the dual of action.]
There are at least two important points to note about sensation, which creates sensations in sensory space from objects in objective space.
The first is that it creates a mass of sensation, rather than individuated sensations. Sensation is individuated, combined, and divided by subsequent conceptualization and visualization; new sensation is not produced in this process. In other words, the entire content of our experience of the world is provided via sensation (e.g., sensation includes things like feelings and emotions).
The second is that sensation is the dual of action. Just as action causes effects in the world, sensation causes effects in us. However, the English grammar describing both events implies that the person is the agent, since the person both senses and acts. This asymmetry is unfortunate, since sensation is caused by the action of the external object (i.e., it is similar to saying that a baseball senses the bat that hits it, when in fact it doesn’t have much of a choice in the matter).
3.7.2 Action
Action affects objective space as a result of
changes in conceptual space.
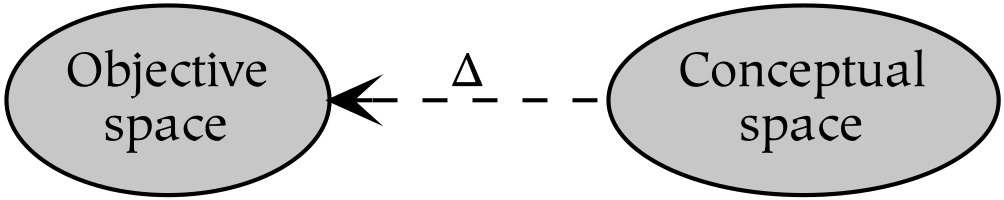
[Figure 3.7.2: Action (Δ) is the dual of sensation.]
Action changes the physical world according to the conceptual intent of an individual, through acts such as walking or the creation of sound waves. When the actions are sounds such as spoken words, action may also convey interpersonal symbolic content.
3.7.3 Conceptualization
Conceptualization creates conceptual wholes
from sensory parts. The creation of concepts entails the bottom-up collection of sensation and concepts into a single whole. Concepts can also be composed of symbols, in which case they are called higher-order concepts. Conceptualization can generally operate in parallel, although emotional attachment and top-down symbolic activation may enforce serial operation.

[Figure 3.7.3: Conceptualization (Φ) is the dual
of visualization.]
3.7.4 Visualization
Visualization creates sensory parts
from conceptual wholes.
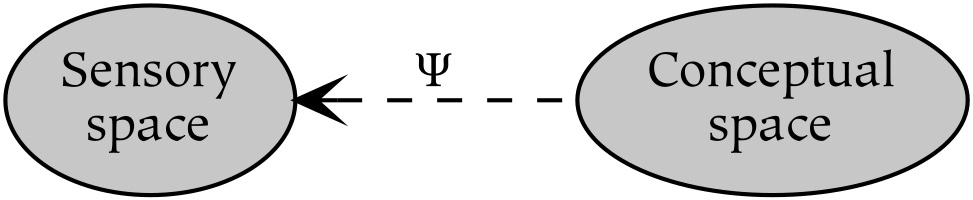
[Figure 3.7.4: Visualization (Ψ) is the dual
of conceptualization.]
Visualization entails the top-down projection of concepts to sensory space. It may be seen as a transformation of concepts back into sense data, or at least a transformation of percepts from a higher to a lower epistemic level. It therefore operates in a direction opposite to conceptualization.
Visualization is not necessarily visual, although visual imagery is often used to describe this process (as in the word imagination). Since partial visualization makes conceptual parts out of larger conceptual wholes, but does not necessarily create “raw” sensation, it is similar to understanding. Visualization is capable of turning multiple concepts into a single concrete unit, which may be the mechanism behind the psychological process of chunking.
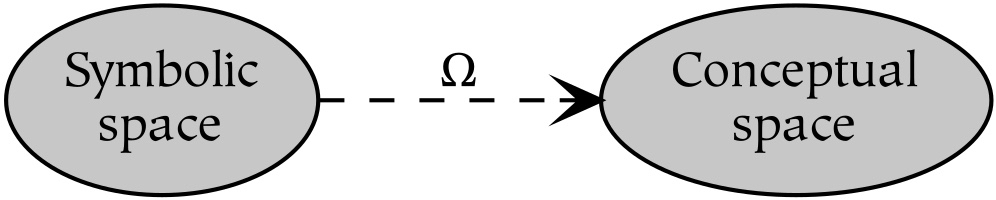
3.7.5 Interpretation
Interpretation activates concepts in conceptual space
from symbols in symbolic space.
[Figure 3.7.5: Interpretation (Ω) is the dual of symbolization.]
Interpretation is an act of dereferencing that creates meaning from a sensory reference. These perceptual references are known as symbols. In the context of cognition, they may also be called cognitive symbols to differentiate them from verbal or written symbols.
Interpretation entails the activation of a concept based on its name. In other words, symbols are symbolizations of their corresponding concepts, and concepts are interpretations of their corresponding symbols. Interpretation is therefore similar to conceptualization, but unlike conceptualization, the interpretation of a sensation is not a mereological whole of that sensation.
3.7.6 Symbolization
Symbolization creates symbols in symbolic space
from concepts in conceptual space.
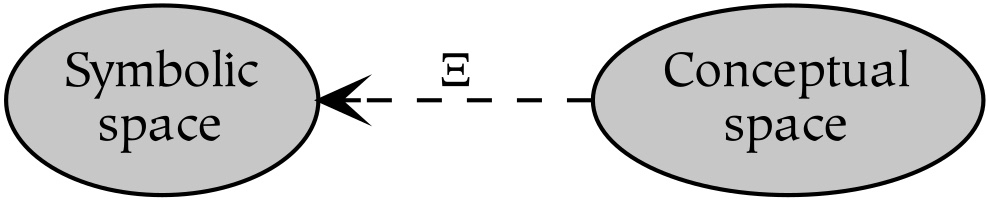
[Figure 3.7.6: Symbolization (Ξ) is the dual of interpretation.]
Symbolization is an act of naming that takes place between a sensation (or the reference) and a concept (or the referent). The sensation becomes the name or symbol for that concept, and thus forms the basis for symbolic thought. For example, the vocalized word earthξ is the name for the concept earthφ.
Symbolization is the dual of interpretation. It resembles visualization in that it produces sensation (i.e., the symbol) from a corresponding concept, but it is different in that the sensation it creates is not a mereological part of that concept. It is perhaps better understood as an internalized shorthand for an action followed by the sensation resulting from that act (e.g., such as occurs in the process of subvocalization and hearing when learning words).
Chapter 8
Recursion

The interaction of subjective spaces and their relations creates higher‑order cognition and symbolic spaces. Symbols are references to concepts that form symbolic space. Symbols are specialized types of sensation: for example, the word windξ is a part of our sensory space that becomes a symbol for the concept windφ. The combination of concepts that are composed of sensation and symbols that refer to concepts establishes the basis for a referential recursion between sensory and conceptual spaces that is illustrated in Figure 3.8a.

[Figure 3.8a: Symbolic space is the part of sensory space
that references conceptual space.]
Figure 3.8b depicts an unrolled version of this sensory/conceptual recursion. Each node’s label is annotated with a superscript indicating the conceptual order, and to the right of each node is a number that indicates the epistemic level.
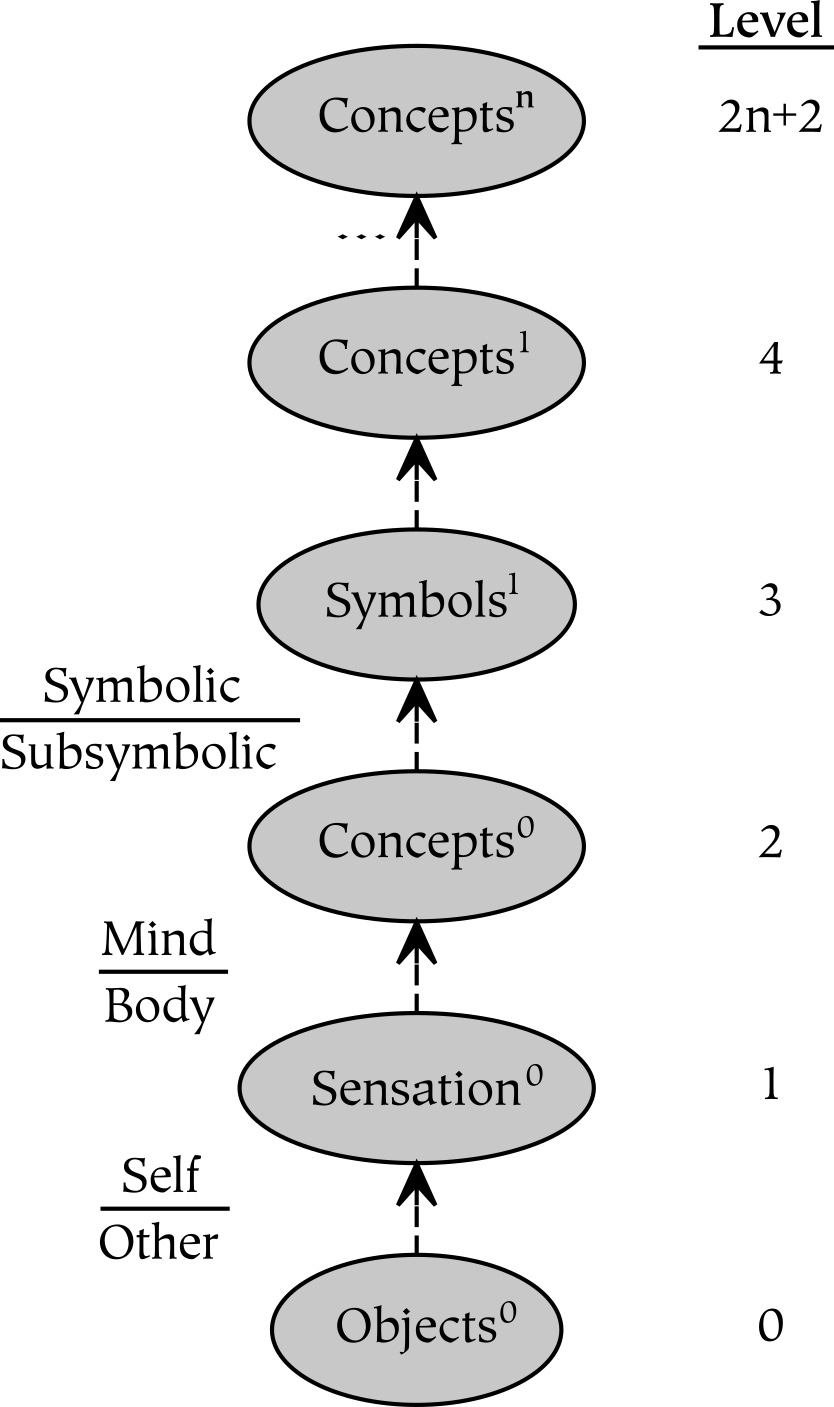
[Figure 3.8b: Epistemic level indicates distance from ground.]
The left side of Figure 3.8b shows the approximate locations of three popular epistemic divisions, each of which traditionally corresponds to a mereological distinction rather than a purely referential distinction:
Self/Other: The self/other distinction epistemically corresponds most closely to the distinction between sensations and objects.
Body/Mind: The distinction between body and mind is approximately the division between nonconceptual and conceptual.
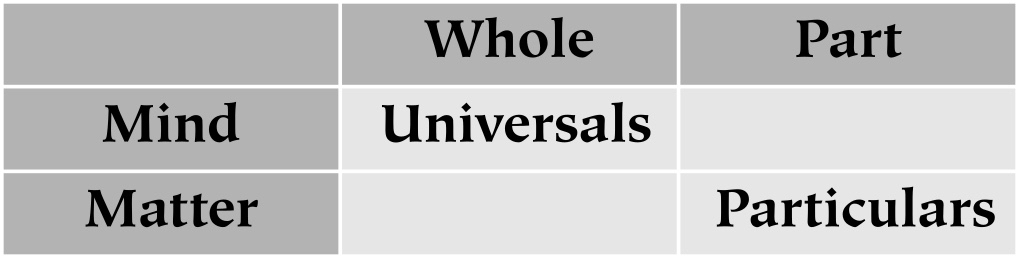
Subsymbolic/Symbolic: The distinction between subsymbolic and symbolic mental content is made at the level of symbols. This is also the division between abstract universals and concrete particulars.
The loop that is formed by conceptualization (Φ) and symbolization (Ξ) allows human minds to create symbolic hierarchies. This loop enables recursive composition, whereby conceptual wholes are represented by symbols, and those symbols in turn form the basis for subsequent conceptual wholes. These hierarchies can be understood by reversing this process, which entails interpretation (Ω) and visualization (Ψ).
In linguistics, the hierarchies thus constructed correspond to the deep structure of language (see [Chomsky, 1995]). In mathematical set theory, there is an analogous structure called the Zermelo or von Neumann Hierarchy. Cognitively, the construction of higher-order concepts out of previously existing concepts is leveraged both long-term to define words as higher-order concepts and short-term to understand sentences that are dynamically-constructed.
The depth of these hierarchies can be quantified using the notion of epistemic level. Epistemic level is a measure of the number of transitions (or arrows) that must be traversed to reach ground, which has an epistemic level of zero by definition. Epistemic level therefore increases every time things are sensed, conceptualized, or symbolized, and decreases with every act, visualization, or interpretation.
3.8.1 Symbolic Space
Symbolic space is the space of symbols,
or sensations that reference concepts. Symbolic space is the subspace of sensation whose parts are interpreted symbolically. Therefore, symbolic space is literally a mental sense, which is distinct from the conceptual understanding to which it corresponds.
Cognitive symbols (or simply symbols) are meaningful in terms of both their conceptual content and their relation to other symbols. The relation of symbols to one another is determined by their common wholes, and is explored further in section Language.
3.8.1.2 Symbolic Parts
The parts of symbolic space are called symbols. An essential characteristic of symbols is that they must activate (or re-present) the same concepts as does perceiving the physical object which they designate. However, unlike the sensations that trigger that concept, they are not required to resemble that object at a sensory level.
Because symbolic understanding destructively interferes with the bottom-up perception of environmental stimuli, symbols must inhibit those competing stimuli in order to activate their intended meaning. This process of preventing irrelevant bottom-up activation from interfering also prevents any other symbols from being simultaneously active. As a result, symbols are understood serially rather than in parallel.
3.8.1.3 Symbolic Dimensions
Because symbols are discrete references, their
collection into wholes forms a discrete space. Symbolic space is composed of discrete (symbolic) references. In other words, since references are not divisible in a meaningful way, symbols correspond to discrete atoms within symbolic space. As such, they may be treated as geometric points, although they have unit extent rather than zero extent. An example atom is depicted in Figure 3.8.1a, where it is assumed that the atom is not mereologically divisible (despite its apparent volume).

[Figure 3.8.1a: An atom (zero discrete dimensions).]
Symbolic dimensions, or the dimensions formed by collecting symbols within a referential space, can be synthesized by combining these referential atoms. For example, a reference can be combined with references to its left and right, thereby forming a line of references. This is depicted in Figure 3.8.1b, where an atom iterated in an arbitrary direction results in a line.

[Figure 3.8.1b: A line (one discrete dimension).]
This process can be repeated: a line creates a plane when iterated in a direction orthogonal to its length, as shown in Figure 3.8.1c. Similarly, many planes collected along an orthogonal dimension form a 3‑D cube, as in Figure 3.8.1d.

[Figure 3.8.1c: A plane (two discrete dimensions).]

[Figure 3.8.1d: A cube (three discrete dimensions).]
In the physical universe, the fourth dimension is called time (at least by physicists). As with the previous dimensions, a novel dimension is created by iterating a lower-dimensional object along a new axis that is orthogonal to the existing ones, as in Figure 3.8.1e.
The depiction of a 5-D object is a particularly interesting example of how each successive dimension is produced, because few people have explicitly extended their conceptualization (or visualization) of dimensionality that far. Implicitly, however, the fifth dimension is used frequently as the measure of possibility. The fifth dimension is not a difficult concept to grasp when understood in this way, although it is not explicitly treated as a spatial dimension.
[Figure 3.8.1e: A timeline (four discrete dimensions).]
To conceptualize the five-dimensional world, it is helpful to imagine another earth that is similar to ours, which exists at the same place and time (or at the same spatial and temporal coordinates), but which occupies a different fifth dimensional coordinate. In philosophical terms, it is a possible world instead of the actual one. Visually, since four dimensions are represented by a world-line, the fifth dimension can be represented by multiple world-lines, as shown in Figure 3.8.1f.
[Figure 3.8.1f: Multiple timelines (five discrete dimensions).]
Because the fifth dimension enables the discussion of possible worlds, it is referred to as the dimension of modality. Support for the hypothesis that the world is at least 5-D, or that objects exist in multiple possible states at once, is given by experiments in physics such as the double-slit experiment.
3.8.2 Higher‑Order Concepts
Higher‑order concepts are concepts
that are composed of symbols.
[Table 3.8.2a: Two Kinds of Concepts.]
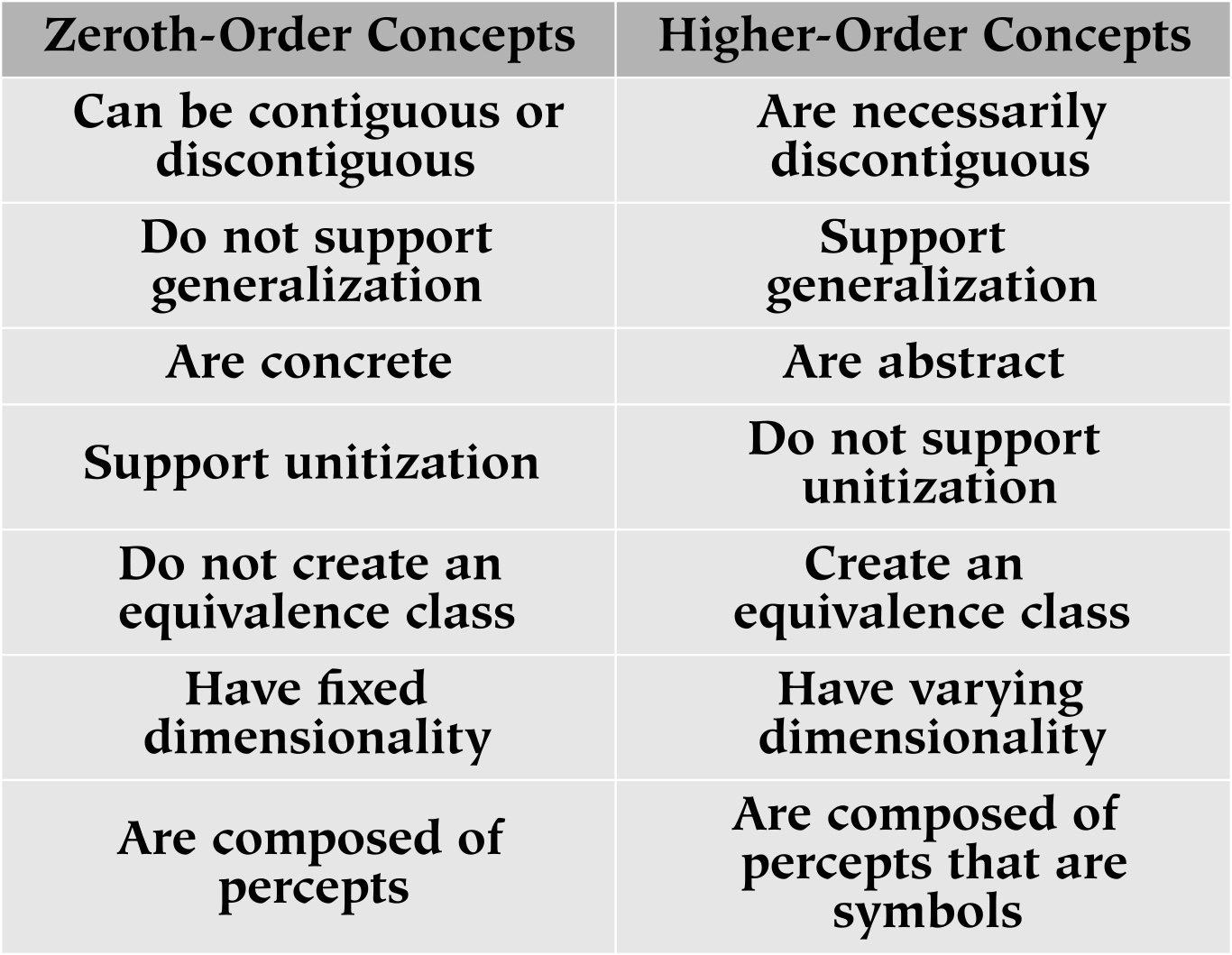
Concepts may be either concrete or abstract. Concrete or zeroth-order concepts are conceptual wholes that are constituted by sensation or by other concrete concepts directly. Abstract or higher-order concepts are wholes of symbolic references, and as such, their order is one more than their constituent concepts. For example, if treeφ is defined as the abstract conceptual whole composed of pineξ and mapleξ, and both pineξ and mapleξ are symbols standing for first-order concepts, then the nominal dimension used to contain these two symbols makes treeφ a second-order concept.
The ubiquity of concepts in different disciplines has led to an abundance of terminology. For example, the following terms are at least approximately synonymous with concepts: wholes, generalizations, unitizations, categories, abstractions, generalities, sets, classes, and equivalence classes. Since the meaning of these terms is imprecise given their somewhat differing use in multiple contexts, Table 3.8.2a clarifies how some of those terms are used here. Several of these terms require further comment, especially with respect to the critical distinction between concrete and abstract concepts:
Contiguity: Higher-order concepts are formed by collecting category members; therefore, they are conceptually granular or chunky. They also tend to represent objects that are discontiguous, since contiguous objects can be represented with zeroth-order concepts.
Unitization: Unitization refers to the process of making successive wholes (or unions of parts) without increasing the order of the concept. It is thus the conceptual operation that corresponds to creating meronomies from concrete parts. For example, the concepts headφ, torsoφ, armsφ, and legsφ may constitute the first-order concept of a bodyφ without requiring any intermediary symbols. Although it is possible to form a meronomy of abstract parts, this is generally not intended (this is explored further in the next section, Cognitive Taxonomies).
To understand the difference between unitizations and generalizations, imagine an apple and an orange. The unitization of these two (i.e., their zeroth-order concept) is the union of all sensations of the apple and the orange; it is a concrete, mereological fusion. On the other hand, the abstract generalization of apple and orange concepts is the intersection of their corresponding ideas, or all parts and wholes of apples and oranges.
Abstraction or generalization: Abstraction entails the formation of a categorical prototype, whose properties are common to the constituent concepts of that category. As mentioned previously, when zeroth-order concepts are formed, they are wholes of their composite sensation. Therefore, they are concrete, just as that sensation is concrete. However, when higher-order concepts are understood, the necessary visualization of their constituent symbols makes them abstract.
Symbols become abstract when an intersection of their conceptual content is formed to isolate specific properties. For example, the property greenφ may be isolated from green trees and green moss by forming the intersection of the constituent properties of those objects. Similarly, the meaning of the higher-order concept “pet dogs”φ involves the intersection of properties belonging to petφ and dogφ ideas, which makes sense even to a person who knows what pets and dogs are, but who has never physically encountered a pet dog.
The reason that higher-order concepts form intersections is that symbols are visualized by negating the opposite of their referent concepts, where the opposite means all concepts which are not parts or wholes of the given concept. When two symbols are combined in this top-down manner, the resulting concept exists as the intersection of the two ideas. This process makes a concept abstract if the intersection lacks any location, which happens when the constituent concepts have disjoint spatial locations.
However, it is not true that the visualization of concepts is always abstract. Take as examples the zeroth-order concepts chairφ1 and chairφ2, which are composed of the union or unitization of the sensations derived from two chairs, chair1 and chair2. There may be a composite zeroth-order concept, chairφ3, that exists as the conceptual unitization of those two chair concepts. Understanding chairφ3 entails only visualization of that union, rather than forming the intersection of two interpreted symbols. Therefore, the resulting concept remains concrete, unlike the higher-order concept that is formed out of the two symbols chairξ1 and chairξ2. To reiterate, although the physical intersection of two distinct concrete entities is necessarily empty, the intersection of the chairφ1 and chairφ2 ideas is not necessarily empty because they share a number of abstract wholes or properties (such as being places to sit).
The combination of these examples means that concepts can be defined both in an absolute sense (via zeroth-order, concrete sensation) and in a relative sense (via higher-order, abstract concepts), which corresponds to the fact that things in the world are known by both experience and definition. In practice, since few concepts exist purely in a zeroth-order or higher-order way, it is impossible to assign them a unique order.
3.8.2.1 Cognitive Meronomies
Knowing entails structural relations between concepts. To know the relations between objects in the world entails structuring the references to those objects in a particular manner. In order to examine the different ways in which this knowledge might be structured, imagine a world in which there is a cat named “Felix”, who has a paw (called “Felix’s paw”). Given a diagram of these conceptual entities, how can one represent that cats have paws? In other words, what is the structure of conceptual space such that its referential structure is isomorphic to the structure in the world between cats and paws?
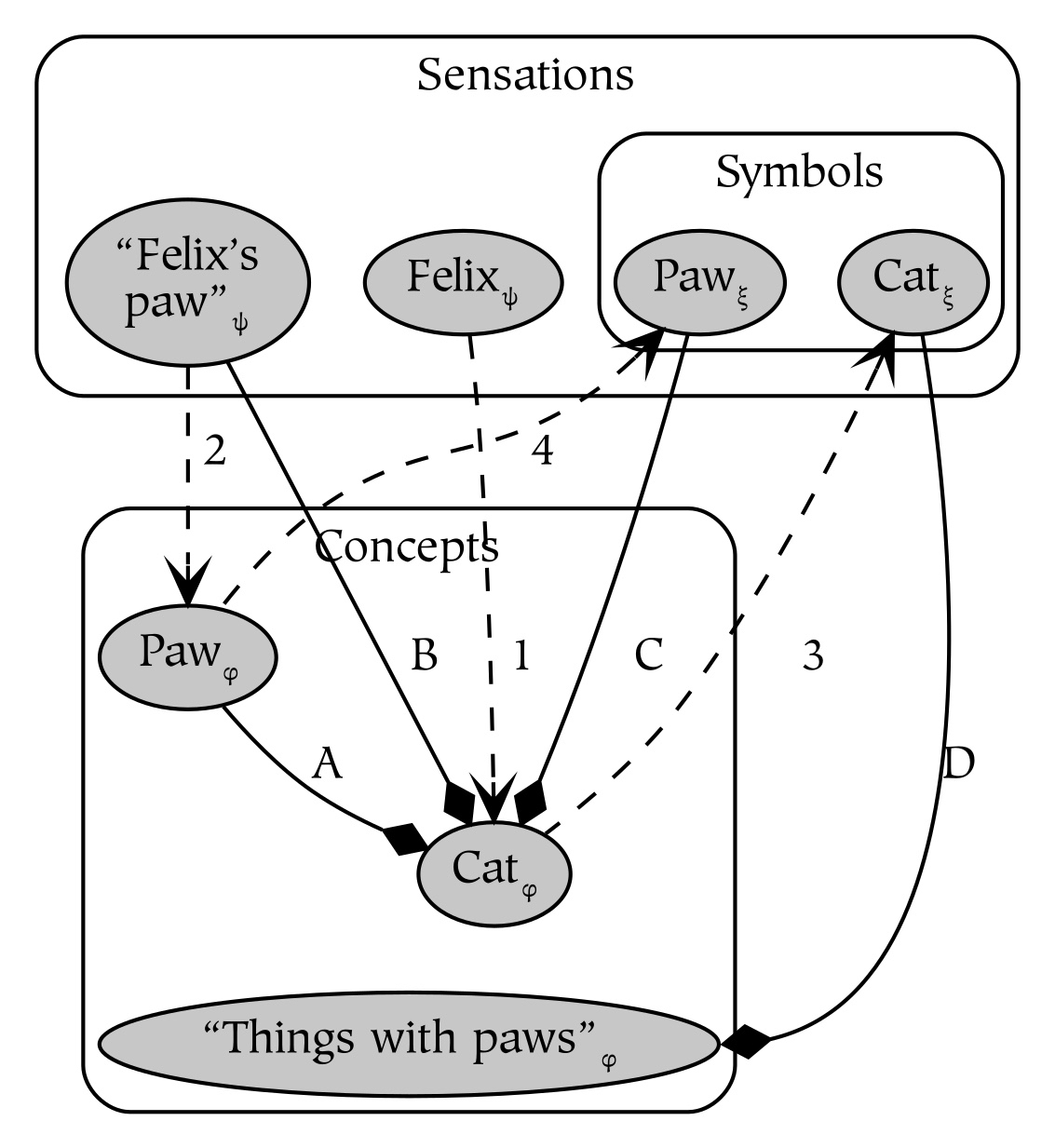
[Figure 3.8.2b: Arrows A–D illustrate several ways
of knowing that “cats have paws”.]
The cognitive structure in which we hope to represent this information is shown in Figure 3.8.2b, where the relations shown by dashed arrows indicate that:
1. catφ = Φ(Felixψ): Felixψ is a catφ.
2. pawφ = Φ(“Felix’s paw”ψ): “Felix’s paw”ψ is a pawφ.
3. catξ = Ξ(catφ): the name of the concept catφ is catξ.
4. pawξ = Ξ(pawφ): the name of the concept pawφ is pawξ.
There are at least several implementation options, which correspond to the solid edges in the diagram:
A. catφ is a whole of pawφ: This option seems like the most obvious answer. Together with relation (1), it creates concepts that are composed of both sensory and conceptual content. It is a relation between concrete entities, however, so it does not capture the universality of “cats have paws”.
B. catφ is a whole of “Felix’s paw”ψ: This option creates a concept catφ that is composed only of sensations (i.e., Felixψ and “Felix’s paw”ψ).
C. catφ is a whole of pawξ: The drawback of this option is that the concept catφ becomes constituted by both sensation (e.g., Felixψ) and symbols (e.g., pawξ), which makes it an uncomfortable amalgamation of different epistemic levels.
D. “Things with paws”φ is a whole of catξ: This option is slightly redundant because it involves a usage of paws which is not connected to the concept of pawφ.
E. (not depicted): In order to know if cats have paws, the existing concrete concepts can be visualized in sensory space, where the parthood relationship can be determined by inspection.
While option (E) may be necessary for visualization or intuition, it is not a solution to the issue of how to store knowledge between abstract concepts. The cleanest solution allows this relation to be modeled in two different ways, which express slightly different things. Options (A) and (B) express the relation “concrete cats have concrete paws” as a concrete meronomy, and option (D) expresses the relation “abstract cats have abstract paws” as an abstract taxonomy. One notable aspect of this pair of solutions is that there is no immediate connection between the concrete concept pawφ and the abstract property “things with paws”φ (this is not a very elegant finding architecturally, although it may not be incorrect from a cognitive perspective). Another notable aspect is that “things with paws”φ is typically modeled in prototype theory as a property, although in the basic model it is modeled as a symbolic (abstract) whole.
3.8.2.2 Cognitive Taxonomies
Higher‑order concepts are composed of symbols that reference concepts, rather than composing concepts directly. In order to explore how higher-order concepts such as “things with paws”φ are constructed, Figure 3.8.2c shows several relations and entities in the objective, sensory, and conceptual spaces. Objective space is depicted at the top level, which causes the sensations aψ, bψ1, and bψ2 in sensory space. These sensations are conceptualized as aφ and bφ in conceptual space. Finally, these concepts are united in a higher-order concept, cφ.
Figure 3.8.2c can be made more concrete by visualizing it as the description of a world that consists of one dog and one cat. Dog and cat concepts (aφ and bφ) are formed by sensing the dog once (aψ1) and the cat twice (bψ1, bψ2). The dog and the cat are both kinds of the abstract type animal (cφ).
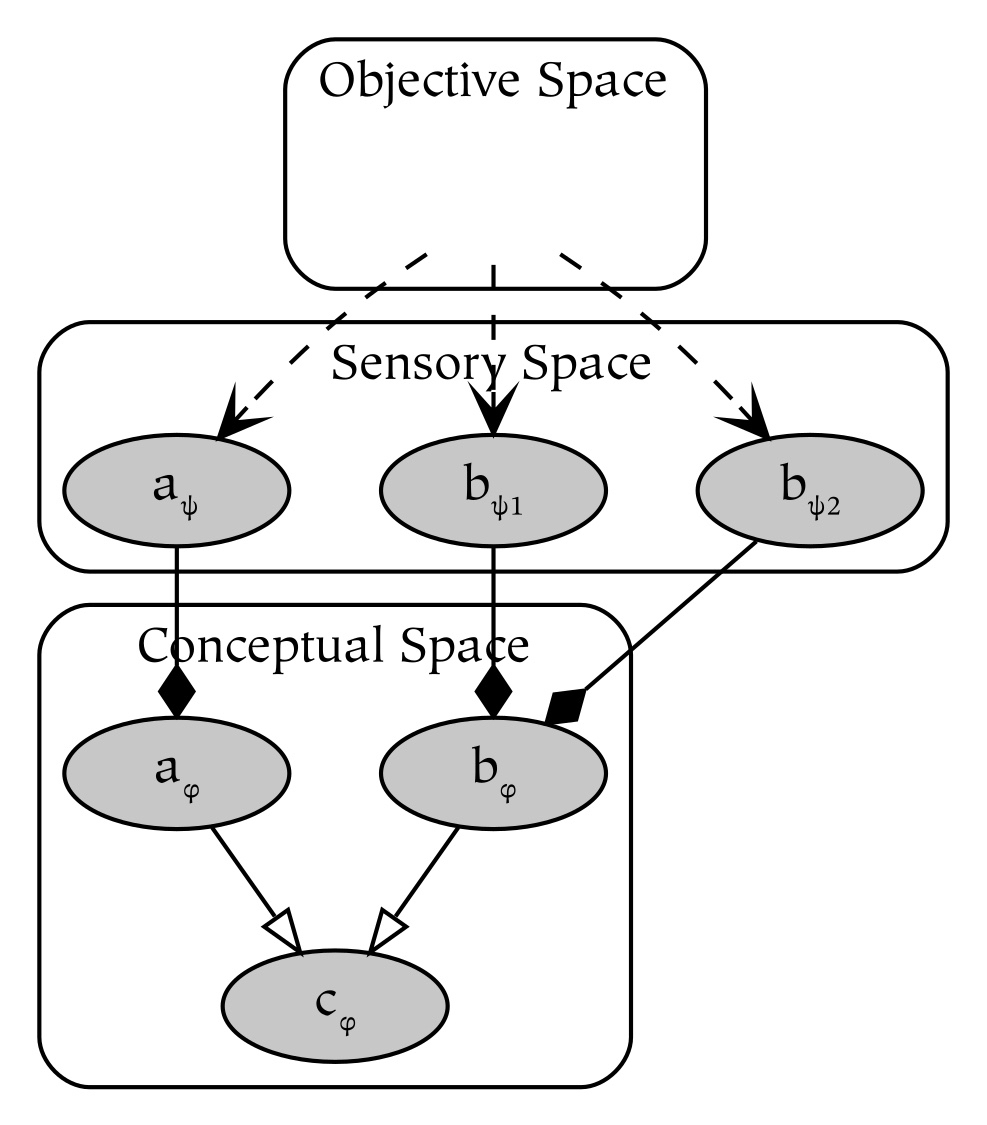
[Figure 3.8.2c: The structure of a higher-order concept (cφ).]
Although this diagram may accurately represent the structure of knowing, the generalization relation that is indicated by empty-triangle arrowheads that point towards cφ is a shorthand that has not been defined using the relations that exist in the basic model. Therefore, the rest of this section explores how the generalization relation can be implemented using wholes (conceptualization), parts (visualization), referents (interpretation), and references (symbolization).
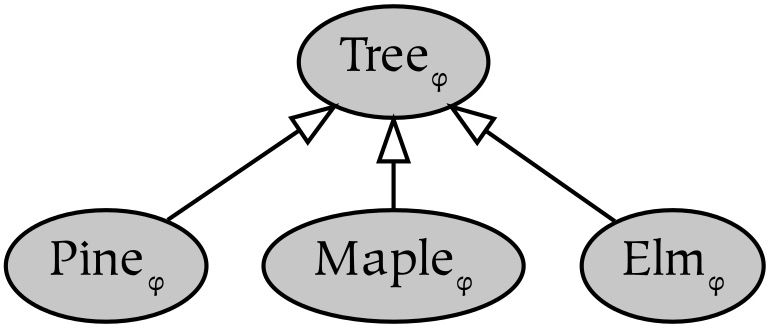
__[Figure 3.8.2d: A taxonomy using the undefined
generalization relation: __ **Treeφ = generalization( Pineφ, Mapleφ, Elmφ ).]**
Figure 3.8.2d depicts an abstract taxonomy. The most obvious choice for implementing this structure without the generalization relation uses the operation of whole to create a meronomy, although that does not capture the intended meaning because the leaf nodes are abstract. In other words, an abstract tree does not have abstract pines and maples as parts; rather, pines and maples are kinds of trees. Therefore, meronomies such as the one in Figure 3.8.2e are generally inaccurate, as discussed in section Meronomies.
[Figure 3.8.2e: An invalid conceptual meronomy with
abstract conceptual elements**
**Treeφ = whole( Pineφ, Mapleφ, Elmφ )]
Valid methods for implementing the taxonomy are illustrated in Figures 3.8.2f and 3.8.2g, each of which has different cognitive consequences. The first method is depicted in Figure 3.8.2f, and entails turning the conceptual wholes back into sense data and then collecting that sense data into a new conceptual whole.
[Figure 3.8.2f: A sensory meronomy**
**Treeφ = whole( Pineψ, Mapleψ, Elmψ )]

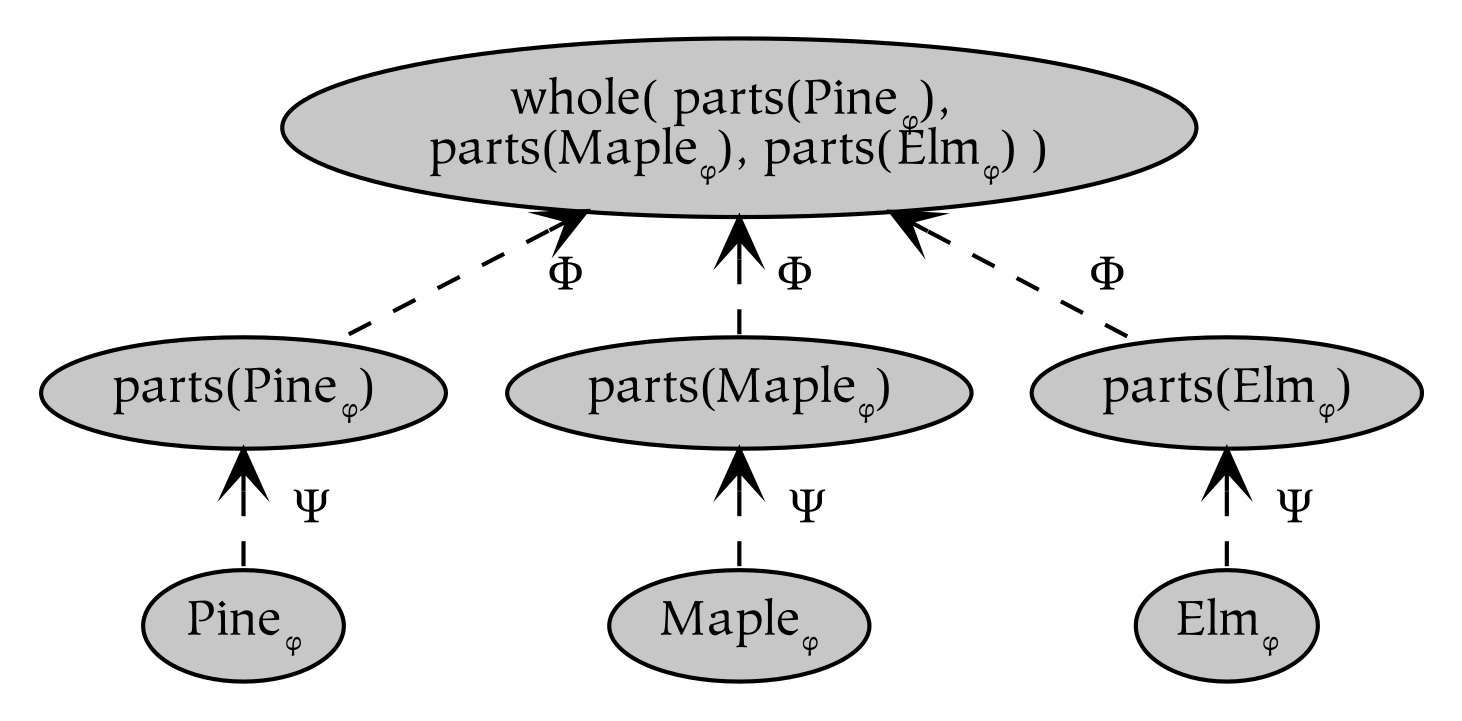
[Figure 3.8.2g: A symbolic meronomy**
**Treeφ = whole( Pineξ, Mapleξ, Elmξ )]
The second method is depicted in Figure 3.8.2g, and entails creating symbolic references to the wholes and then collecting those symbols into a new, higher-order conceptual whole. The difference between these two structures is that the first one does not increase the conceptual order of the concept treeφ. In other words, the treeφ of the sensory meronomy is of the same order as its constituents, while the treeφ of the symbolic meronomy is one order higher than its constituents as a result of creating symbolic references to them.
3.8.3 Language
Languages are dynamic systems for expressing
and understanding conceptual space. Symbolic space is a discrete space that is composed of symbols and which represents conceptual space. In order to express that symbolic space intersubjectively, humans use words and language. Since abstract parts of speech require higher-order concepts, language involving syntactically complex sentences is generally possible only for humans
Language can be understood as a way to create meaning from conceptual structures, which are in turn derived from a series of abstract parts of speech. The next three sections briefly explore three aspects of language: semantics, syntax, and sentences.
3.8.3.1 Semantics
Semantics is the study of symbolic meaning. Concepts have two kinds of semantics, one with respect to the sensory and conceptual parts that they compose, and one with respect to the conceptual wholes of which they form a part. These two kinds of semantics form the absolute and relative meaning of those concepts. Mathematically, they are analogous to the intension and extension of a set.
To learn an object as a zeroth-order concept requires emotional motivation and direct experiences with that object. When such experiences happen a sufficient number of times, a conceptual whole is formed that consists of concepts corresponding to the parts of that object, and which in turn forms a part of the larger conceptual contexts in which that object appears. Thus, the semantics of zeroth-order concepts depends on the concrete parts and wholes of the corresponding object, or its mereological context.
To learn what an object is as a higher-order concept requires knowing its abstract (symbolic) parts and wholes. For example, the meaning of the abstract concept treeφ derives from pines and maples being kinds of trees, and trees in turn being kinds of plants. Further, the meaning of the concept treeφ also derives from things it is not; for example, the digram in Figure 3.8.3a illustrates that trees are plants that are not house plants.
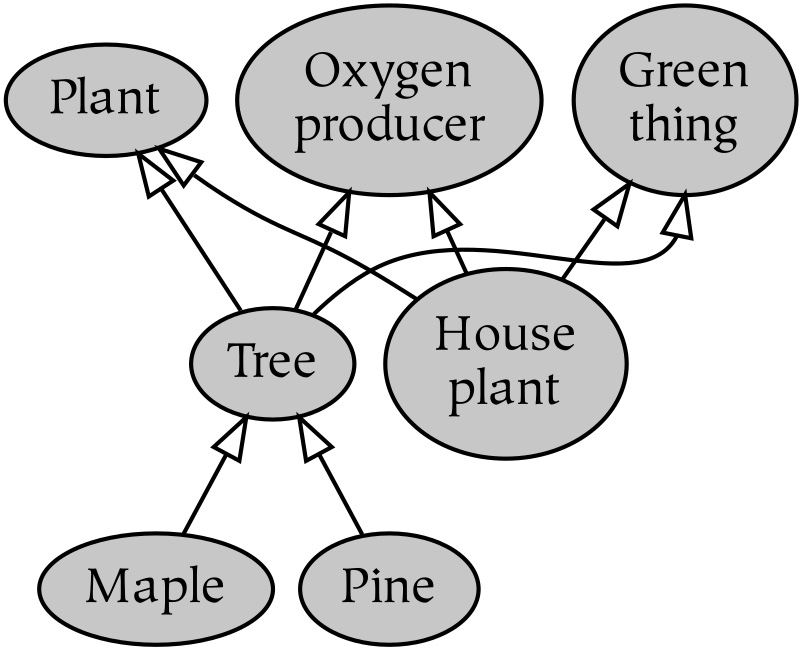
[Figure 3.8.3a: Trees, presented in an abstract
taxonomic context.]
Figure 3.8.3a may not be a typical model of the cognition of city dwellers, in that it depicts treeφ as a concept that is a composite of other higher-order concepts. For people not living in nature, treeφ is more likely a basic category that is learned without symbolically differentiating the different types of trees.
3.8.3.2 Syntax
Syntax is the study of symbolic combination. One of the most interesting features of syntax is that it allows a collection of preexisting symbols to produce a novel semantic result. As a result, while the concept iceφ is known on a concrete basis to residents of Canada, it may be known only on an abstract basis to residents of a hot country with no refrigeration (i.e., where there is no ice). In other words, residents of that hot country know iceφ only by its definition: solid, cold water. This process of using syntax to recombine known concepts is used both dynamically to understand language, and as the underlying structure to create higher-order concepts.
In order to keep the syntactic analysis fairly simple, this section focuses on the following sentence (and ignores its definite article, “the”):
The green frog croaked loudly.
Under the assumption of a binary-branching syntax with verb phrases (VP), noun phrases (NP), adjectives (ADJ), adverbs (ADV), nouns (N), and verbs (V), the syntactic production rules for this sentence may be written as:
S → VP (NP)
NP → ADJ (N)
VP → ADV (V)
These rules are sufficient to create the deep structure of the sentence, but they are not sufficiently detailed to show how various parts of speech relate to concepts. To begin, the sentence is analyzed into the following parts of speech:
greenADJ, frogN, croakedV, loudlyADV
Applying the syntactic rules given above to these parts of speech results in the syntax tree depicted in Figure 3.8.3b. Since each of these parts of speech correspond to cognitive symbols, the parsed sentence may also be represented as:
loudlyξ( croakedξ ) ( greenξ( frogξ ) )
As the sentence is constructed, the symbols of the sentence are visualized one after the other. Since the noun “frog” is the deepest part of the structure, it is the first concept to be visualized and understood.
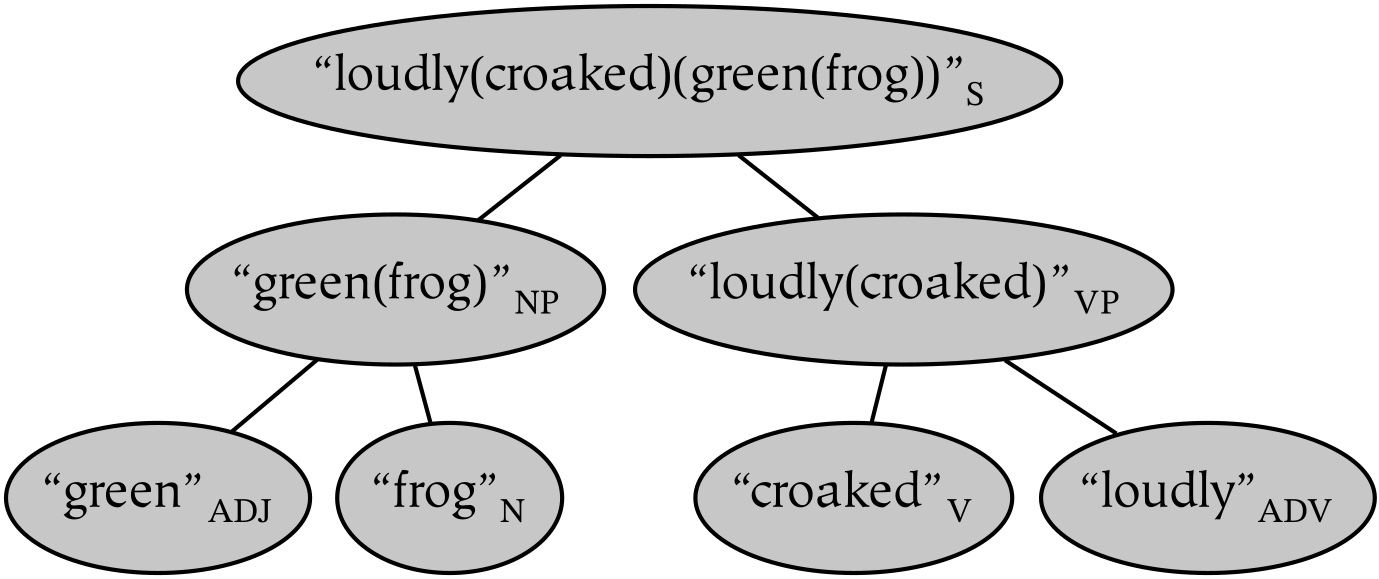
[Figure 3.8.3b: The deep structure of the phrase
“green frog croaked loudly”.]
This syntactic process can be modeled with more precision by augmenting the intuitive understanding of nouns and verbs with a more formal notion that includes their dimensionality. In particular, the recombination of abstract parts of speech such as nouns (which lack any temporal aspect) and verbs (which lack any spatial aspect) results in sentences that can be visualized in a concrete, high-dimensional space. In this example, if the dimensionality of the entire sentence is n, then the dimensionality of frog is n-x, and the dimensionality of croaking is x. Therefore, combining these concepts restores the dimensionality of the original event (n). Other parts of speech play similar syntactic roles; for example, definite articles reduce the generality of their associated count nouns.
Hypothetically, this syntactic process that restores the dimensionality of concrete experience is necessary to fully visualize abstract concepts. For example, the visualization of the abstract subject frogξ requires seeing the frog’s wide mouth and big eyes as it squats on a lily pad. The visualization of the other parts of the sentence further limits the scope of the visualization. For example, greenφ removes the possibility of the frog being brownφ, and the visualization of the verb croakξ prevents the frog from just sitting there, doing nothing.
Applying this process to the original sentence results in four acts of successive visualization:
Ψ(frogφ), Ψ(greenφ), Ψ(croakedφ), Ψ(loudlyφ)
To generalize this process, the original grammar can be extended so that the terminal nodes consist exclusively of sensation, which creates a transformation from sentence structure to the concrete sensory space in which the meaning of the sentence is visualized:
S → VP (NP)
NP → ADJ (N)
VP → ADV (V)
ADJ → Ψ (Ω (adjξ)
N → Ψ (Ω (nξ)
ADV → Ψ (Ω (advξ)
V → Ψ (Ω (vξ)
3.8.3.3 Sentences
There are fundamentally two types of sentences:
sentences about events and sentences about identity. The previous section explored the structure of a sentence about a physical event. A second kind of sentence describes language itself, and is used to define words by expressing relations at a higher epistemic level. The distinction between sentences about the world and sentences about language mirrors a fundamental dichotomy known in many contexts with different terminology: synchronic/diachronic, knowledge/news, a priori/a posteriori, synthetic/analytic, de re/de dicto, necessary/contingent, etc. Distinguishing between these two types of sentences is vital; to mistake one type of sentence for the other leads to subtle but serious confusion.
Sentences about events can be understood as formulas to dynamically construct zeroth-order concepts, while sentences about language describe higher-order relations. Sentences about language typically take the following form: word is a definition (or part is-a whole). Therefore, sentences of this kind should be interpreted as definitions that express (abstract) logical relations, rather than (concrete) contingent statements about the world:
Apples are fruits.
An apple is a fruit.
Apples are red.
An apple is a red thing.
The underlying structure of these sentences is significantly different from the structure of sentences about events. Consider the example made famous by Helen Keller, who at the age of twenty-one learned her first word: waterξ. Her understanding entailed knowing that the word waterξ meant waterφ. It is tempting to model this as an association between the auditory word waterφ and the physical experience of waterφ:
water-wordφ = water-objectφ
Although the equivalence relation does not exist in the basic model as an associative link, it can be expressed as two symbols that belong to a common whole, which establishes an equivalence class over those symbols:
whole( ref( water-wordφ ) , ref( water-objectφ ) )
The equality between these two concepts is thereby achieved by the introduction of symbols that designate each of them, and a metaconcept which creates an equivalence class that contains the abstract water-object and water-word concepts, which is graphically depicted in Figure 3.8.3c. In this way, metaconcepts equate verbal concepts with their corresponding physical concepts, thus associating word and object.
[Figure 3.8.3c: The proposed structure behind knowing
“water is water”.]
Part 4
PRACTICAL IMPLICATIONS

A human being is a part of the whole, called by us “Universe”, a part limited in time and space. He experiences himself, his thoughts and feelings as something separated from the rest—a kind of optical delusion of his consciousness. This delusion is a kind of prison for us, restricting us to our personal desires and to affection for a few persons nearest to us. Our task must be to free ourselves from this prison by widening our circle of compassion to embrace all living creatures and the whole of nature in its beauty.
Albert Einstein [Einstein & Sullivan, 1972]
Chapter 9
Concrete and Abstract

Concrete or zeroth-order concepts are wholes of sensation. Because they have the dimensionality of those sensations, they are concrete, open-dimensional events. Similarly, entire sentences that are formulas for constructing zeroth-order concepts result in concepts of the same dimensionality. In contrast, individual parts of speech represent abstract concepts and have only a portion of the dimensionality of sensory events, which is exactly what makes them universally applicable. For example, the color blue would not be able to color all the blue things if it had a particular spatial location.
As another example, take the sentence “She dances beautifully”. Most people probably accept that without a dancer of some sort, there can be no dance. Similarly, there can be no dancer without a dance; a dancer does, will do, or has done a dance. The co-occurrence of the dancer and the dance is a necessity; they constitute a concrete whole that corresponds to a complete sentence, that cannot be validly referenced using either a noun (the dancer) or a verb (to dance) exclusively. More poetically, neither a dancer nor a dance has ever existed independently of the other.
As abstract parts of speech are combined, they create concepts that are increasingly concrete. For example, the phrase “she dances” exists more concretely than its subject (“she”) or its predicate (“dancing”); the entire sentence is more real, meaningful, or concrete than its noun or verb phrases individually. The physical things that are designated by nouns are abstract concepts that lack a temporal dimension, and thus they cannot form a concrete part of a high-dimensional space (unless those nouns are implicitly or explicitly modified by verbs). Although nouns are meaningful in an abstract sense, their dimensionality is lower than that of physical space because they lack a temporal extent (nouns are abstract precisely because they generalize over the temporal dimension). Therefore, they are dimensionally incomplete, and only concrete concepts are completely meaningful as parts of a high-dimensional space.
The distinction between concrete and abstract can be understood by analogy: although the adjective “quick” has some meaning, it is easy to recognize as incomplete. It begs the questions, “Quick what? What is it that is quick?”. However, nouns such as “me” are also incomplete and should raise similar questions: “I did what?”. Further, nouns are not merely incomplete in terms of sentence structure, but in terms of corresponding to anything concrete (presumably because material solidity is regarded as sufficient for existence under the classical (Newtonian) view of space).
The implications of incorrectly reifying nouns or other parts of speech are both practical and significant. Since desire affects things as we understand them, misunderstanding the nature of things renders us less capable of achieving our desires. For example, although we will be unhappy if we like “tasting sugar” but mistakenly seek “tasting salt”, we will probably be able to learn from our mistakes in a straightforward way. On the other hand, desiring the wrong type of things is a more subtle and pernicious problem. For example, if we like the taste of sugar but end up seeking sugar as a substance, we might collect sugar far beyond the time or capacity that we have to taste and enjoy it, a mistake that is considerably more difficult to detect and correct.
Chapter 10
Identity

The relation of identity is defined under the assumption that events are unique, so by default, no two events are identical. Similarly, all sensations are different: there is always a dimension along which any two sensations differ. However, when sensation is collected into concepts, the symbols that refer to those concepts enable generalization by higher-order concepts. In virtue of that generalization, differences between individual concepts are forgotten, and they become conceptually identical. This nominal identity is not entirely subjective since it captures aspects of truth about the world, but in virtue of forgetting, it does not capture the entire truth about the world.
Because nominal identity requires the association of a symbol and an object, it is invariably approximate. This inexact relationship often manifests in non-obvious ways, such as the paradox of the heap (see [Hyde & Raffman, 2018]):
If there is a heap of sand, and grains are removed one at a time, at what point is the heap no longer a heap?
While it is clear that a heap exists at one point and does not at another, it seems odd that a single grain of sand could make the difference between a heap and a non-heap. If a single grain of sand cannot make such a big difference, then heapsφ and other concepts apply to their objects to varying degrees, which is problematic for anyone who believes that propositions about objects in the world can be fully true or false.
In virtue of generalization, the same object can be described in many different and valid ways. For example, the following large-scale and small-scale descriptions of an apple given by people in different lines of work illustrate this point:
Sociologist: “The apple is a food-stuff which the proletariat can turn into cider and feed to the masses to keep them from revolting.”
Psychologist: “The apple often stops the hunger neurons from firing, thus contributing to the cessation of the apple-gathering response.”
Biologist: “The apple is a fruit, whose sweetness has been selected by evolution to provide for the disbursement and fertilization of the tree’s dicotyledonous progeny.”
Chemist: “The apple is a complex of medium-chain, starchy hydrocarbons. It contains approximately twenty grams of fructose.”
Physicist: “The apple contains primarily carbon, hydrogen, and oxygen. It warps spacetime in virtue of its mass.”
Each statement is only a partial truth with respect to the apple object. The variety of these statements demonstrates that the characterization of an apple depends on an observer’s perspective, and that none of these statements provides an exhaustive account. Even a single observer characterizes the apple differently at different times: the observer-when-hungry categorizes the apple as something to eat, while the observer-when-fed categorizes the apple as merely a fruit. These different levels of description are not inherently exclusive of each other, but only one formulation exists at one time when they are formulated symbolically. In virtue of that, emotions and thoughts often isolate a single story from all of the possible narratives that could be told about an apple.
Chapter 11
Absolute and Relative

Imagine a town in which “… all the children are above average”. Although it is a pleasing image, such a town cannot exist; in order for someone to be above average, someone else must be below average. Nonetheless, it serves as an excellent and very explicit example of the relativity of properties.
The properties of an object, such as being average, are either relative or absolute because they are extrinsic (and inessential) or intrinsic (and essential) to that object. In other words, the relative properties of blueberries such as being something that grows on bushes, depend on the relationship of those blueberries to other things. Their absolute properties, such as high fructose content, depend on aspects of blueberry-matter and are theoretically independent of the relationship of blueberries to other things. However, the distinction between these two types of properties is not always clear; for example, the relative property “eaten by people” is related to the absolute property “fructose content”. Ultimately, it may not be possible to completely separate the absolute nature of a thing from all of its possible (relative) interactions.
If all properties are relative, however, things become meaningless. Therefore, one might conclude that only certain properties are absolute, or have a definable essence that is independent of other things. But which properties are absolute? Is the mass of a tree absolute, since it does not depend on the mass of non-tree things? However, the mass of a tree is defined in kilograms, and kilograms are in turn defined relative to the mass of a certain volume of water at sea level. This chain of reasoning seems to indicate that even abstract properties of an object such as its mass are relative to external objects.
The implicit resolution to this issue is that things are relative to one another in virtue of their wholes, while they are absolute in virtue of their parts. Even the expression of absolute properties such as mass is relative, however, because expression is necessarily symbolic. On the other hand, our sensory experience of heaviness is inexpressibly unique and experienced absolutely (or in a bottom-up sense).
The philosophy of reductionism makes this identification between parts and the absolute explicit by claiming that relative descriptions are exclusively external (or whole-based) and that absolute descriptions are exclusively internal (or part-based). Reductionism also claims that a thing is fully known when one knows its parts. For example, to understand people and their behavior, one must study psychology. To understand how psychology works, one must study physiology and the mechanism of the brain. To understand how physiology works, one must study biology and the mechanism of the neurons within the brain. To understand how biology works, one must study chemistry or physics and the mechanism of the molecules and quarks that make up the neurons, et cetera.
While there is no question that reductionistic analysis results in a very detailed explanation, it does not necessarily entail an increase in explanatory power. In other words, although a description that uses small parts is more detailed, it may be unnecessarily complicated. Similarly, the operation of the whole is not caused by the operation of parts any more than the operation of the parts is caused by the operation of the whole; causation requires that causes temporally precede their effects, which is not the case for spatial wholes and their parts.
Therefore, appeal to microscopic objects is not always necessary (or even beneficial), since movement at a macroscopic level can often be fully described in terms of macroscopic objects. For example, the neural or neuroanatomical description of a person is substantially less useful to most people than a physiological description, since we can more easily make physiological changes like getting exercise than direct changes to our chemical composition. Further, although the microscopic level does offer a more detailed analysis, it substitutes rational and relative thought for intuitive and absolute knowing, which is not always beneficial.
Chapter 12
Negation

Sensation derives meaning by referring to objects; that meaning is absolute in so far as it is not relative to other sensations. Similarly, the bottom-up aspect of concepts is defined by the sensations of which those concepts are composed. The top-down aspect of concepts, on the other hand, is defined relative to other concepts; concepts without sensory input rise to the foreground only when others recede to the background. As a result, concepts formed by a bottom-up process do not have a negation, whereas concepts formed by a top-down process do have a negation.
In other words, what is absolute is regarded as a whole; it is not a part, and therefore it is not relative to some larger thing. Because the absolute is whole and does not have a complement, therefore it has no negation. Similarly, the reason that conceptual parts do have a negation is that they are defined top-down, relative to a larger conceptual space. Specifically, they have complements with respect to the concepts that are their wholes, and those complements form their negations (i.e., with respect to those wholes). Thus, concepts in and of themselves are neither strictly relative or absolute: they are absolute in virtue of the sensation of which they are a whole, and they are relative in virtue of the larger concepts of which they are a part.
At the endpoints of the sensory–conceptual continuum are purely analytical entities that have no absolute aspect (or negative entities), and unanalyzed things that have no relative aspect (or positive entities). These two types of entities offer an excellent example of the separability of the relative and the absolute.
Negative entities such as a holeφ are defined in relation or relative to other concepts, but they have no (absolute) sensory content of their own. Therefore, holes are known only in relation to a larger context; for example, a hole in the ground is known only in virtue of being surrounded by earth. At the other end of the spectrum are positive entities, which are purely sensory or absolute, and therefore do not have a negation. For example, “the negation of a tree” is not meaningful at a sensory level: it does not entail any other sensation, and it is not clear what the not-tree looks like. In other words, there is no such thing as a sensory opposite, since all sensation is positive appearance.
Most entities have both relative and absolute aspects; therefore, the relative aspect of an entity has a negation while the absolute aspect does not. From a subjective point of view, anything is absolute if it is left unanalyzed and experienced purely bottom-up, and anything is relative if it is analyzed and experienced in virtue of top-down influence. More simply, every entity is absolute as a whole and relative as a part.
Chapter 13
Intuition

Intuition is not the same as rational thought, but it is not irrational either; it is multi-rational, capable of understanding multiple concepts at the same time. Therefore, intuition is able both to use concepts and to transcend some of the limitations of rational thought. Although intuition is sometimes characterized as nonconceptual since it is non-symbolic, it is more properly characterized as multi-conceptual since it utilizes the content of multiple concepts (just as a picture is worth a thousand words). Because conceptualization is often relatively course, intuition is often associated with sensation rather than conceptualization, although intuition could not represent any mereological relations if it had no part/whole structure.
As opposed to intuition, rational thought is constructed of statements, where each statement has a syntactic structure that corresponds to a single conceptual hierarchy. Although there are exceptions such as puns that may correspond to two or more conceptual hierarchies, most statements are structurally unambiguous. Intuition, by contrast, uses an extremely limited syntax (i.e., mereological parthood without symbols), but it can use all words at once. Hence, intuition is a structure which corresponds to a dense mass of mereological and referential relations between multiple, overlapping concepts. Therefore, for the intuitive mind, everything is related to everything else.
Historically, the division between intuition and rational thought has manifested in many ways. Evans and Stanovich, reporting on this division in the context of Dual Process Theory, sum up this situation as follows:
The distinction between two kinds of thinking, one fast and intuitive, the other slow and deliberative, is both ancient in origin and widespread in philosophical and psychological writing. Such a distinction has been made by many authors in many fields, often in ignorance of the related writing of others.
Jonathan Evans, [Evans & Stanovich, 2013].
In the language of Dual Process Theory, intuition is a product of System 1 and rational thought is a product of System 2. In terms of the basic model, System 1 consists of sensation and zeroth-order concepts, while System 2 consists of symbols and higher-order concepts. As System 1 is multi-conceptual and operates in parallel, it cannot be symbolic. Conversely, symbolic processing must happen serially, so System 2 is only capable of conceptualizing a single concept at a time.
The informal argument that System 2 necessarily operates serially is based on inhibition. In particular, the argument from inhibition that symbols must operate serially is that symbols are defined in terms of both the presence and absence of other concepts. In other words, symbols are defined in terms of what they are not, in addition to what they are. Further, the strength of top-down activation must outweigh bottom-up activation, otherwise it would be impossible to conceptualize things that are not present. In other words, symbols must be capable of representing and reactivating what they represent in the context of unrelated bottom-up activation, and the way to do that is by strongly inhibiting the negation of that concept.
Type 2 thinking, by inhibiting all irrelevant activation and forgetting numerous other conceptual relations, emphasizes a particular meaning. Therefore, while Type 2 thought is capable of knowing the parts and wholes of a concept, it causes the isolation of that idea by inhibiting its complement.
In contrast, Type 1 knowing does not isolate things in this way; it does not take things out of context or understand things solely in terms of their parts, but rather knows things in relation to everything else; no relations are completely inhibited. Type 1 knowing is therefore not only capable of knowing many concepts about something, but it is capable of knowing those concepts simultaneously (i.e., since concepts do not inherently interfere with one another). Additionally, because conceptual operations are performed in parallel, intuition is extremely fast. In fact, the intuitive mind knows so much so quickly that it cannot be adequately described in language. As a result, intuition is often expressed metaphorically, since relating intuitions to one another bypasses the need to define them explicitly.
Chapter 14
Animal Cognition

The differences between animal and human cognition are remarkable given the relative lack of neuroanatomical and genealogical differences. Unfortunately, understanding these differences is inherently difficult for at least two reasons. First, animals are reticent to talk in public, so it is difficult to hear about their experience first-hand. Second, although humans talk voluminously, it is difficult to isolate the aspects of cognition that are uniquely human, other than vague indications of “consciousness” or “language”. Therefore, in order to contextualize the theory of animal cognition, it is helpful to have a more general theory about where all things fit; inanimate objects and plants should have a place in the theory as well. Not surprisingly, these other forms of life are categorized by the basic model according to their degree of referentiality, or their epistemic level.
At the level of epistemic ground are inanimate objects, which lack the ability to sense or to act. For example, rocks do not sense or react to a neighboring brook in virtue of any internal mechanism or references.
A tree, on the other hand, senses things; for example, it senses the sun, and reacts to that sensation by growing toward it. Thus, the tree may be said to be conscious of itself in the same way as a rock, but it also has awareness of its referents, such as the sun. In terms of the basic model of cognition, plants sense, but they do not conceptualize because they do note have a mechanism by which they can form unitizations. For the same reason, animals with nervous systems can conceptualize things.
To summarize, non-referential (or reflexive) consciousness is something that all things have, referential awareness is something that only plants and animals have, and conceptual awareness is something that only animals have. The further differentiation between animal and human cognition is primarily attributed to the human capacity for words and symbols. The smallest change to the basic model of cognition that is capable of producing this difference is the removal of interpretation (Ω). Removing interpretation (and possibly also symbolization, as shown in Figure 4.14) from the basic model results in a model of animal cognition that allows sensation and concrete concepts, but not symbols or higher-order concepts. This model predicts that animals may sense, visualize, and to some extent conceptualize in the same manner as humans, but they cannot engage in symbolic thought, and as a result, animal cognition is necessarily concrete.

[Figure 4.14: Animal cognition, which lacks
interpretation (Ω) and symbolization (Ξ).]
As symbols enable abstraction, animals can form concrete unitizations but not abstract generalizations, and therefore they cannot think abstract thoughts. Further, they do not experience objects as nominally identical, since identity requires higher-order concepts. This difference in cognitive structure therefore entails differences in what is a priori for animals as opposed to humans.
Behaviorally, the lack of interpretation can be understood in relation to the distinction in linguistics between signs and symbols: animals understand words as signs, but not as symbols. Signs are concrete, and often indicate that something else is impending (e.g., sitφ, stayφ, “play dead”φ, etc.); they are always embedded in a causal context because they form a single spatiotemporal unit. On the other hand, symbols represent something and can be used to directly activate the concept that they represent.
Symbols enable a dramatic evolutionary advance because they enable the easy formation of recursively constructed concepts, and therefore the creation of linguistic structures of arbitrary complexity (see [Chomsky, 1995]). Linguistically, therefore, while animals may generate and understand certain verbal behaviors, the underlying deep structure of those utterances is significantly different from human syntax. Since the concepts of animals are always zeroth-order, characterizing how animal communication is different from human language requires identifying what parts of speech correspond to zeroth-order concepts. The basic model of cognition indicates that animal cognition is formed of entire sentences. Thus, what animals lack is the ability to create and comprehend abstract parts of speech such as nouns and verbs. In other words, since animals cannot form concepts corresponding to abstract parts of speech, their sentences are limited to be one word long and to represent entire events.
Expressed slightly differently, the theory of animal cognition proposed here is that animals comprehend the world as composed of events, rather than spatial objects and temporal processes. On a syntactic level, animals comprehend entire sentences, but not parts of speech such as nouns and verbs. This is a direct result of not comprehending space or time as independent entities on a cognitive level (see [Rogers, 1995]). In other words, the concepts of time and space as independent entities in human cognition are only possible in virtue of the abstract generalizations that are enabled by symbolization. Studies of animal behavior reinforce this finding by demonstrating that behavior is unitized, or that learning to perform a given spatial task entails learning both a time and rate at which that task is performed [Gallistel, 1980].
Chapter 15
Bottom‑Up and Top‑Down

Bottom-up and top-down processes in cognition imply a vertical dimension that can be measured using the notion of epistemic level. Sensory parts are responsible for bottom-up activation, and symbolic wholes are responsible for top-down inhibition.
The role of bottom-up processes in cognition is fairly straightforward: concepts are recognized in virtue of sensory input, especially if those concepts are emotionally significant. The role of top-down processes in both what is perceived and how it is perceived is a bit more complicated, and often underestimated. As a result of that top-down influence, our perception operates with significant distortion and even creation, an interference that often goes unnoticed. Perhaps this is inevitable, since the cognitive detection of what is not perceived requires extraordinary investigation.
A simple example of bottom-up and top-down effects in the visual system is the phenomenon of blind spots, which are areas of the visual field for which there is no sense data. Figure 4.15 can be used to see your blind spots—or better yet, can be used to not see them. First, close one eye and look at the dot on the side opposite of your open eye, and move the figure slowly closer and farther from your face. At a certain distance, the dot that you are not directly looking at disappears from your visual field.

[Figure 4.15: Blind spots.]
The blind spot in each eye is created by a small patch of missing retina, medial to the point directly behind the pupil where the optic nerve connects to the eyeball. As a result, there is no sensation from the corresponding part of the visual field. Blind spots demonstrate both that there are areas of reality that we expect to sense that we do not, and that we are not aware of this lack of sensation. Further, blind spots are not only sensory holes that we do not see and of which we have no awareness, but are areas of illusion. To more clearly demonstrate this fact, if you pass a pen in front of the blind spot that you identified during the previous experiment, you will not see a hole in the pen; rather, the missing part of the pen is filled in by your mind. Therefore, blind spots are characterized by both bottom-up, sensory deficits and top-down illusions.
These missing bits of sensation and areas of hallucination clearly demonstrate that the world as we perceive it is not the world as it is; our sensations are filtered to make them comprehensible. Although this is not necessarily a bad thing, the lack of awareness of this alteration is problematic. As another example of top-down influence, consider the following question:
What part of your mind are are you using?
Most people who read this sentence see it as identical to the following sentence: “What part of your mind are you using?”. As the first sentence is not syntactically well-formed, they are not identical unless the first sentence is mentally altered to conform to our expectations and understanding. This type of correction is often beneficial; for example, it automatically corrects the syntax of ill-formed sentences such as the one above and causes false positive percepts of dangerous creatures. However, it is harmful if it creates a world that always conforms to our understanding and in which we recognize only familiar things, since living in such a world would not enable our understanding to increase.
Dreaming and hallucinations are even more extreme examples of playing a creative role in what we sense. If the dream of a tree can arise without a tree, then no external cause at all is necessary for perception. Although this does not invalidate the more common case in which the tree-sensation arises in conjunction with a tree-object, it does make the relationship between the two more tenuous. As a rather extreme example that a number of philosophers and novelists have used to illustrate this issue, there is no way to tell if we are dreaming or not when sensations do not depend on the presence of objects.
As these examples illustrate, top-down and bottom-up processing offer both an opportunity to balance and a risk of imbalance. That balance is particularly necessary in contexts such as learning a new activity, since top-down cognition is essential for many activities, but it also hinders expert performance. In such cases, therefore, it is beneficial to be able both to think about things and to not think about things.
Chapter 16
Attention

Attention is an operation that restricts the domain of perception from an omnipresent awareness to a localized awareness. This selectivity causes an individual to be relatively blind to things outside of the aperture of attention, a phenomenon called inattentional blindness. This process is analogous to putting blinders on a horse: although it causes the horse to be less distracted, the horse also perceives less. On a positive note, however, the decreased aperture of awareness allows the horse to more fully analyze what is perceived.
Attention is governed by both bottom-up and top-down processing, which gives rise to two types of attention called stimulus-driven and goal-directed attention, respectively. In terms of the basic model, attention is determined bottom-up by conceptualization and top-down by visualization. Bottom-up sensory activation is prone to grab attention if it is particularly intense, unexpected, or emotionally valent. Top-down conceptual inhibition guides attention to what is relevant by inhibiting conceptual wholes outside the scope of attention. As a side effect, top-down attention is limited to concepts that already exist.
Attention has a similar relationship to both concepts and emotions. Just as concepts guide attention and the subsequent scope of attention limits subsequent concepts, attention is also guided by emotion and that scope limits subsequent emotions. In both cases, we are conceptually and emotionally reactive primarily to the content of awareness, and it is attention which in turn governs the aperture of that awareness.
Attention, in addition to being viewed in an impersonal causal chain, is a skill that we are practicing all the time, regardless of whether we are conscious of doing so or not. Training in attention explicitly often entails exercising both its mereological and referential aspects.
Mereological training in attention consists of exercises such as directing awareness to areas of space of of which one is infrequently aware as in body scan meditations, or sustained awareness of a particular location as in meditation on an object. This process happens routinely as a side effect of various activities such as physical exercise or piano practice.
Referential training in attention explicitly directs awareness to different epistemic levels. For example, one may direct awareness exclusively to the occurrence of thoughts or to the referential content of those thoughts, where the latter awareness is of a higher referential level than the former. This type of training figures prominently in various forms of contemplative practice and meditation. In terms of the basic model of cognition, meditation that enables one to remain at the epistemic level of concrete concepts develops one’s intuitive ability.
Chapter 17
Emotions

Despite the profound importance of emotions, much less is known about them than is known about thoughts. Perhaps this is due to their relative complexity, the tendency of cultures to preserve teachings from higher epistemic levels better than teachings from lower epistemic levels, or to the fact that emotions are not well-suited to being described symbolically.
The cultural dissociation of identity from emotionality is so prevalent that it is encoded in numerous social and legal systems. For example, there is no legal responsibility for actions that are committed “in the height of passion”. On the other hand, crimes committed when “thinking too much” are considered premeditated, which increases the amount of responsibility. But if we are responsible for what we think, why are we not responsible for what we feel? One possible answer is that identification with thoughts rather than emotions is an attempt to define ourselves as what we have control over. However, the claim that we have control over what we think about is dubious, because most of us cannot even control whether we are thinking or not. In other words, if one does not have sufficient control over one’s mind to start and stop thinking, then how could it be possible to have the fine degree of control required to choose the content of thought?
Historically, the reluctance to scientifically examine emotions is probably related to their antagonism with rational thought. However, the depiction of cognition as consisting of exclusively bottom-up emotions and top-down symbols is an overly simplistic characterization, since emotions play a large role in reasoning, just as emotions have their reasons. Although strong emotions can certainly encourage illogical conclusions, we think certain thoughts as opposed to others precisely because of their emotional valence and their conceptual context.
The role of emotions is particularly complex because they can become attached to symbols, or to references themselves instead of their referents. For example, consider the profound differences between liking the sensation of “peanut butter”ψ, liking the concept of “peanut butter”φ, and liking the symbols “peanut butter”ξ.
Liking the sensation of peanut butter means that you enjoy the taste of peanut butter or the mouth feel of dollops smeared on an apple or carrot.
Liking the concept of peanut butter means that you like the concept rather than the experience. Perhaps peanut butter is good because it is buttery, vegan, and not as expensive as cashew butter.
Liking the symbol of peanut butter means that you like the actual symbols (i.e., the words “peanut butter”). Although it may seem odd to like symbols, they may become significant triggers if they are conditioned by their association with desirable and undesirable objects.
The emotional valence of concepts becomes the emotional valence of objects in a process called cognitive fusion. Cognitive fusion is an operational hazard of the way cognition works because it causes emotional confusion between concepts and objects (i.e., as a result of the concept inheriting the emotional significance of its referential content). The occurrence of the concept may additionally cause physiological responses that are inherently rewarding beyond the actual experience, such as activation of the parasympathetic rest/digest mechanism. As a result, thinking has the potential to condition concepts even further by creating a feedback loop that does not involve the presence of the object.
At least in some instances, emotional attachment to symbols rather than their intended objects seems to be a mistaken form of cognition. It can certainly be disadvantageous if it biases which thoughts do and do not occur in virtue of how much we like the thoughts themselves as opposed to the content of those thoughts. Evolutionarily, it may be simply a side effect of emotions conditioning sensory space, and symbols making use of that same sensory space to represent concepts. On a practical level, even if emotional attachment to symbols is not viewed as an inherently negative thing, disentangling the attachment to symbols from the attachment to the concrete phenomena that those symbols represent is a formidable task.
Regardless of one’s views on the proper role of emotions with respect to epistemic level, two things are clear and worthy of reflection. One is that emotional attachment to higher epistemic levels in general causes strong top-down influence, which can be disadvantageous. Another is that lack of equanimity with respect to symbols at any given epistemic level causes bias in that top-down influence. The combination of these two, therefore, is especially problematic.
Chapter 18
Beauty

Where do we experience beauty? Do we experience it in the space of our bodies, or in a space that is coextensive with the world that we perceive? Folk wisdom holds that beauty is in the eye of the beholder, and psychologists hold that the positive feelings associated with beauty originate in the dopaminergic neurons of the limbic system. Similarly, the world points to us and tells us that our sense of beauty is subjective, or in our heads. However, we experience beauty in the objects of the world, and tell the world that we experience beauty out there. If beauty can only be present in a single location, then which location do we choose?
This incongruity with respect to the location of beauty is typically taken as evidence for indirect realism, rather than support for the argument that consciousness is extended. In other words, there are objective aspects of sensation that are due to the world and subjective aspects that are due to us, and beauty is regarded as one of the latter. However, these internal references to an external world provide two possible points of view with respect to the location of beauty.
From the objective or material perspective, references are not treated as referential, so the experience of those references is located within the body. This is the predominant view within science as a whole: it views human bodies as parts of larger wholes and minds as collections of references to that larger whole that are contained within the body. Awareness of the external world is enabled by referentially bringing the external world within the brain or body, which is often assumed to be the location of consciousness.
From the subjective or mental perspective, on the other hand, experience is constituted by the content of those references, and as a result, the experience of beauty is located throughout the world. In other words, since we perceive the referential content instead of the references themselves, beauty is located in beautiful objects rather than in the head. Even if the objects are beautiful because of our previous experience with them, then beauty is a relation in the world which we perceive or not in virtue of having that experience.
If both truths are compatible and valid from different points of view, then it would be a mistake to let the concepts of others interfere with our experience of beauty or vice-versa. Therefore, to cease feeling that external objects are beautiful because we are told that nothing is objectively beautiful would be a terrible mistake. If we find roses beautiful and someone else does not, all that is entailed is that we have the concepts that allow us to see their beauty because of our experiential history, while others do not.
The question about where beauty resides can be seen as part of a larger question about whether objective space should be seen as the intersection of all subjective perspectives or as the union of all subjective perspectives. For example, it is common to see pine trees as green, and it is less common to see them as beautiful. This is typically taken as evidence that greenness is an objective aspect of pine trees, while their beautifulness is a subjective aspect. Although there is no question that beauty is harder to define, the further assumption that beauty is purely subjective while green is purely objective amounts to an intersectional thesis. According to this thesis, the physical universe is itself entirely devoid of beauty; beauty is merely the subjective judgment of an observer.
The unional thesis entails that objective reality actually contains all subjective truths. For example, a pine tree is both beautiful and ugly from different yet valid points of view. According to this thesis, each of us knows aspects of a larger, multifaceted truth in which things in the world are actually beautiful and ugly from different perspectives. In other words, beauty and ugliness are relations between our perceptions and our prior experience that also exist in the world.
In slightly different terminology, the fact that the objective point of view must be valid for all observers removes beauty and other properties from objective space, because the translation of what is beautiful from one perspective to another is considerably more difficult than moving from one spatial location to another. It is worth considering, however, that just as standing in a different location brings some of the subjective observations of different observers into accord with one another, knowing each other’s prior experience with the world may bring into accord subjective judgments such as what is beautiful.
Chapter 19
Kindness

To understand intersubjective kindness, consider the following analogy:
The right hand looks after the left hand without thinking in a discriminatory way, “I am the right hand. I am taking care of the left hand.”
Thich Nhat Hanh [Hanh, 1998].
This analogy illustrates that kindness to oneself does not require justification; it is completely natural. To better understand kindness, it is useful to categorize behavior along two dimensions: self/other and dual/nondual.
Kindness entails that the self/other distinction is commutative. For example, if you have a sense of joy when someone else is pulled over for speeding instead of you, your cognition is not commutative with respect to the division between self and other. This commutativity is captured by various formulations of the golden rule, a rule that holds we should be equally kind to ourself and others. If kindness is not commutative, then it is either selfish, in virtue of which you make yourself happy at the expense of others, or it is otherish, in virtue of which you make others happy at your own expense.
Kindness can also be understood with respect to dualistic and nondualistic cognition, where duality is seen as the result of using top-down symbolic processing in cognition. Selfishness and otherishness are both inherently dualistic, since it is not possible to consistently and intentionally benefit oneself or another without reifying the duality between oneself and another; therefore, nondualistic selfishness and nondualistic otherishness do not exist (i.e., if one acts without conceptual duality, one may be kind or unkind, but not exclusively with respect to self or other).
Acting with kindness presents different obstacles depending on the presence or absence of duality. The potential hazard of dualistic selflessness is that unconditional love is necessary to unite the self and other, since they exist at a conceptual distance from one another (i.e., dualistically). If that love remains conditional, conceptual dualism is prone to tip the emotional scales in one direction or the other. On the other hand, the potential hazard of nondualistic selflessness is that if the nondualistic stance is a result of ignorance or lack of conceptual differentiation, then attempts to benefit self or other will not be reliably successful.
Because nonduality is difficult to talk about without reinforcing dualism, nondual traditions often express either that everything is self or that there is no self whatsoever. In both cases, the subject/object duality disappears. For example, if we identify with our body, we might enjoy eating savory foods or feel sad when we hurt. However, if we identify with our family or some other larger whole, then it doesn’t matter if we personally win a race or have some good thing happen to us, as long as the person who does is a part of our larger group; this is just what it means to identify as a part (of a group), rather than as a whole (of ones bodily parts).
As this discussion indicates, kindness depends crucially on the location of the self/other conceptual boundary. A more nuanced solution might preserve intellectual duality while allowing emotional nonduality; in other words, one should care for both self and other, but not confuse self and other by failing to intellectually distinguish between them. This is difficult to achieve in practice, since a person may have various habitual tendencies of which they are not conscious. For example, one might take too much credit for their own thought, rather than acknowledging the causal role in one’s thought of the entities that are perceived or referenced. This situation is similar to a prideful mirror that imagines it is entirely responsible for moving its reflections about, when in fact its (mental) references are causally moved largely by the movement of the reflected entities themselves.
Chapter 20
Overlap

The parts of the world are overlapping and interdependent. Therefore, although the use of mereological analysis to draw boundaries between things is useful, the associated tendency to consistently rely on a single partition of reality into concepts is problematic.
Unfortunately, the cognitive tendency to view the world as consisting of some number of non-overlapping parts is difficult to avoid. A particularly pernicious example of the lack of overlap is exemplified by what Daniel Dennett calls the Cartesian theatre, which exists in various explicit and implicit forms (see [Dennett, 1991]). It arises from the view that the brain contains mental representations of the world and that consciousness is the spatially-withdrawn witness of these representations, rather than being something that is unified with them. Unfortunately, theories of consciousness that posit an internal witness often involve an infinite regress, since any witness would have to form an internal witness of their own to understand the external representations that they witness. More recent philosophical theories hold that consciousness is a kind of field that exists within the brain and encompasses (and has direct awareness of) its representations. But if consciousness occurs in an extended region, why is it necessarily restricted to occurring within the brain? After all, it has not been observed there any more often than it has been observed in the world.
In general, the lack of overlap between concepts is an operational hazard of higher-order cognition; our rational minds necessarily traffic in mutual exclusivity as a result of using higher-order concepts. Although the creation of higher-order concepts out of existing concepts is more efficient than building new concepts out of sensation directly, higher-order concepts are hierarchical, and hierarchy does not represent multiple overlapping concepts very well. Therefore, it is important to balance the convenience of higher-order concepts with the precision of zeroth-order concepts. In light of this cognitive bias against the formation of overlapping concepts, it is necessary to be diligent to recognize such overlap in the world. As a particularly interesting case of overlap, consider the mereological and referential overlap of two people.
[Figure 4.20a: Mereological overlap of two people.]
Mereological overlap of two people is shown in Figure 4.20a. This kind of overlap is unusual, because it entails sharing body parts (although it happens in cases of conjoined twins and (arguably) during pregnancy). Referential overlap of individuals occurs if the subjective space of one individual overlaps the subjective space of another. This is shown in Figure 4.20b, where the numeric subscript denotes a given individual.

[Figure 4.20b: Referential overlap of two people.]
Although overlap of bare sensation is trivial because it occurs whenever two individuals see the same thing, the overlap of subjective spaces in general requires that the two conceptual spaces are the same. Thus, it is less clear to what degree the subjective spaces of two individuals are identical. For example, when you perceive a pine cone, and I perceive the same pine cone (from the same physical perspective), do we perceive the same thing? In other words, assuming an identical object, are the appearances of that object identical to each of us?
Because subjective experience cannot be verified by anyone other than the subject, perhaps there is no way to be certain. We might communicate with one another to arrive at an answer, although using communication to verify identity creates further difficulties, since we may use the same words but mean two different things. If we believe that mentality and physicality are tightly coupled, we may use the principle of Occam’s razor to argue that two individuals with the same sensory organs and concepts have identical perceptions in response to the same object. In that case, the subjective spaces of two individuals literally overlap because they perceive the same thing, whether in virtue of extended consciousness or internal representation. In more detail, two minds sensing the same object have similar perceptions up to the epistemic level at which a significant difference occurs, whether that difference occurs in virtue of different sense organs or subsequent conceptualization. In this way, referential overlap establishes a basis in virtue of which two minds are identical, even if they do not mereologically overlap.
Chapter 21
Self and Other

One of the most important conceptual dichotomies, and perhaps the first concept that we form as children, creates self and other. So how exactly is the concept of self defined? The anthropologist Margaret Mead wrote that “self-consciousness is constituted by adopting the perspective of the other toward oneself.” [Zahavi, 2008]. This entails that the self is a higher-order concept; it entails not just sensing oneself, but knowing oneself on a relative level as an abstract and enduring object. One’s self-identity is often an emotionally laden subject. Therefore, from an emotional point of view, the question “who are you?” is often related to the question “what is the largest whole of yourself that you strongly care about?”. In modern times, self is typically defined as a whole consisting of bodily parts. In older times, the self more often existed only as a part of a tribe. In both cases, however, the differentiation between self and other relies on both mereological distinctions (such as body/world) and referential distinctions (such as subjective/objective). Therefore, the rest of this section explores the notion of self in virtue of how it exists both mereologically and referentially.
A mereological theory of self is defined in terms of wholes and parts, and determines characteristics such as the spatial location and size of the self. Several options for the boundary of a mereological self are shown in Figure 4.21a. The location of the self is most often coextensive with the body or a specific part of the body, such as the heart or brain. Mereological theories of self tend to be materialistic, and therefore emphasize connections between objects in physical (or mereological) relation to one another. Mereological theories also tend to deny consciousness, although in modern culture, consciousness and the subjective perspective are more often ignored than explicitly denied.
Of the possible locations of material self-identification, identification with the body is particularly intuitive because it is the largest object that affords relatively consistent sensations while also being contiguous within the field of experience. Contiguity is especially important because it facilitates concept formation; although the body is composed of many parts and is in turn a part of many larger wholes, it is typically viewed as a single mereological whole. As linguistic evidence for this fact, a synonym for person is individual, which literally means “unable to be divided”. The importance of the bodily self in our daily lives and routines is cemented by its use as the legal definition of a person. For example, taking care of the self is a legal mandate in the modern world, while taking care of others is optional (except in the case of one’s adolescent children).
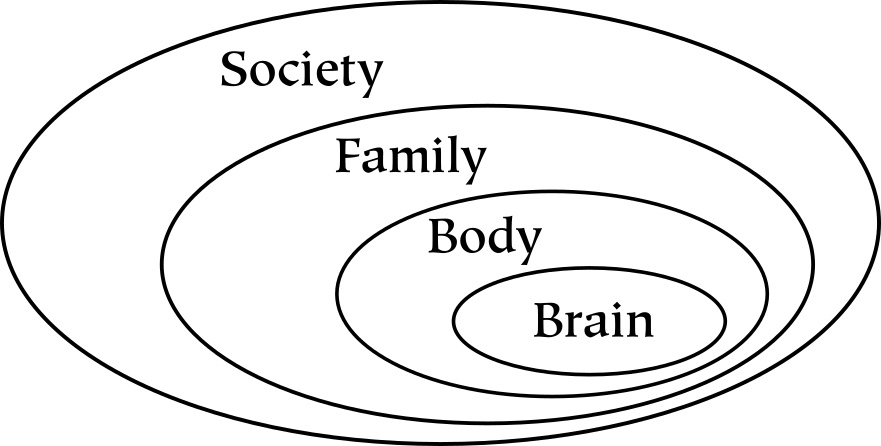
[Figure 4.21a: Analysis of the self in terms of parts and wholes.]
A referential theory of self, on the other hand, is defined in terms of references and referents, and is more closely linked with idealistic philosophies. For example, to identify only at the epistemic level of referents (as opposed to references) leads to identification with what is experienced, rather than with who does the experiencing. Although it might seem strange to identify with the experiences themselves rather than the experiencer, the opposite extreme of exclusive identification with the experiencer is perhaps even more paradoxical, since an experiencer remains unknown unless it is identical with the experience of that experiencer—and on most accounts, it is not. In other words, if you identify as the witness rather than the witnessed, then you do not know yourself from the non-referential point of view, but only at an epistemic distance (i.e., just as you would know someone else).
Referential theories of self such as idealism remove this subject/object dilemma by positing that there is no epistemic ground, or no material level of being which consists of ultimate referents. As a result of encouraging identification with perceptual content itself rather than the perceiver, this view downplays the distinction between the perception of our bodies and the perception of the world, since the location of the references is less relevant.
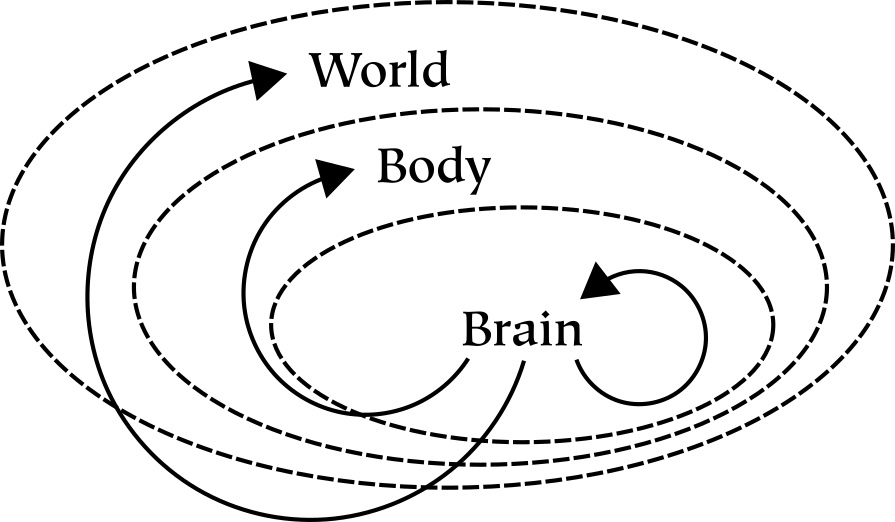
[Figure 4.21b: Analysis of the self in terms of references.]
Referential identification is illustrated in Figure 4.21b, which depicts a brain as a collection of references to both itself and to a body and world outside of itself. To identify with the references themselves implies a materialistic view and identification with the brain. To identify with the referents of those references implies identification with the referential content of the mind: the brain, body, and world as experienced.
Another way to characterize self-identification utilizes both a mereological self and an epistemic level at which one relates to that self. For example, one might identify with the bodily self, yet relate to that body in various different ways. This is illustrated in Figure 4.21c, which shows three referential spaces from which one may relate to the bodily self: objective, sensory, and conceptual. The self is viewed within each of these spaces differently, as either an object (body), a sensation (bodyψ), or a concept (bodyφ), respectively. In other words, even though one identifies with the physical body in all three cases, there is a profound difference in how one acts, feels, or thinks with respect to that body.
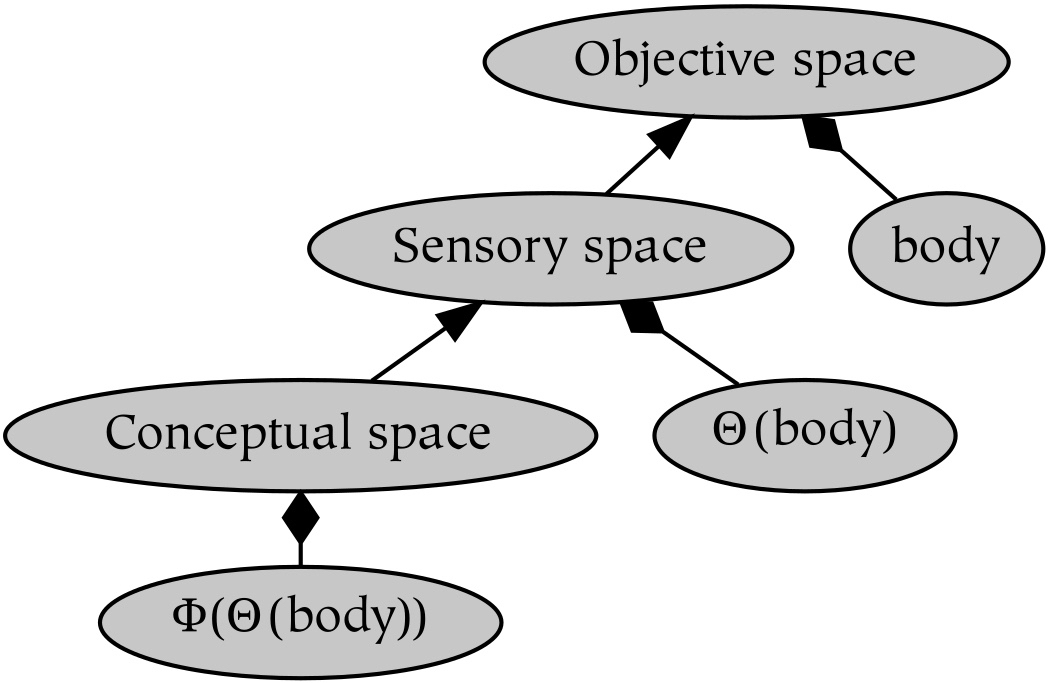
[Figure 4.21c: Depiction of bodily (physical) self,
sensation of that body, and the concept of that sensation. ]
Chapter 22
Stratified Self

To some extent, any attempt to define a self may be criticized as trying to identify a complex, concrete entity within a simple, abstract context. For example, the question “Who am I?” uses an abstract pronoun “I”, with which we are intended to identify in order to formulate an answer. However, this identification is problematic if it is incorrect to identify with anything abstract in the first place. Refusing to engage with the question is not a good solution, however, since our actions depend on our understanding, even if that understanding is implicit. Therefore, this section explores a stratified self-concept, and attempts to disentangle where we are (mereologically) from what we are (referentially).
Boundaries
Although there are many different forms of conceptual dualism, the primary form of dualism is that between self and other. The location of this self/other boundary is contentious because it is not well-defined from exclusively a mereological or referential point of view.
From the mereological point of view, the body/world boundary does not distinguish self and other as entirely different kinds of things. There are no boundaries from the perspective of an outside observer looking inwards; psychology has been exploring the brain for a long time expecting to find the location of consciousness, but it has not found any boundary where one crosses from the material world into the mental world.
From the referential point of view, the body/mind boundary does not distinguish self and other as entirely different kinds of things, either. We cannot experience the world except as it presents to us through our bodies, so in some sense we experience the world as our body. Further, a number of psychological experiments show that mind knows body at a referential distance, in the same way that it knows the world. For example, the stories that we tell about the reasons for our own actions are very often wrong, which indicates that we learn and theorize about ourselves and our motivations in much the same way that we learn and theorize about the rest of world.
Since the self/other boundary is not completely and precisely defined either mereologically or referentially, understanding it requires a more sophisticate analysis.
An Interaction
The mereological and referential perspectives of the self/other dichotomy are illustrated in Table 4.22a, where the mereological body/world distinction creates the columns and the referential body/mind distinction creates the rows.
[Table 4.22a: The self/other dichotomy viewed from mereological (body/world) and
referential (body/mind) perspectives.]

From the objective or physical perspective, there is no access to referential content (i.e., selfb and selfc are known only from the subjective perspective). Therefore, as everything is known non-referentially and materially, the distinction between the body and mind of other individuals collapses (i.e., the table becomes one row). As a result, an individual’s self is identical to the mereological body from the objective perspective, or selfa and selfb taken as an undifferentiated first column.
From the subjective or mental perspective, referents as material things are initially unknown, and the world is contained within the mind. In other words, there is no other from the subjective point of view since there is no a priori distinction between body and world in terms of referential level. Therefore, self is initially identified with what is perceived from the subjective perspective, or selfb and selfc taken as an undifferentiated second row.
To summarize these two points of view, the mind is a part of the body from the objective or material perspective, and the body exists within the mind from a subjective or mental perspective. In terms of references, the objective perspective experiences or locates us where the references are located, and the subjective perspective experiences or locates us where the referents of those references are located.
Other as Negative Entity
In Table 4.22a, other is a negative entity. It exists in the same way as a hole in the ground; both are things that exist for a given subject on an exclusively conceptual level, neither of which has any sensory content. In other words, there is never consciousness of other; it only exists as imputed from higher epistemic levels. However, since there is no experience of other, experience of self is not relatively meaningful. In more poetic terms, the self/other dichotomy exists at the level of the head (in terms of concepts), but it does not exist at the level of the heart (in terms of sensation). As a result, although neither objective nor subjective perspectives are abandoned, subject/object dualism collapses because the axes cease to be dependent (i.e., they are not orthogonal).
It is an open question how the divisions of different epistemic levels should relate to one another. For example, while it may make sense to conceptually discriminate between self and other, to feel inherently differently about them may indicate a confusion with respect to epistemic levels. That is, it may indicate an unjustifiable projection of a difference from one epistemic level onto another (i.e., where that difference does not exist in the way in which it was conceived). In terms of Table 4.22a, this entails identification with selfb but not selfc, even though we are aware of both only at an epistemic distance (and in fact, at the same epistemic distance). Even if this projection is not mistaken, a more detailed understanding of how we exist simultaneously on multiple different epistemic levels is sure to be of benefit.
Self as Intersection vs Union
One method to reconcile the objective and subjective points of view allows them to be independent and does not force them into agreement: the objective perspective dictates where the body is and the subjective perspective dictates what the mental experience is. According to this method, selfb in Table 4.22a constitutes an interaction that does not exist except as a mixture of subjective and objective views, a localized and referential self which is valid from neither point of view on its own, and which exists only because the mereological and referential points of view are (perhaps erroneously) forced to share a common terminological self.
On the other hand, if the self is regarded as a combination of the body from a mereological perspective and the mind from a referential perspective, then there are two main options to define that combination: self as an intersection or self as a union. The view that the self is an intersection results in the identification of the self with selfb in Table 4.22a. Informally, this view may be expressed as a result of taking the mind to be inside the mereological body and the body inside the referential mind. The view of the self as a union results in the identification of the self with selfa…c. Informally, this view may be expressed by saying that the mind exists outside of the mereological body and that the body extends beyond the referential mind.
Increasing Continuity
Although dualism is often criticized for dividing things, it may also be criticized for not dividing enough, or having an insufficient number of differentiations. To address this criticism, the mereological body/world and referential body/mind divisions from Table 4.22a are expanded in Table 4.22b. As before, the columns represent mereological size and the rows represent epistemological level.
[Table 4.22b: A stratified view of the self.]

Table 4.22b illustrates that the notion of self is spread across several epistemic levels and mereological boundaries. In general, the more highly referential the self is (which corresponds to the lower rows of the table), the less localized it becomes. The table may therefore be viewed as a discrete approximation of a continuum from a proximal, dense, and non-referential self to a distal, sparse, and referentially-distant self.
This table also illustrates that the self, which is constituted by the lower-left triangle, is composed of references from multiple epistemic levels. It does not contain any others, which exist as the unknown negative entities forming the upper-right triangle. According to this model, the various selves are distinguishable from one another; for example, the sensory self is not the conceptual self. Further, the location of each aspect of self varies. For example, self1 is located in a body, and self2 is located in a perceptual neighborhood. More generally, the self of each level is located in the same place as its referential content.
Appendix A
Formal Summary
Formal equations corresponding to the basic model, to mereology, and to logic.
This appendix is divided into three main parts:
A summary of the basic model of cognition.
Zeroth-order (mereological) logic.
Higher-order (symbolic and abstract) logic.
These parts may be seen as stages, each of which is developed out of its predecessor; the basic model gives rise to mereology, and mereology gives rise to symbolic logic. This introduction summarizes the motivation behind this formulation by introducing relevant aspects of topology, mereology, and logic.
Overview
One of the main endeavors of this book is to explore the aspects of our experience that are due to the nature of our minds. To do that, it is helpful to determine what can be known independently of the world, or a priori. By identifying and then removing what we contribute to our experience, we can know the world better as it is.
The basic model of cognition relies on the theories of mereology and reference to describe both how our minds are situated in the world and how in turn our minds give rise to mereology and reference. The choice of mereology is motivated by the fact that the existing symbolic formalisms of mathematics and logic run counter to our intuition about the world. In particular, the most common forms of topology and logic work best only when applied to the abstract geometric and symbolic atoms they take as primitive. As Einstein noted:
Geometry […] is not concerned with the relation of the ideas involved in it to objects of experience, but only with the logical connection of these ideas among themselves.
Albert Einstein [Einstein, 1924]
On one hand, it is clear that abstract sciences cannot involve objects directly, since symbols are inherently abstract and cannot be made concrete without losing their linguistic usefulness. However, geometry and logic are used precisely for their ability to describe real-world scenarios, so it is essential that they fit the world as well as possible. The proposal here is to use mereology as a basis for both symbolic logic and topology, in order to take better account of physical objects (which are clearly not composed of zero-dimensional points). To do so, high-dimensional objects are introduced as mereological primitives, which form a subsymbolic foundation. That foundation is augmented with (nominal) references to create symbols that allow the creation of abstract low-dimensional entities, such as geometric primitives. Beginning with high-dimensional objects is motivated by the following observations:
Point-free topologies such as mereotopology are necessary for a subsymbolic model of mind, since point-set topology is inherently atomistic due to its dependence on partless points. In other words, mathematical models of cognition that require everything to have a symbolic description do not leave room for the ineffable, and therefore they are poor models of intuition.
Mereotopology as a mathematical model of mind provides an excellent fit for human cognitive development. Further, it is clear that geometric thought precedes algebraic thought during the course of development.
Purely reductionistic or point-based approaches do not make good psychological models, as cognitive science clearly demonstrates that our minds consist of both bottom-up and top-down processes.
Some people might argue that logic and topology do not need to be cognitively or intuitively correct, since they work in practice. However, if they are used as models of reality, they will inevitably produce an error of the type this book has argued against: treating objects as if they were equivalent to our symbolic representations of them. Therefore, any reduction of this type of mismatch (i.e., by using a non-atomistic model) will be beneficial for both thinking about and interacting with reality.
Mereology
Mereology is a nominalistic version of set theory that was developed by the Polish mathematician Stanislaw Lesniewski. The operation of reference in the current work is roughly equivalent to Lesniewski’s naming operator (ε). Historically, mereology was viewed as a mathematical contender to set theory, a mathematical formalism for collections of things due primarily to the German mathematician Ernst Zermelo. A mathematical set can be implemented in the basic model by combining two operations, reference and whole. In fact, the operation of whole is the primary reason that mereology is an excellent fit for subsymbolic cognition; while set is an intransitive and discrete operation, whole is both transitive and continuous.
In mereotopology, which is the science of mereology applied to topology, it is often assumed that parts have the same dimensionality as their respective wholes. In the version of mereotopology developed here, the universe in which topological analysis begins is continuous and open-dimensional. The combination of these two premises seems to suggest that mereotopology does not allow points and lines; however, they are permitted in a nominal sense, as abstract entities. For example, zero-width points on an interval are nominal boundaries: they do not constitute that interval, but merely divide it. The situation is analogous to using a sharp knife to cut a loaf of bread in half: there is no part of the loaf which is not displaced to one side or the other by the knife. Although the location of the cut may be given a name (e.g., “0.5”), there is no bread associated with it. In mereotopology, therefore, real numbers are understood as nominal boundaries, or a collection of names for the divisions of an interval, rather than entities which constitute that interval.
Points cannot be divided; therefore, point-set topology begins with points, which are the smallest objects it will ever know, and uses sets to construct a universe from those points. This approach is not valid from a psychological perspective because mental space does not have a smallest element out of which other things are built; it is always possible to imagine something smaller. For the same reason, the complementary topological approach that begins with the largest possible object and divides it is also invalid. Therefore, the version of mereotopology presented here starts in the middle, taking arbitrary spatiotemporal volumes as primitive, and uses them to create unions and intersections as necessary.
As opposed to points, the objects that mereotopology takes as primitive are neither infinitely small nor singular. They are concrete by definition and are linguistically better represented by mass nouns than count nouns. As there is no requirement to have partless parts or wholeless wholes, space is assumed to be both continuous and unbounded (i.e., open above and below). Therefore, mereotopology is inherently neither holistic nor reductionistic, and it analyzes objects just as cognition does: by examining them in terms of both their smaller parts and their larger wholes. Using a set of medium-sized objects as a starting point for analysis underscores another facet of psychological compatibility: such a starting point is constructive, in the same way as mental development itself (i.e., medium-sized objects are epistemically prior to both atoms and universes). Therefore, objects are always unknown to some degree, in that their parts and wholes are never fully specified.
As the aim of the version of mereotopology developed here is to be a good fit for cognition, it does not follow a standard mereological development. For a more formally rigorous theory, see GEMTC as developed by Roberto Casati and Achille Varzi [Casati & Varzi, 1999]. For a thorough overview of mereological systems, see the book by Peter Simons [Simons, 2000].
Logic
The reason that a model of cognition requires subsymbolic logic can be demonstrated with the following proposition:
The soccer ball is white.
The problem is that this statement is neither true nor false, since some parts of a soccer ball are white and other parts are not. Symbolic logic, however, requires the subject “soccer ball” to be singular; it is not allowed to have parts (or at least, the subject is necessarily treated as a singular and partless whole by any predicates). In other words, predicates require a symbolic subject in order to yield an all-or-none, true-or-false answer. Therefore, traditional symbolic logic does not work for objects that are continuous. On the other hand, mereological logic allows predicates to be applied to continuous shapes in addition to discrete symbols, so it provides a better fit for actual objects.

[Figure Aa: The five mereological relations.]
In order to illustrate the continuous nature of mereotopology, consider again the five mereological relations presented in section Mereological Space, depicted in Figure Aa. The fifth case is problematic because it cannot be described using classical logic and the parthood relation, unless a new symbol is introduced to name the region of intersection. Intuitively, however, it is clear that A and B overlap; they are parts of each other to some degree, and to a complimentary degree, they are not parts of one another.
[Table Ab: Shape Logic]
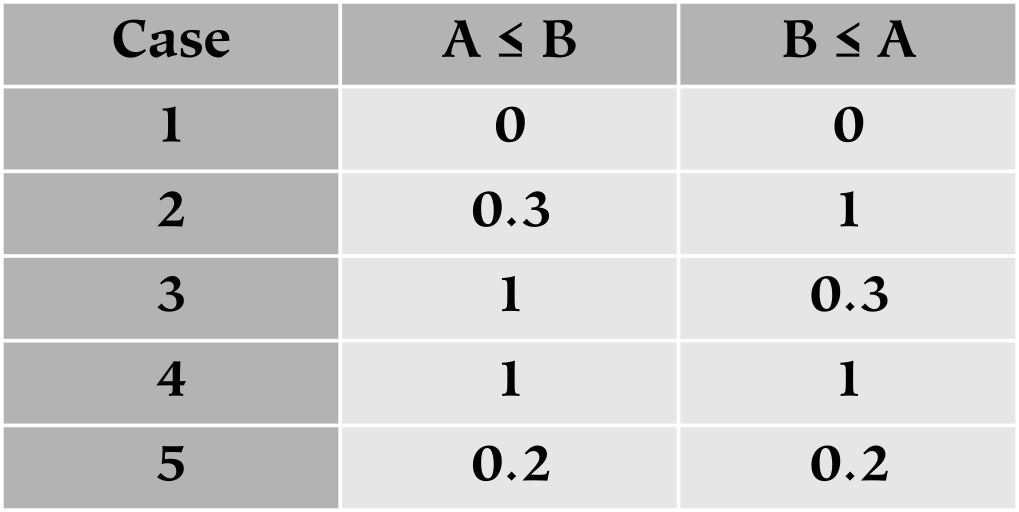
Table Ab is a truth table that illustrates how mereological logic gives a complete description of the situation in Figure Aa, where true is denoted as 1 and false is denoted as 0. The first column of row (5) can be read as “20% of A is a part of B and 80% is not”. This solution is therefore an improvement over symbolic logic because it accounts for the overlap in case (5) without having to explicitly refer to the entity created by the intersection, and therefore it avoids unnecessary symbolic proliferation.
The formula that describes parthood as a continuous function of x and y that yields a value between zero and one is:

A.1 The Basic Model
The basic model of cognition is a foundation
for mereology and symbolic logic. The set of equations that corresponds to the basic model of cognition are developed according to the following guidelines:
A developmental or constructive approach is followed as much as possible. The model is constructive in that it begins with a universe constituted by some number of objects that create sensations. Conceptual wholes are created by taking unions of these sensory features, and parts are created by taking intersections.
Theoretic devices such as axiomatic reduction are not attempted without psychological motivation, since the primary purpose of the model is to describe cognition.
The details of psychological implementation are omitted, since they would interfere with creating a model that is easily understood.
The desired outcome of becoming familiar with the model is a clarification of thought.
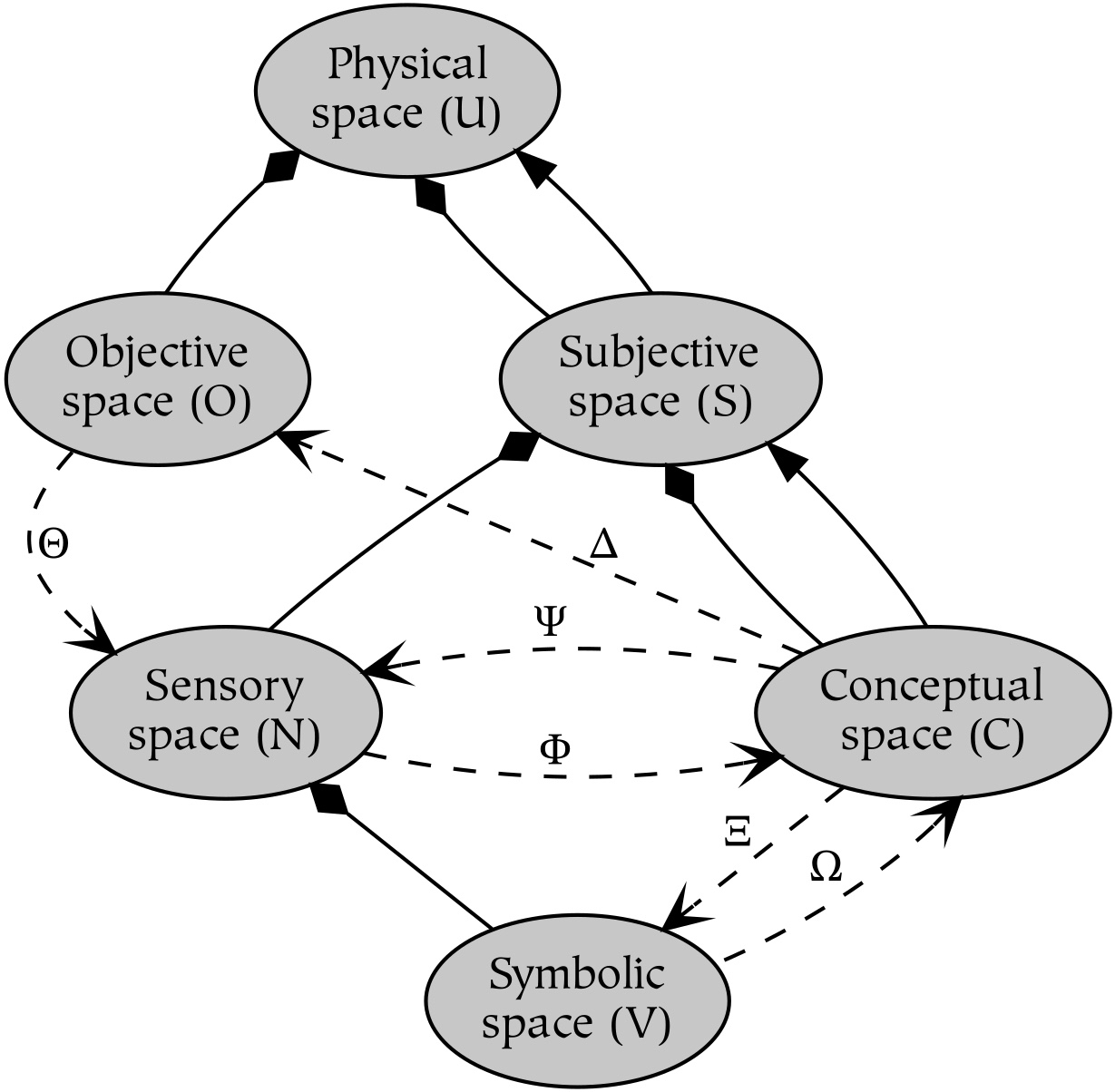
[Figure A.1: The basic model of cognition,
with sensation (Θ) and action (Δ)
mapping to/from subjective subspaces.]
Universes
Physical space (U) is reality, or the physical universe. Its parts are called events.
Subjective space (S) is everything in physical space that is experienced by a given individual. Its parts are called experiences.
Conceptual space (C) is everything within an individual’s subjective space that is conceptualized by that individual. Its parts are called concepts.
Objective space (O) is the space within physical space that is not subjectively experienced (i.e., it is independent of the observer). Its parts are called objects.
Sensory space (N) is the space within a subjective space that is composed of all subjective experience that is not conceptual, such as sensations and emotions. It is composed of sensation or sense data.
Symbolic space (V) is the subspace of sensory space that consists of references to concepts. Its parts are called symbols.
Equation 1.1: Physical Space
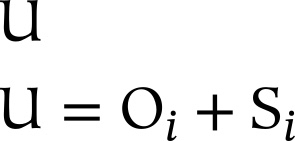
The basic model begins with the physical universe (U).
Physical space is equivalent to the combination of the subjective (Si) and the objective (Oi) spaces of a given individual (i).
Equation 1.2: Subjective Space

Subjective space (S) is defined to be all references to physical space from a given individual’s perspective (i).
Subjective space is equivalent to the union of an individual’s sensory (N) and conceptual (C) spaces. In this and the following equations, the perspective of a particular individual (i) is assumed.
Equation 1.3: Objective Space
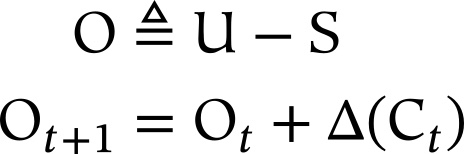
Objective space (O) is defined to be that part of physical space (U) which is not subjective space (S).
Objective space is the result of action (Δ).
Equation 1.4: Sensory Space
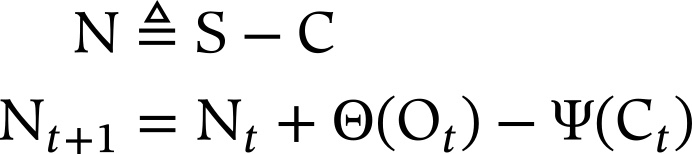
Sensory space (N) is defined to be that part of subjective space (S) which is not conceptual space (C).
Sensory space is the result of bottom-up sensation (Θ) and top-down visualization (Ψ).
Equation 1.5: Conceptual Space
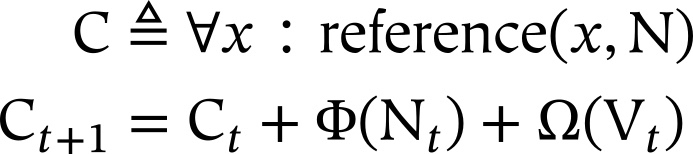
Conceptual space (C) is defined to be all references to sensory space (N).
Conceptual space is the result of conceptualization (Φ) and interpretation (Ω).
Equation 1.6: Symbolic Space
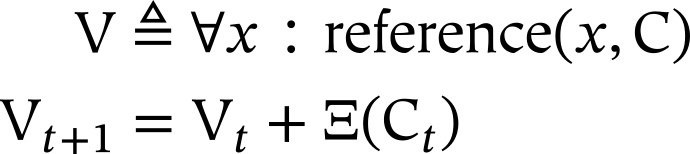
Symbolic space (V) is composed of references to conceptual space (C).
V is the result of symbolization (Ξ).
Relations
Sensation (Θ): Sensation is a causal function. It changes N as a result of O.
Action (Δ): Action is a causal function. It changes O as a result of C.
Conceptualization (Φ): Conceptualization is the whole function. It creates increasingly conceptual wholes as one moves from N to C.
Visualization (Ψ): Visualization is the part function. It creates increasingly sensory parts as one moves from C to N.
Interpretation (Ω): Interpretation is the referent function. It activates referents in V in virtue of symbolic references in N.
Symbolization (Ξ): Symbolization is the reference function. It activates symbolic references in N in virtue of referents in V.
Equation 1.7: Sensation

Sensation (Θ) is a causal function that maps from O to N.
Sensation may be written as the sum of smaller acts of sensation (Θi).
An act of sensing (Θi) produces a sensation (xψ) from an object (x).
Equation 1.8: Action

Action (Δ) is a causal function that maps from C to O.
Action may be written as the sum of smaller acts (Δi).
An act (Δi) produces an object (x) from a concept (xφ).
Equation 1.9: Conceptualization
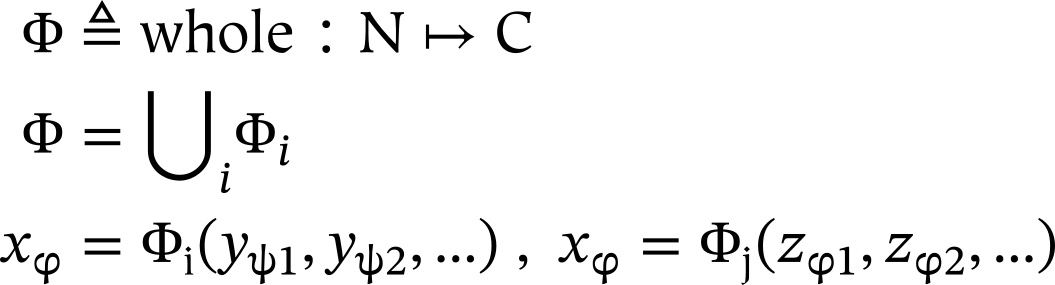
Conceptualization (Φ) is the whole function, that maps from N to C.
Conceptualization may be written as the union of smaller acts of conceptualization (Φi).
An act of conceptualization (Φi, Φj) produces a concept (xφ) from some number of sensations (yψ) or concepts (zφ).
Equation 1.10: Visualization
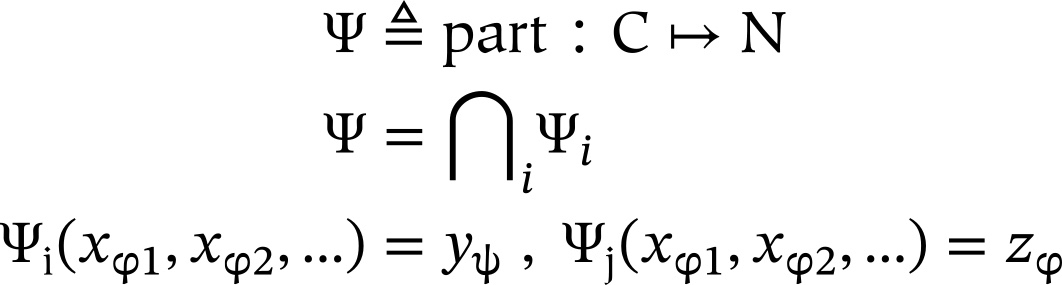
Visualization (Ψ) is the part function, that maps from C to N.
Visualization may be written as the intersection of smaller acts of visualization (Ψi).
An act of visualization (Ψi, Ψj) produces a sensation (yψ) or concept (zφ) from larger conceptual wholes (xφ).
Equation 1.11: Interpretation

Interpretation (Ω) is the referent function, that maps from V to C.
An act of interpretation (Ωi) produces a concept (xφ) from a symbol (xξ).
Equation 1.12: Symbolization

Symbolization (Ξ) is the reference function, that maps from C to V.
An act of symbolization (Ξi) produces a symbol (xξ) from a concept (xφ).
Equation 1.13: Epistemic Level

The epistemic level of a thing is the total number of epistemic operations that must be taken in order to reach ground.
Equation 1.14: Conceptual Order

The conceptual order of a concept or sensation increases according to the number of symbolizations required for its construction. It is analogous to referential level.
A.2 Zeroth-Order Logic
Zeroth-order logic is implemented using
mereology and reference.
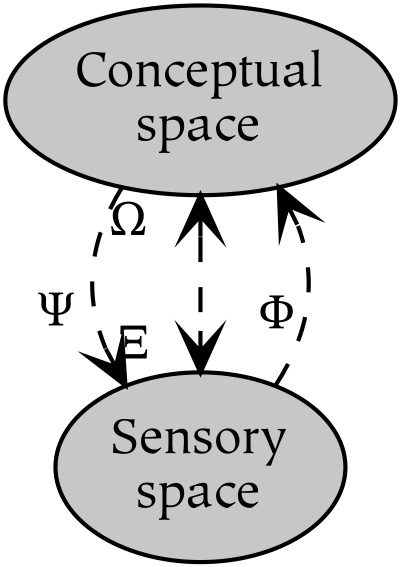
[Figure A.2: a priori cognition.]
The a priori operations of the basic model correspond to part (Ψ), whole (Φ), reference (Ξ), and referent (Ω). All of these operations are used on the right-hand side of the definitions in zeroth-order logic to produce variables on the left. The use of references created with the referential operator (Ξ) as variables on the right-hand side of definitions, however, requires higher-order logic.
The transition from basic model operations to logical values is made by defining zeroth-order truth as being real: a thing is true to the degree that it is a part of the universe. That degree is represented by a continuous truth value between 0 and 1, as described in the section Logic at the beginning of this appendix. In terms of visualization, a continuous version of parthood can be written as:
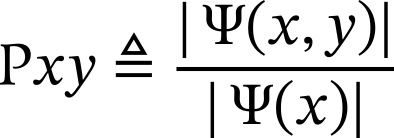
x is a part of y (or Pxy) in proportion to the visualization formed by x and y as a fraction of the visualization of x.
For simplicity, however, the truth values that are used in the following section are binary (i.e., following Boole, they are true and false instead of continuous values; see [Boole, 2003]). Therefore, parthood is written as:
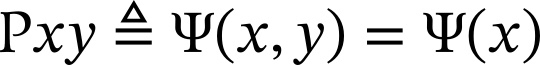
As a final note, all variables (e.g., x and y) in the following equations are assumed to be concepts, so the subscripts indicating conceptuality are omitted (i.e., it is implied that x=xφ and y=yφ unless otherwise indicated).
Mereology
Visualization (Ψ): Given some number of entities, their intersection creates a part.
Conceptualization (Φ): Given some number of entities, their union creates a whole.
Equation 2.1: Part

x is a part of y if the visualization of x and y (which is their intersection) is equal to the visualization of x (i.e., the intersection of x and y removes nothing from x).
Equation 2.2: Whole

x is a whole of y if the conceptualization of x and y is equal to the conceptualization of x (i.e., the union of x and y adds nothing to x).
Similarly, x is a whole of y if and only if y is a part of x.
Equation 2.3: Everything
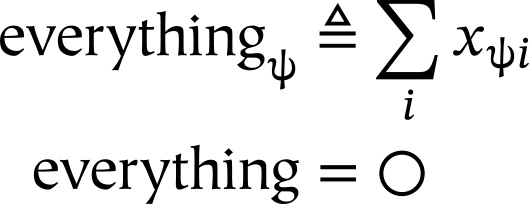
Everythingψ is defined as the combination of all possible sensations.
Everything can be written with the circle symbol (as with other entities, the lack of a subscript indicating epistemic level indicates that the entity is non-referential, or of epistemic level 0).
Equation 2.4: Truth
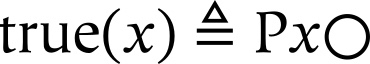
At a mereological level, truth is equated with being real, or a part of everything. As a result, false or unreal things disappear when they are visualized (i.e., they are negative entities). Mereological truth is a continuous form of truth, so x is true to the degree that all parts of x are parts of everything.
Reference
Symbolization (Ξ): Given an entity, a reference to it can be obtained by symbolization.
Interpretation (Ω): Given a reference, its referent can be obtained by interpretation.
Equation 2.5: Reference

x is a reference to y if x is produced by symbolizing y.
Equation 2.6: Referent

y is the referent of x if y is produced by interpreting x.
Basic Operations
Symbolization (Ξ): Given an entity, a reference to it can be created by symbolization.
Interpretation (Ω): Given a reference, its referent can be created by interpretation.
Equation 2.7: Negation
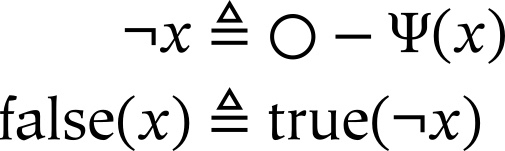
The negation of a variable is its sensory opposite.
The falsity of a variable is the truth of that variable’s negation.
Equation 2.8: Conjunction
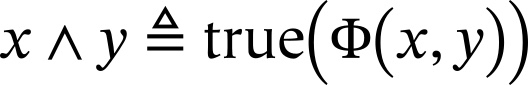
The conjunction of two entities is the truth of the concept that combines both.
Equation 2.9: Disjunction
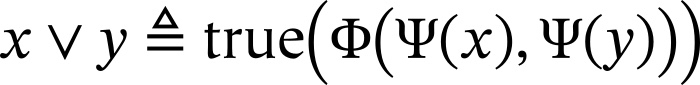
The disjunction of two entities is the truth of the concept that combines the visualization of each.
Equation 2.10: Proper Part
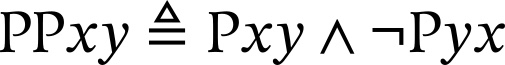
x is a proper part of y if x is a part of y and y is not a part of x.
Equation 2.11: Referential Level
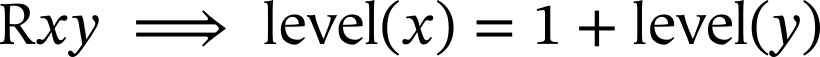
The referential level of a reference is one higher than the referential level of its referent.
Equation 2.12: Dimensionality
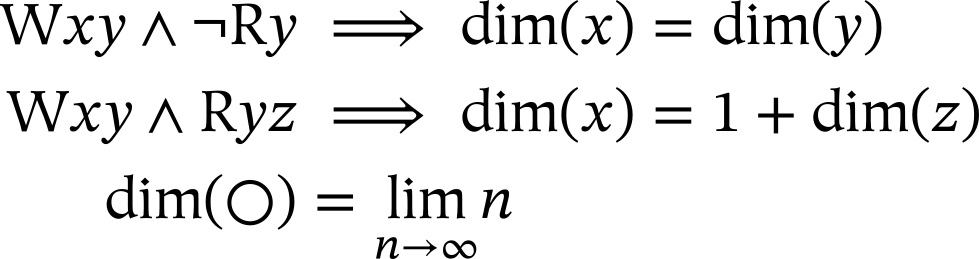
The dimensionality of a part that is not a reference is equal to the dimensionality of its whole.
The dimensionality of a whole of references is one more than the dimensionality of the referent of that reference.
The dimensionality of the universe is limitless.
Equation 2.13: Mereological Context
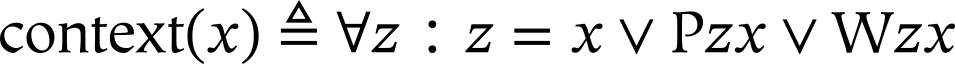
The mereological context of a concept is the concept itself as well as all conceptual parts and wholes of that concept.
A.3 Higher-Order Logic
Higher-order logic is characterized by the use of references. Higher-order logic is presented in two sections: first-order logic and abstract logic.
First-order logic is equivalent to a mereological formulation of modern (first-order) symbolic logic. Therefore, the section on first-order logic uses familiar mereological primitives and existential quantification.
Abstract logic is the basis for creating abstract entities. All abstract entities are defined nominally (i.e., in terms of references). Abstract entities are created by the intersection of higher-order entities; that process causes them to be entities of lower dimensionality, therefore they are incapable of being concrete parts. This reduction of dimensionality is also the basis for introducing boundaries and geometric primitives:
A point is the boundary between two intersecting lines.
A line is the boundary between two intersecting planes.
A plane is the boundary between two intersecting solids.
These geometric entities are not explicitly defined here, since their development follows from the fact that the intersection of two higher-order entities can produce an entity of lower dimensionality.
First-Order Logic
First-order logic allows the use of references via symbolization. Therefore, existential quantifiers (such as ∃ or ∀) are permitted on the right-hand side of definitions.
Equation 3.1: Existence
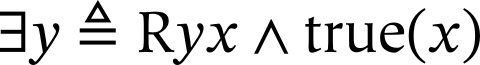
Existence is a property of references that holds if the referent is true (in the sense described in the previous section).
Equation 3.2: Universality
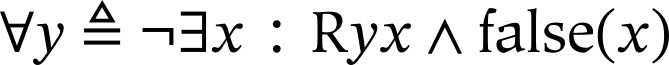
A reference is true for all entities if there is no referent of that reference for which it is false.
Equation 3.3: Atoms
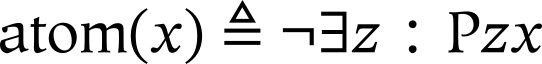
A thing is an atom if it has no parts.
Equation 3.4: Universes
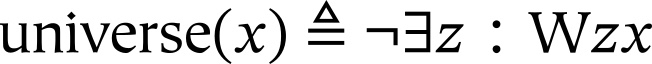
A thing is a universe if it has no wholes.
Equation 3.5: Empty Reference
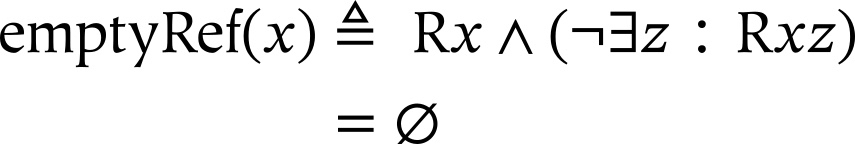
A reference is empty if its referent does not exist.
Empty references are denoted using the null-set operator.
Equation 3.6: Full Reference

A reference is full if it is a reference to a universe.
Equation 3.7: Overlap
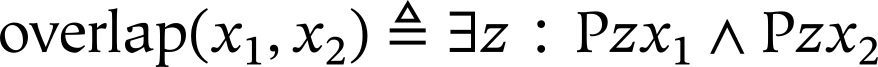
Two entities overlap one another if there is some third entity that is a part of both.
Equation 3.8: Underlap

Two entities underlap one another if there is some third entity that is a whole of both.
Equation 3.9: Complement
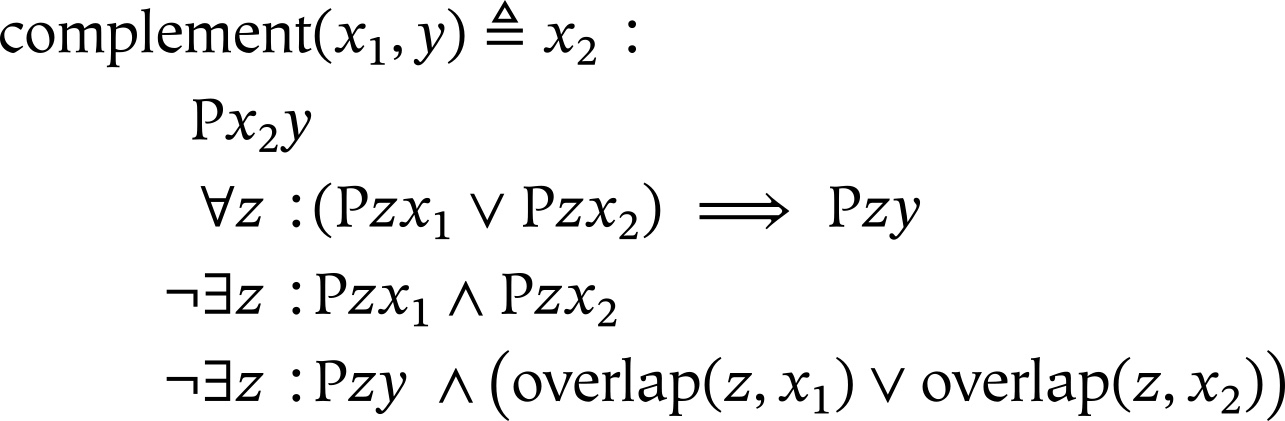
The complement of a part (x1) with respect to a whole (y) is a second part (x2) such that:
- x2 is part of y.
- All parts of x1 or x2 are parts of the whole y.
- x1 and x2 have no parts in common.
- There is no part of that whole which does not overlap with either x1 or x2.
Equation 3.10: Connected
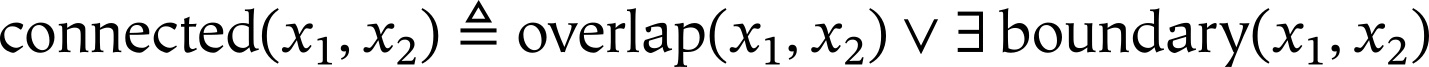
Two entities are connected if they overlap or their boundary exists, where existence of that boundary requires only positive dimensionality.
Equation 3.11: Internal Identity
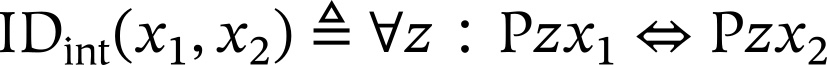
Two things are internally identical if they have the same parts.
Equation 3.12: External Identity

Two things are externally identical if they have the same wholes.
Equation 3.13: Mereological Identity
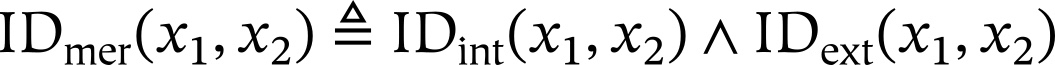
Two things are mereologically identical if they are both internally and externally identical.
Equation 3.14: Referential Identity

Two references are referentially identical if they are equal, or their referents are referentially identical.
Equation 3.15: Nominal Identity

Two things are nominally identical if there is a whole that contains references to both, or there are references to each that are nominally identical.
Abstract Logic
Abstract logic describes abstract entities, which are the low-dimensional result of the intersection of higher-order entities.
Equation 3.16: Boundary
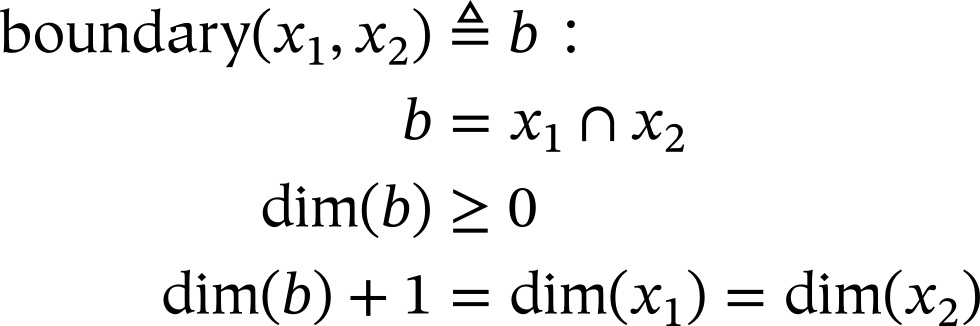
A boundary between two entities (x1, x2) is an entity (b) such that:
- b is the intersection of x1 and x2.
- The dimensionality of b is a non-negative number
- The dimensionality of b is one less than the dimensionality of x1 or x2.
Less formally, a boundary is an intersection of two entities that overlap in some dimensions but not in others. As a result, boundaries are abstract objects, since their dimensionality is less than the dimensionality of the objects that they divide. Hence, the existence of boundaries as abstract objects does not require them to have the dimensionality of the containing space.
Glossary

absolute: A thing is absolute if it does not depend on other things and relative if it does depend on other things. Conceptually, the parts of a thing form its absolute nature, and the wholes of a thing form its relative nature.
abstract: Concepts are abstract if their dimensionality is lower than a concrete concept. The degree of abstraction is therefore related to its conceptual order: zeroth-order concepts are concrete and higher-order concepts are abstract.
atom: An atom is an indivisible thing.
awareness: In the context of this book, awareness is intentional; awareness is always awareness of something else. The term consciousness is used when a more general meaning is intended.
basic categories: Basic categories are “… ‘in the middle’ of a general-to-specific hierarchy. Generalization proceeds ‘upward’ from the basic level and specialization proceeds ‘downward’.” [Lakoff, 2008]. Experimentally, basic categories are basic in the sense that they are known better, as measured by cue validity.
boundary: Boundaries are nominal objects that have an extent of zero along the dimension which they divide. Therefore, boundaries create parts that are connected. For a more substantial discussion, see [Varzi, 2015].
chunking: Chunking is the cognitive process by which multiple concepts in short-term memory are turned into a single concept. It is roughly synonymous with unitization.
complement: The complement of a part is defined as the whole of that part, less that part.
concept: A concept is a part of the conceptual universe. It serves as the basis of thought, it is a whole of sensations, other concepts, or symbols, and can in turn be referenced by a symbol.
conceptual order: Conceptual order indicates the number of symbols that must be traversed in order to reach ontological ground. Zeroth-order concepts consist of sensation and other zeroth-order concepts, while higher-order concepts consist of symbols (or references to constituent concepts). It is also possible for concepts to be composed of other concepts directly (as unitizations), in which case they have a higher epistemic level but not a higher order.
concrete: See abstract.
consciousness: In the context of this book, consciousness is understood as phenomenal consciousness, rather than access consciousness (see [Block, 1995]). Consciousness may be reflexive or intransitive, in which case it is not mediated by references. See also awareness.
contiguous: A thing is (spatially) contiguous if its parts are connected, or discontiguous if its parts are not connected.
deep structure: The deep structure of a sentence, as opposed to its surface structure, is the underlying syntactic structure that determines how its semantic value can be computed from its various syntactic parts (i.e., the words).
dichotomy: A dichotomy is an operation that divides a whole into two parts.
dimension: A dimension is an axis that allows discrimination along its length. It may be nominal, ordinal, interval, or ratio.
direct realism: Direct realism entails that the mind directly engages with external reality. Indirect realism entails that the mind consists of representations of an external reality, and that there is consciousness only of these representations (i.e., and not of external reality itself). Indirect realism is also known as representationalism, or the Representational Theory of Mind (RTM). It is often contrasted with views such as the Computational Theory of Mind (CTM), which posit that mind is a result of mental operations. For a modern treatment, see [Fodor, 2008], [Lycan, 2019], or [Pitt, 2018].
discontiguous: See contiguous.
Dual Process Theory: The theory that the mind can be roughly divided into two systems or processes, one intuitive (System 1) and one rational (System 2). In the Dual Process context, intuitive thinking is called Type 1 and symbolic thinking is called Type 2.
epistemic level: The epistemic level (or referential level) of an entity is its distance from some ontological ground, which has an epistemic level of zero. See also conceptual order and section Recursion.
epistemological priority: An entity is epistemologically prior to another if it has a lower epistemic level.
epistemology: Epistemology is the study of knowledge. Ontology is the study of being or existence.
equivalence class: An equivalence class is a set of things whose members are equal or equivalent. Equivalence classes are created by wholes of references. See nominal identity.
existence: Existence is a property exclusively of references. If a reference exists, then it can be validly dereferenced (i.e., its reference has a location).
extension: See intension.
external identity: See internal identity.
feature space: A feature space is a space that consist of features. For example, visual space is a 3‑D space augmented with the feature of color.
finitism: Mathematically, finitism entails that infinity is a process, while infinitism entails that infinity is a completed process or a number (as required for infinitesimal points or infinite sets). For more details, see the essays section of the companion web site, [http://theWholePart.com/essays].
Flatland: Flatland is a two-dimensional world that was originally described by Edwin Abbott [Abbott & Stewart, 2008]. Three-dimensional people do enter that land, but they have unexplainable and magical properties from the point of view of the people of Flatland.
generalization: Generalization refers to the way that a higher-order concept becomes abstract. For example, a conceptual whole can generalize across its parts by isolating their common features. See also unitization.
hierarchy: A hierarchy is a tree-like structure that consists of one or more dimensions. Two prominent types of taxonomies are meronomies, which are hierarchies composed of concrete parts and wholes, and taxonomies, which are hierarchies composed of abstract parts and wholes.
holism: Holism explains a system in terms of its wholes. Reductionism explains a system in terms of its parts. Both are biased views in that they presuppose that their own explanation is more valid or fundamental than other alternatives.
idea: The idea corresponding to a given concept is the activation of that concept along with its mereological context, or all of its parts and wholes.
indirect realism: See direct realism.
infinity: Infinity is often understood as a number that is bigger than any other number, although it is better understood as a limit process with no upper bound (at least according to finitism).
intension: The intension of a set is a characteristic property of all members of that set. The extension of a set is the enumeration of the elements of that set.
intentionality: Intentionality is a philosophical term that indicates a sense of aboutness. For example, awareness is intentional because it is always awareness of something else (and therefore inherently dualistic).
internal identity: Internal identity establishes the identity of an object in terms of its parts. External identity establishes the identity of an object in terms of its wholes.
isomorphism: Isomorphism, which literally means “the same shape”, is a relation between two things that expresses equivalence or congruence of shape. In referential space, a reference is isomorphic to its referent if they are internally and externally identical within their respective domains.
mereology: Mereology is the study of parts and wholes. As a mathematical science, it was originally developed by the Polish philosopher Stanislaw Lesniewski.
metaconcept: A metaconcept is a particular kind of higher-order concept whose purpose is to equate a word and its object by composing both of their symbols. It is a key component of the proposed mechanism for language: the symbol of the word activates the metaconcept, which causes the subsequent interpretation and visualization of the symbol for the object.
negative entity: A negative entity is a concept that cannot possibly be sensed such as a hole in the ground, that is known only in virtue of the lack of sensation of dirt. A positive entity is a concept that can only be sensed, and which is known only bottom-up.
nominal identity: Nominal identity entails that two entities are identical in virtue of being designated by the same name or type. For example, me from eight years ago is the same me that woke up today because I have the same name now that I did then.
nominalism: Nominalism is a philosophical doctrine that claims that objects in the world are only nominally identical from moment to moment, and not intrinsically identical (see nominal identity). Nominalism is opposed to philosophical realism, which typically maintains that reality is composed of natural kinds. Believers in natural kinds hold that objects have an essence in virtue of which they remain the same from moment to moment. For example, I am the same person now that I was eight years ago because of certain enduring and essential properties.
object: An object is a physical thing, as opposed to sensations and concepts which are different forms of mental things.
object permanence: Object permanence entails knowing that unobserved objects continue to exist in an external world. This requires that a concept is developed for an object that exists independently of the sensation of that object. Object permanence as a developmental stage was first studied by the psychologist Jean Piaget.
ontology: See epistemology.
open: If a space is open, or open from above and open from below, then there are no ultimate mereological limits such as largest universes or smallest atoms. Dimensionality may also be open, such that an open-dimensional space has an unlimited number of dimensions.
orthogonal: Two dimensions are orthogonal if a change in one dimension does not entail a change in the other. In two dimensions, for example, orthogonal lines form a right angle to one another.
overlap: Two objects overlap if they share a common part. Two objects underlap if there is some larger whole that contains both.
panpsychism: Panpsychism is the belief that everything is conscious, or that mind and matter are everywhere coextensive. See [Goff & Seager, 2017].
part: A part is a thing that is contained in another thing, which is called its whole. If the part is strictly smaller than that whole, it is called a proper part. Similarly, a thing is a whole if it has parts.
partition: A partition of a thing is a complete and exact decomposition of that thing into some number of parts. Every location within the whole is contained in some part, and no location is contained in more than one part.
particular: A particular object is a concrete, individual object, such as a specific horse, which may be seen as a collection of universals. A universal is a property such as horseness that may apply to multiple particular horses.
percept: A percept is a combination of a concept and its associated sense data. A percept is a part of perceptual space that exists in a continuum between sensory and conceptual spaces.
positive entity: See negative entity.
prototype theory: Prototype theory is proposal within cognitive psychology that pertains to the understanding of cognitive categories, and which is often contrasted with exemplar theory.
realism: See nominalism.
reductionism: See holism.
reference: A reference is a representation of a thing, as opposed to the thing itself. Linguistically, references may be either lightweight signs or heavyweight symbols. A reference names or refers to a referent.
referent: See reference.
reflection: Reflection is a continuous version of (discrete) reference.
relative: See absolute.
self-reference: See self-reference.
sensation: A sensation is a part of sensory space. It constitutes nonconceptual mental content and is a subjective referent to either an object or a concept (in the latter case, it is called a symbol).
set of all sets: The set of all sets (or the Universal Set) is that set which is composed of all sets. If not created using constructivist principles, it creates paradoxes such as the Russell-Zermelo Paradox (see [Irvine & Deutsch, 2016]).
space: Space is used in this book as a metaphor for both reality and mind. It operates as a subsymbolic replacement for discrete entities.
subsymbolic: In the context of cognitive science, theories such as connectionism offer a subsymbolic alternative to traditional symbolic approaches. In mathematics, a similar distinction exists between point-free topologies such as mereology and point-set topology. See also symbol.
symbol: A symbol is a sensation that references a concept. The term “symbol” as used in this book entails a cognitive symbol, as opposed to verbal or written symbols that exist independently of mind. See also subsymbolic.
thing: A thing is a generic term that is further differentiated into objects, sensations, concepts, and symbols; for further details, see section Epistemic Universes.
token: A token is an instance of a type. For example, there are two tokens of the word “a” in the previous sentence, but those tokens consist of a single type. A type is also called a class of things.
type: See token.
underlap: See overlap.
unitization: Unitization refers to the process of making wholes as unions of parts. It does not entail generalization.
universal: See particular.
universe: A universe is a space that is an ultimate whole from some particular point of reference.
whole: See part.
Bibliography

Abbott & Stewart, 2008: Abbott, E. A., & Stewart, I. (2008). The annotated flatland: A romance of many dimensions. New York, NY: Basic Books.
Armstrong, 2010: Armstrong, D. M. (2010). Universals: An opinionated introduction. Boulder, CO: Westview Press.
Austin, 2005: Austin, J. H. (2005). Zen and the brain: Toward an understanding of meditation and consciousness. Cambridge, MA: MIT Press.
Baddeley, 2001: Baddeley, A. (2001). The Concept of Episodic Memory. Philosophical Transactions: Biological Sciences, 356(1413), 1345–1350.
Baylor & Lamb, 1979: Baylor, D. A., Lamb, T., & Yau, K.-W. (1979). Responses of retinal rods to single photons. The Journal of Physiology, 288(1), 613–634.
Bermudez & Cahen, 2015: Bermúdez, J., & Cahen, A. (2015). Nonconceptual mental content. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Fall 2015). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/fall2015/entries/content-nonconceptual/]
Bernays, 1991: Bernays, P. (1991). Axiomatic set theory. New York, NY: Dover Publications.
Block, 1983: Block, N. (Ed.). (1983). Readings in philosophy of psychology. Vol. 1. Cambridge, MA: Harvard University Press.
Block, 1995: Block, N. (1995). On a confusion about a function of consciousness. Behavioral and Brain Sciences, 18(2), 227–247.
Bohm, 2002: Bohm, D. (2002). Wholeness and the implicate order. New York, NY: Routledge.
Boole, 2003: Boole, G. (2003). The laws of thought. Amherst, NY: Prometheus Books.
Braitenberg, 2004: Braitenberg, V. (2004). Vehicles: Experiments in synthetic psychology. Cambridge, MA: MIT Press.
Capra, 1992: Capra, F. (1992). The Tao of physics: An exploration of the parallels between modern physics and Eastern mysticism. London, United Kingdom: Flamingo.
Casati, 2009: Casati, R. (2009). Surfaces, holes, shadows. In R. L. Poidevin (Ed.), The Routledge Companion to Metaphysics, 382–388. Routledge.
Casati & Varzi, 1999: Casati, R., & Varzi, A. C. (1999). Parts and places: The structures of spatial representation. Cambridge, MA: MIT Press.
Chomsky, 1980: Chomsky, N. (1980). Rules and representations. New York, NY: Columbia University Press.
Chomsky, 1995: Chomsky, N. (1995). Aspects of the theory of syntax. Cambridge, MA: MIT Press.
Churchland, 1988: Churchland, P. M. (1988). Matter and consciousness: A contemporary introduction to the philosophy of mind. Cambridge, MA: MIT Press.
Dennett, 1991: Dennett, D. C. (1991). Consciousness explained. Boston, MA: Little, Brown and Co.
Dogen et al., 2013: Dōgen, Tanahashi, K., & Levitt, P. (2013). The essential Dogen: Writings of the great zen master. Boston, MA: Shambhala.
Domjan & Burkhard, 1993: Domjan, M., & Burkhard, B. (1993). The principles of learning and behavior. Pacific Grove, CA: Brooks/Cole Publication Co.
Einstein, 1924: Einstein, A. (1924). Relativity: The special and the general theory (R. W. Lawson, Trans.). London, United Kingdom: Methuen & Co Ltd.
Einstein & Sullivan, 1972: Einstein, A., & Sullivan. (1972, March 29). The Einstein papers: a man of many parts. New York Times, p1 [https://www.nytimes.com/1972/03/29/archives/the-einstein-papers-a-man-of-many-parts-the-einstein-papers-man-of.html]
Evans & Stanovich, 2013: Evans, J. St. B. T., & Stanovich, K. E. (2013). Dual-process theories of higher cognition: Advancing the Debate. Perspectives on Psychological Science, 8(3), 223–241. [https://doi.org/10.1177/1745691612460685]
Fodor, 2008: Fodor, J. A. (2008). LOT 2: The language of thought revisited. Oxford, United Kingdom: Oxford University Press.
Gallistel, 1993: Gallistel, C. R. (1993). The Organization of learning. Cambridge, MA: MIT Press.
Ganeri, 2012: Ganeri, J. (2012). The self: Naturalism, consciousness, and the first-person stance. Oxford, United Kingdom: Oxford University Press.
Gardenfors, 2004: Gärdenfors, P. (2004). Conceptual spaces: The geometry of thought. Cambridge, MA: MIT Press.
Gersho & Grey, 2003: Gersho, A., & Gray, R. M. (2003). Vector quantization and signal compression. Boston, MA: Kluwer.
Gladwell, 2007: Gladwell, M. (2007). Blink: The power of thinking without thinking. New York, NY: Back Bay Books.
Goff & Seager, 2017: Goff, W., Philip, Seager, & Allen-Hermanson, S. (2017). Panpsychism. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Winter 2017). [https://plato.stanford.edu/archives/win2017/entries/panpsychism/]
Grossberg, 1988: Grossberg, S. (Ed.). (1988). Neural networks and natural intelligence. Cambridge, MA: MIT Press.
Gyamtso, 1988: Gyamtso, T. & Hookham, S. K. (Ed.). (1988). Progressive Stages of Meditation on Emptiness. Oxford, United Kingdom: Longchen Foundation.
Hanh, 1998: Thich Nhat Hanh (1998). Mindful Consumption. Dharma talk, July 17, 1998. Plum Village, France.
Hansen, 2017-1: Hansen, C. (2017). Zhuangzi. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Spring 2017). [https://plato.stanford.edu/archives/spr2017/entries/zhuangzi/]
Hein, 2010: Hein, J. L. (2010). Discrete structures, logic, and computability. Boston, MA: Jones and Bartlett.
Hofstadter & Dennett, 1988: Hofstadter, D. R., & Dennett, D. C. (Eds.). (1988). The mind’s I: Fantasies and reflections on self and soul. Toronto, Canada: Bantam Books.
Huang et al., 2006: Huang, A. L., Chen, X., Hoon, M. A., Chandrashekar, J., Guo, W., Tränkner, D., Ryba, N. J. P., & Zuker, C. S. (2006). The cells and logic for mammalian sour taste detection. Nature, 442(7105), 934–938. [https://doi.org/10.1038/nature05084]
Hyde & Raffman, 2018: Hyde, D., & Raffman, D. (2018). Sorites paradox. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Summer 2018). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/sum2018/entries/sorites-paradox/]
Ingram & Tallant, 2018: Ingram, D., & Tallant, J. (2018). Presentism. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Spring 2018). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/spr2018/entries/presentism/]
Irvine & Deutsch, 2016: Irvine, A. D., & Deutsch, H. (2016). Russell’s Paradox. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Winter 2016). [https://plato.stanford.edu/archives/win2016/entries/russell-paradox/]
Jackendoff, 1994: Jackendoff, R. (1994). Patterns in the mind: Language and human nature. New York, NY: BasicBooks.
Jackendoff, 2007: Jackendoff, R. (2007). Language, consciousness, culture: Essays on mental structure. Cambridge, MA: MIT Press.
Jackendoff, 2009: Jackendoff, R. (2009). Foundations of language: Brain, meaning, grammar, evolution. Oxford, United Kingdom: Oxford University Press.
Jennings, 2015: Jennings, C. (2015). The standard theory of conscious perception. Proceedings of the 37th Annual Meeting of the Cognitive Science Society.
Kahneman, 2013: Kahneman, D. (2013). Thinking, fast and slow. New York, NY: Farrar, Straus and Giroux.
Kant, 1781: Kant, I., & Meiklejohn, J. M. D. (1990). Critique of pure reason. Buffalo, NY: Prometheus Books.
Kellner, 2011: Kellner, B. (2011). Self-awareness (svasamvedana) and Infinite Regresses: A Comparison of Arguments by Dignāga and Dharmakīrti. Journal of Indian Philosophy, 39(4/5), 411–426.
Koerth-Baker, 2010: Koerth-Baker, M. (2010, November). Kids (and animals) who fail classic mirror tests may still have sense of self. Scientific American.
Lakoff & Núñez, 2011: Lakoff, G., & Núñez, R. E. (2011). Where mathematics comes from: How the embodied mind brings mathematics into being. New York, NY: Basic Books.
Lakoff, 2008: Lakoff, G. (2008). Women, fire, and dangerous things: What categories reveal about the mind. Chicago, IL: The University of Chicago Press.
Langer, 1967: Langer, S. K. K. (1967). An introduction to symbolic logic. New York, NY: Dover Publications.
Levine, 2000: Levine, D. S. (2000). Introduction to neural and cognitive modeling. Mahwah, NJ: Lawrence Erlbaum Associates Publishers.
Lewis, 1991: Lewis, D. K. (1991). Parts of classes. Oxford, United Kingdom: Blackwell.
Libet, 1985: Libet, B. (1985). Unconscious cerebral initiative and the role of conscious will in voluntary action. Behavioral and Brain Sciences, 8(4), 529-539.
Linnebo & Shapiro, 2019: Linnebo, Ø., & Shapiro, S. (2019). Actual and Potential Infinity. Noûs, 53(1), 160–191. [https://doi.org/10.1111/nous.12208]
Longchenpa & Barron, 2007: Longchenpa, D. & Barron, R. (2007). The precious treasury of philosophical systems: A treatise elucidating the meaning of the entire range of spiritual approaches. Junction City, CA: Padma Publications.
Lycan, 1998: Lycan, W. G. (Ed.). (1998). Mind and cognition: A reader. Oxford, United Kingdom: Blackwell.
Lycan, 2019: Lycan, W. (2019). Representational theories of consciousness. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Fall 2019). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/fall2019/entries/consciousness-representational/]
Lyons, 1981: Lyons, J. (1981). Language and linguistics: An introduction. Cambridge, United Kingdom: Cambridge University Press.
Markosian, 2016: Markosian, N. (2016). Time. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Fall 2016). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/fall2016/entries/time/]
Maturana & Varela, 1992: Maturana, H. R., & Varela, F. J. (1992). The tree of knowledge: The biological roots of human understanding. Boston, MA: Shambhala.
Minsky & Lee, 1988: Minsky, M., & Lee, J. (1988). The society of mind. New York, NY: Simon & Schuster.
Minsky, 2006: Minsky, M. (2006). The emotion machine: Commonsense thinking, artificial intelligence, and the future of the human mind. New York, NY: Simon & Schuster.
Moltmann, 2003: Moltmann, F. (2003). Parts and wholes in semantics. Oxford, United Kingdom: Oxford University Press.
Nagel, 1989: Nagel, T. (1989). The view from nowhere. New York, NY: Oxford University Press.
Percy, 2000: Percy, W. (2000). The message in the bottle: How queer man is, how queer language is, and what one has to do with the other. New York, NY: Picador.
Pinker, 1997: Pinker, S. (1997). How the mind works. New York, NY: Norton.
Pinker, 2000: Pinker, S. (2000). Words and rules: The ingredients of language. New York, NY: Perennial.
Pinker, 2007: Pinker, S. (2007). The stuff of thought: Language as a window into human nature. New York, NY: Viking.
Pitt, 2018: Pitt, D. (2018). Mental representation. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Winter 2018). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/win2018/entries/mental-representation/]
Potter, 2004: Potter, M. D. (2004). Set theory and its philosophy: A critical introduction. Oxford , United Kingdom: Oxford University Press.
Quine, 1969: Quine, W. V. (1969). Ontological relativity and other essays. New York, NY: Columbia University Press.
Quine, 1980: Quine, W. V. (1980). From a logical point of view: 9 logico-philosophical essays. Cambridge, MA: Harvard University Press.
Quine, 2001: Quine, W. V. (2001). Word and object. Cambridge, MA: MIT Press.
Reicher, 2019: Reicher, M. (2019). Nonexistent objects. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Winter 2019). Retrieved from [https://plato.stanford.edu/archives/win2019/entries/nonexistent-objects/]
Rogers, 1995: Alec Rogers. (2005, June 1). Temporal and spatial variability in animal cognition. Retrieved from [http://theWholePart/essays/thesis]
Rucker, 1983: Rucker, R. v. B. (1983). Infinity and the mind: The science and philosophy of the infinite. Canada: Bantam Books.
Russel, 1972: Russell, B. (1972). A history of western philosophy. New York, NY: Simon and Schuster.
Sacks, 1985: Sacks, O. (1985). The man who mistook his wife for a hat and other clinical tales. New York, NY: Summit Books.
Scarantino & de Sousa, 2018: Scarantino, A., & de Sousa, R. (2018). Emotion. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Winter 2018). [https://plato.stanford.edu/archives/win2018/entries/emotion/]
Schwartz & Reisberg, 1991: Schwartz, B., & Reisberg, D. (1991). Learning and memory. New York, NY: Norton.
Shantideva & Wallace, 1997: Shantideva, Wallace, V. A., & Wallace, B. A. (1997). A guide to the Bodhisattva way of life: Bodhicharyavatara. Ithaca, NY: Snow Lion Publications.
Simons, 2000: Simons, P. M. (2000). Parts: A study in ontology. New York, NY: Oxford University Press.
Sorensen, 2019: Sorensen, R. (2019). Nothingness. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Summer 2019). [https://plato.stanford.edu/archives/sum2019/entries/nothingness/]
Stjernfelt, 2007: Stjernfelt, F. (2007). Diagrammatology: An investigation on the borderlines of phenomenology, ontology, and semiotics. Dordrecht, Netherlands: Springer.
Thakchoe, 2011: Thakchoe, S. (2017). The theory of two truths in India. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Spring 2017). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/spr2017/entries/twotruths-india/]
Thanissaro, 2013: Thanissaro Bhikkhu. (2013). Satipatthana sutta: frames of reference (Thanissaro Bhikkhu, Trans.). Access to Insight (BCBS Edition), Majjhima Nikaya 10. [http://www.accesstoinsight.org/tipitaka/mn/mn.010.than.html]
Tiles, 2004: Tiles, M. (2004). The philosophy of set theory: An historical introduction to Cantor’s paradise. Mineola, NY: Dover Publications.
Varela, Thompson & Rosch, 2000: Varela, F. J., Thompson, E., & Rosch, E. (2000). The embodied mind: Cognitive science and human experience. Cambridge, MA: MIT Press.
Varzi, 2015: Varzi, A. (2015). Boundary. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Winter 2015). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/win2015/entries/boundary/]
Wasserman, 2018: Wasserman, R. (2018). Material constitution. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy (Fall 2018). Metaphysics Research Lab, Stanford University. [https://plato.stanford.edu/archives/fall2018/entries/material-constitution/]
Wilson et al., 1989: Wilson, T. D., Dunn, D. S., Kraft, D., & Lisle, D. J. (1989). Introspection, attitude change, and attitude-behavior consistency: The disruptive effects of explaining why we feel the way we do. In Advances in experimental social psychology 22, 287–343. Elsevier.
Zahavi, 2008: Zahavi, D. (2008). Subjectivity and selfhood: Investigating the first-person perspective. Cambridge, MA: MIT Press.


![]()
![]()